Analysis of the Finite Point Method for Fractional Cable Equations
-
摘要:
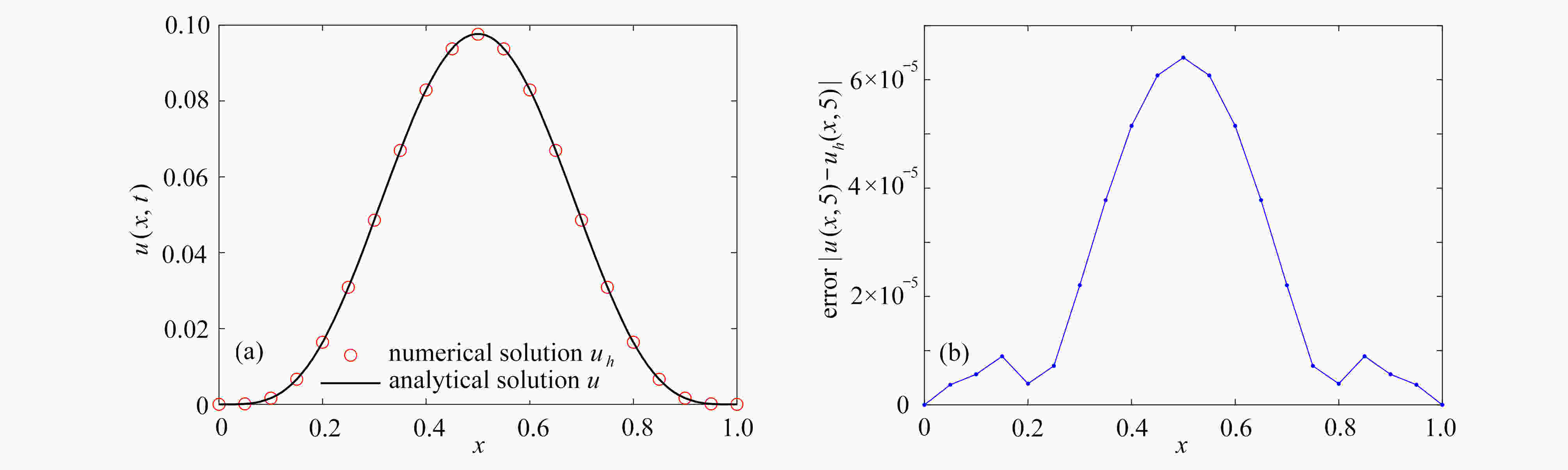
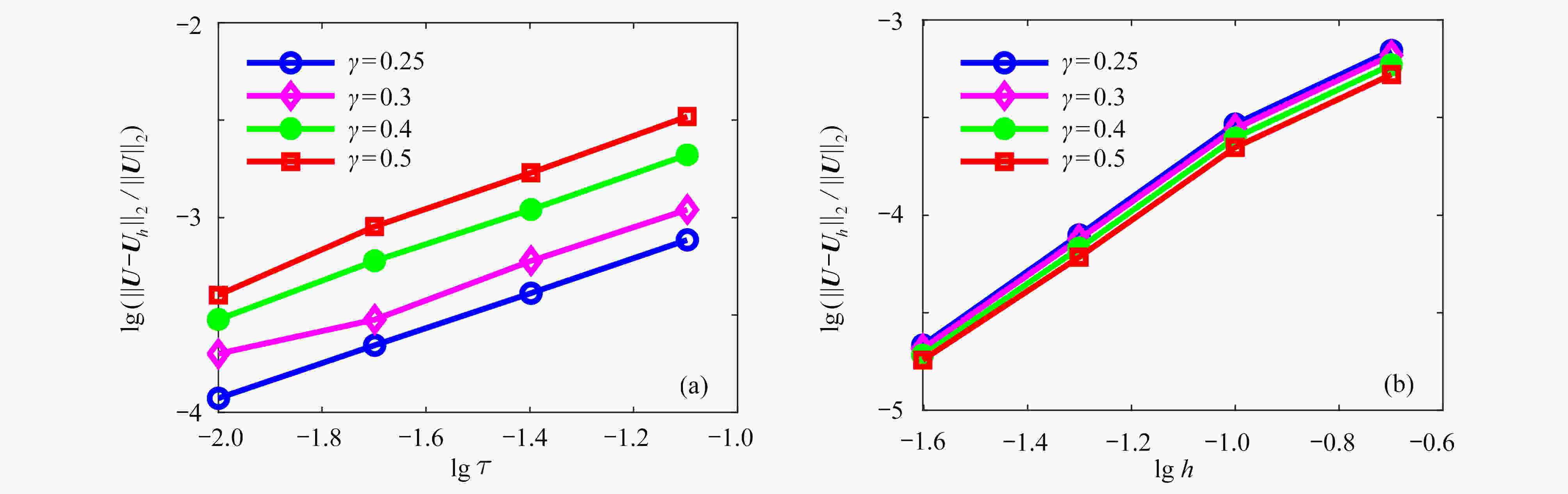
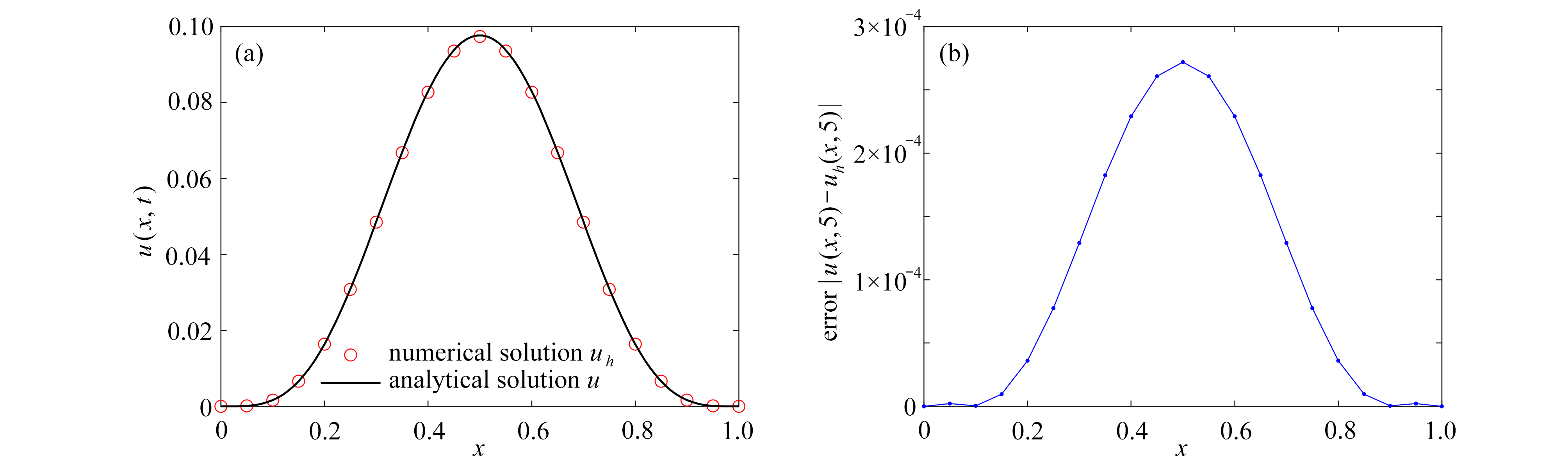
通过采用中心差分格式离散Riemann-Liouville时间分数阶导数和用有限点法建立离散代数系统,提出了数值求解分数阶Cable方程的无网格有限点法,详细推导了该方法的理论误差估计。数值算例证实了该方法的有效性和收敛性,并验证了理论分析结果。
-
关键词:
- 分数阶Cable方程 /
- 有限点法 /
- 无网格法 /
- 误差估计
Abstract:With the central difference scheme to discretize the Riemann-Liouville time fractional derivatives and by means of the finite point method to establish discrete algebraic equation systems, a meshless finite point method was proposed for the numerical analysis of the fractional Cable equation. The error estimation of the method was derived and discussed in detail. Numerical examples verify the efficiency and convergence of the method and confirm the theoretical results.
-
Key words:
- fractional Cable equation /
- finite point method /
- meshless method /
- error estimation
-
表 1 有限点法和径向基函数法在
$h = 0.1 ,\;T=1$ 时的$ {L^\infty } $ 误差Table 1. The
$ {L^\infty } $ -errors of the finite point method and the radial basis function method gained with$h = 0.1,\; T=1$ $ \tau $ $ \gamma = 0.25 $ $ \gamma = 0.3 $ the finite point method the radial basis function method[8] the finite point method the radial basis function method[8] 1/10 1.670 9E−5 4.515 2E−4 2.256 6E−5 3.831 0E−4 1/20 1.110 9E−5 2.542 5E−4 1.409 1E−5 1.926 8E−4 1/40 7.983 2E−6 1.557 3E−4 9.429 7E−6 9.759 9E−4 1/80 6.275 8E−6 1.065 3E−4 6.903 4E−6 5.011 6E−5 -
[1] HU X L, ZHANG L M. Implicit compact difference schemes for the fractional Cable equation[J]. Applied Mathematical Modelling, 2012, 36(9): 4027-4043. doi: 10.1016/j.apm.2011.11.027 [2] LIAO H L, SUN Z Z. Maximum norm error estimates of efficient difference schemes for second-order wave equations[J]. Journal of Computational and Applied Mathematics, 2010, 235(8): 2217-2233. [3] KHAN M A, ALI N H M, HAMID N N A. The design of new high-order group iterative method in the solution of two-dimensional fractional Cable equation[J]. Alexandria Engineering Journal, 2021, 60(4): 3553-3563. doi: 10.1016/j.aej.2021.01.008 [4] QUINTANA-MURILLO J, YUSTE S B. An explicit numerical method for the fractional Cable equation[J]. International Journal of Differential Equations, 2011, 72(2): 447-466. [5] ZHUANG P, LIU F, ANH V, et al. Stability and convergence of an implicit numerical method for the non-linear fractional reaction-subdiffusion process[J]. IMA Journal of Applied Mathematics, 2005, 74: 645-667. [6] AL-MASKARI M, KARAA S. The lumped mass FEM for a time-fractional Cable equation[J]. Applied Numerical Mathematics, 2018, 132: 73-90. doi: 10.1016/j.apnum.2018.05.012 [7] ZHENG R, LIU F, JIANG X, et al. Finite difference/spectral methods for the two-dimensional distributed-order time-fractional Cable equation[J]. Computers & Mathematics With Applications, 2020, 80(6): 1523-1537. [8] DEHGHAN M, ABBASZADEH M, MOHEBBI A. Error estimate for the numerical solution of fractional reaction-subdiffusion process based on a meshless method[J]. Journal of Computational and Applied Mathematics, 2015, 280: 14-36. doi: 10.1016/j.cam.2014.11.020 [9] DEHGHAN M, ABBASZADEH M. Analysis of the element free Galerkin (EFG) method for solving fractional Cable equation with Dirichlet boundary condition[J]. Applied Numerical Mathematics, 2016, 109: 208-234. doi: 10.1016/j.apnum.2016.07.002 [10] CHENG Y M. Meshless Methods[M]. Beijing: Science Press, 2015. [11] 王红, 李小林. 二维瞬态热传导问题的无单元Galerkin法分析[J]. 应用数学和力学, 2021, 42(5): 460-469. (WANG Hong, LI Xiaolin. Analysis of 2D transient heat conduction problems with the element-free Galerkin method[J]. Applied Mathematics and Mechanics, 2021, 42(5): 460-469.(in Chinese)WANG Hong, LI Xiaolin. Analysis of 2D transient heat conduction problems with the element-free Galerkin method[J]. Applied Mathematics and Mechanics, 2021, 42(5): 460-469. (in Chinese)) [12] 李煜冬, 王发杰, 陈文. 瞬态热传导的奇异边界法及其MATLAB实现[J]. 应用数学和力学, 2019, 40(3): 259-268. (LI Yudong, WANG Fajie, CHEN Wen. MATLAB implementation of a singular boundary method for transient heat conduction[J]. Applied Mathematics and Mechanics, 2019, 40(3): 259-268.(in Chinese)LI Yudong, WANG Fajie, CHEN Wen. MATLAB implementation of a singular boundary method for transient heat conduction[J]. Applied Mathematics and Mechanics, 2019, 40(3): 259-268. (in Chinese)) [13] OÑATE E, IDELSOHN S, ZIENKIEWICZ O C, et al. A finite point method in computational mechanics: applications to convective transport and fluid flow[J]. International Journal for Numerical Methods in Engineering, 1996, 39(22): 3839-3866. doi: 10.1002/(SICI)1097-0207(19961130)39:22<3839::AID-NME27>3.0.CO;2-R [14] ORTEGA E, FLORES R, OÑATE E, et al. A-posteriori error estimation for the finite point method with applications to compressible flow[J]. Computational Mechanics, 2017, 60: 219-233. doi: 10.1007/s00466-017-1402-7 [15] OÑATE E, PERAZZO F, MIQUEL J. A finite point method for elasticity problems[J]. Computers & Structures, 2001, 79(22/25): 2151-2163. [16] LI X L, DONG H Y. Error analysis of the meshless finite point method[J]. Applied Mathematics and Computation, 2020, 382: 125326. doi: 10.1016/j.amc.2020.125326 [17] CHEN C M, LIU F, TURNER I, et al. A Fourier method for the fractional diffusion equation describing sub-diffusion[J]. Journal of Computational Physics, 2007, 227(2): 886-897. doi: 10.1016/j.jcp.2007.05.012 [18] LI X L. Error estimates for the moving least-square approximation and the element-free Galerkin method in n-dimensional spaces[J]. Applied Numerical Mathematics, 2016, 99: 77-97. doi: 10.1016/j.apnum.2015.07.006 [19] BRENNER S C, SCOTT L R. The Mathematical Theory of Finite Element Methods[M]. New York: Springer, 1994. -















 下载:
下载:



































 渝公网安备50010802005915号
渝公网安备50010802005915号