Boundary Value Problems of a Kirchhoff Type Plate Model Based on the Simplified Strain Gradient Elasticity and the Application
-
摘要:
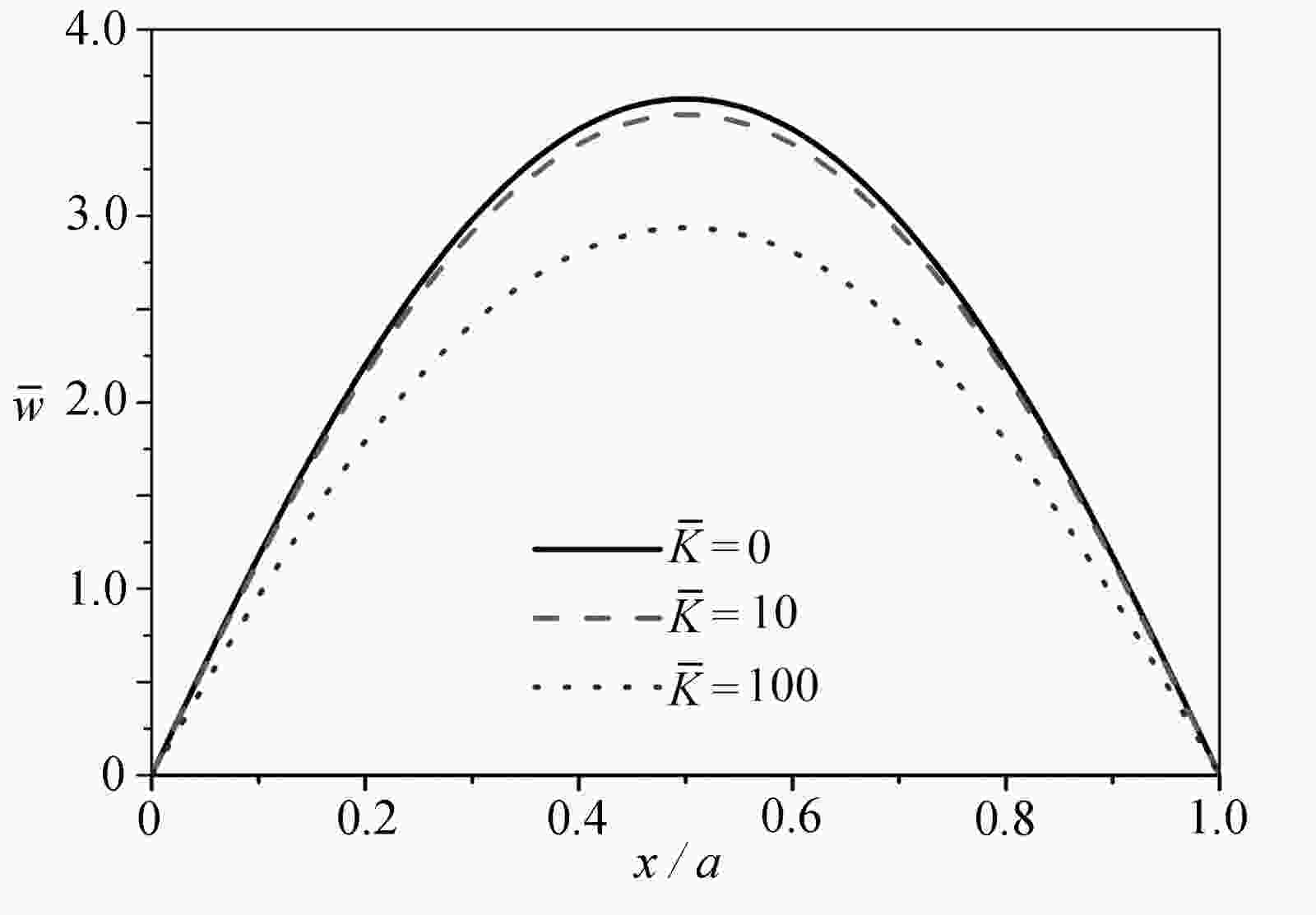
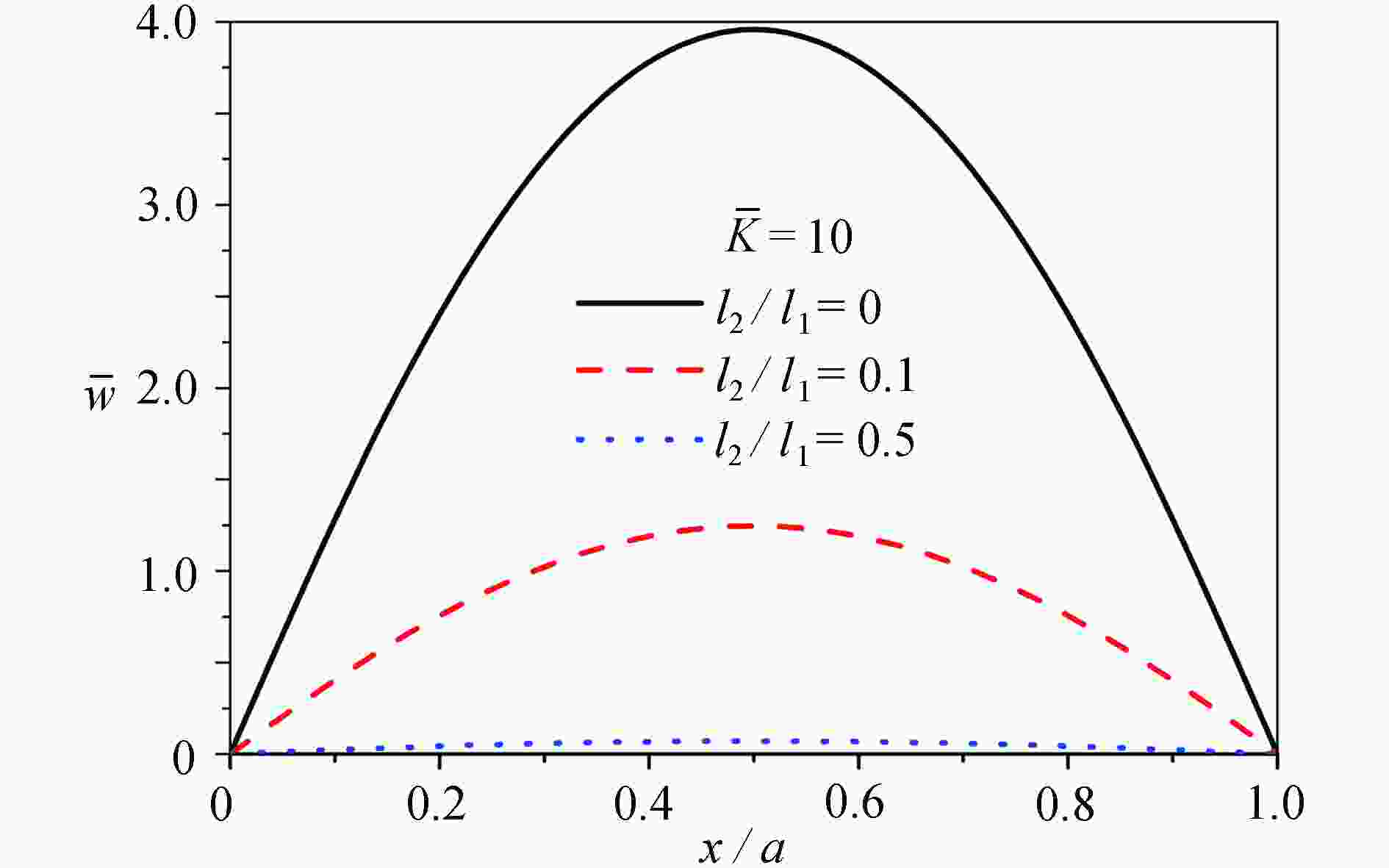
考虑应变梯度和速度梯度的影响，建立薄板控制微分方程及给出其边值问题的提法，修正了前人给出的薄板角点条件。采用Levy法，给出受分布力作用下简支板的挠度及自由振动频率的解析解。通过与文献中分子动力学数据对比，验证了该文模型的有效性并提出校核材料参数的一种方法。研究结果表明，增大弹性地基和应变梯度参数可以有效提高板的等效刚度，而速度梯度参数则相反。该文提出的板的边值问题为研究薄板在复杂支撑边界及外荷载等条件提供了理论依据。同时，有望为其有限元法、有限差分法和基于能量原理的Galerkin法等数值方法提供理论依据。
Abstract:A new type of thin plate model and the related nonclassical boundary value problems were established within the framework of strain gradient and velocity gradient elasticity. The closed-form solutions of deflections and free vibrational frequencies of a simply supported plate resting on an elastic foundation were obtained. The results of the present model agree well with those predicted by the molecular dynamics. Numerical results show that, the elastic foundation and the strain gradient parameter have a stiffness-hardening effect, while the velocity gradient parameter has a stiffness-softening effect. The proposed boundary value problems are of great significance to the study of the mechanical behaviors of plates under complex boundary conditions and external loadings. Furthermore, it will be useful for developing effective numerical methods such as the finite element method, the finite difference method and the Garlerkin method.
-
Key words:
- plate /
- vibration /
- deflection /
- strain gradient theory /
- variational principle
-
图 2 弹性地基上的周边简支矩形板
Figure 2. A fully simply supported rectangular plate resting on an elastic foundation
图 3 周边简支方形板的基频与其边长的关系
Figure 3. The fundamental frequency vs. the side length of a simply supported square plate
图 4 地基刚度系数对周边简支方板位移形状的影响
Figure 4. Effects of the foundation stiffness on the displacement of a simply supported square plate for y=a/2
图 5 地基刚度系数对周边简支方板基频的影响
Figure 5. Effects of the foundation stiffness on the fundamental frequency of a simply supported square plate
图 6 应变梯度参数l2对周边简支方板位移形状的影响
Figure 6. Effects of strain gradient parameter l2 on the displacement of a simply supported square plate for y=a/2
图 7 应变梯度参数l2对周边简支方板基频的影响
Figure 7. Effects of strain gradient parameter l2 on the fundamental frequency of a simply supported square plate
图 8 速度梯度参数l1对周边简支方板位移形状的影响
Figure 8. Effects of velocity gradient parameter l1 on the displacement of a simply supported square plate for y=a/2
图 9 速度应变梯度参数l1对周边简支方板基频的影响
Figure 9. Effects of velocity gradient parameter l1 on the fundamental frequency of a simply supported square plate
A1 坐标系(x, y)和(n, s)及板边界Γ
A1. Coordinate systems (x, y) and (n, s) at a piecewise smooth plate boundary Γ
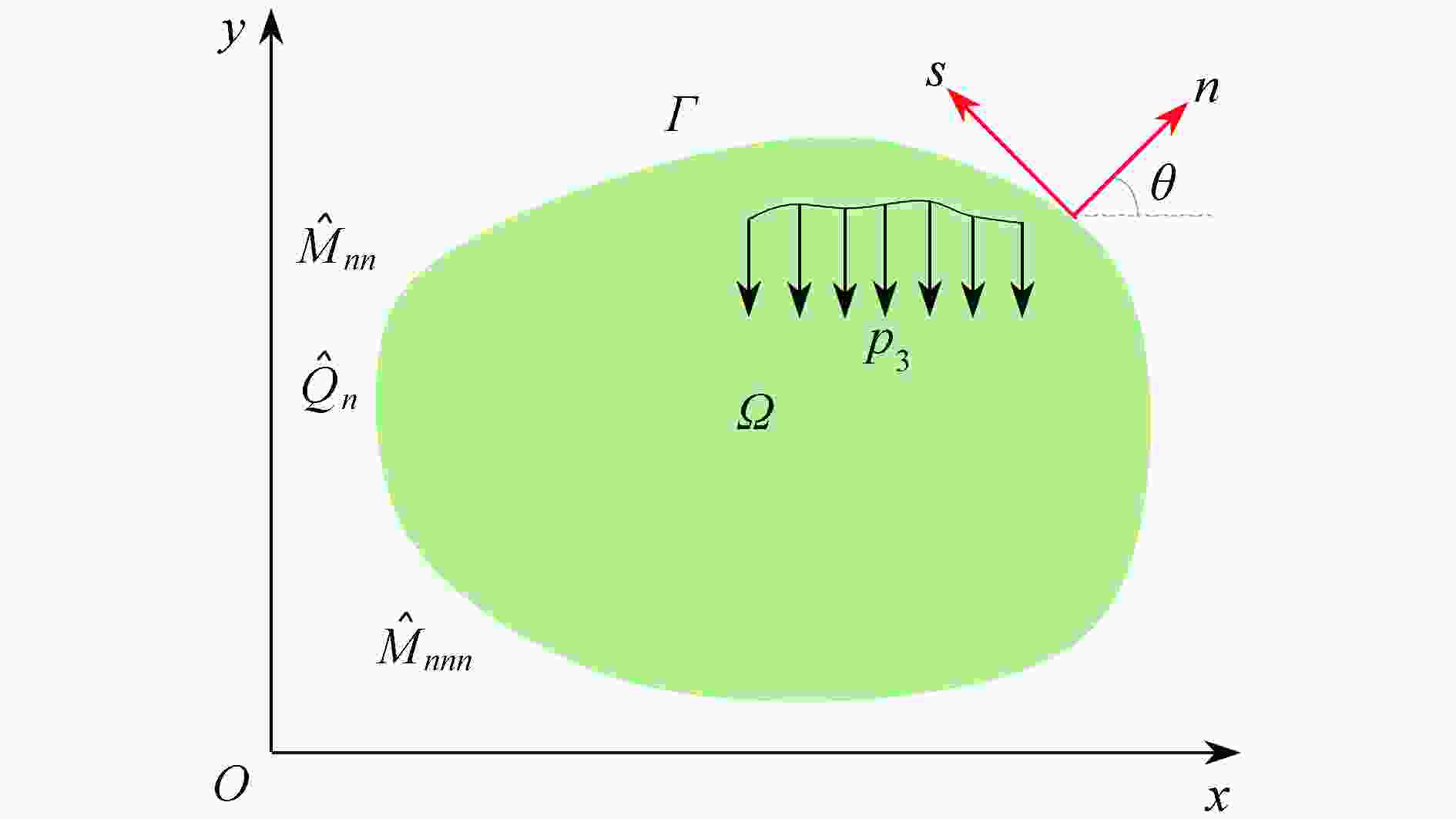
表 1 矩形薄板3种常见的边界条件
Table 1. Three common boundary conditions (BCs) for a rectangular plate
boundary condition BC1 BC2 BC3 clamped w=0 w,x=0 Mxxx=0 or w,xx=0 simply support w=0 M*xx=0 Mxxx=0 or w,xx=0 free $ Q_x^* + \rho hl_1^2{\ddot w_{,x}} = 0 $ M*xx=0 Mxxx=0 or w,xx=0 
下载: 导出CSV
-
[1] 周剑秋, 黄连军, 王英. 基于应变梯度位错理论的纳晶-无定形态层状复合材料的力学性能研究[J]. 工程力学, 2014, 31(1): 224-228. (ZHOU Jianqiu, HUANG Lianjun, WANG Ying. Mechanical behaviors of crystalline-amorphous nanolaminates based on strain gradient dislocation theory[J]. Engineering Mechanics, 2014, 31(1): 224-228.(in Chinese) doi: 10.6052/j.issn.1000-4750.2012.07.0497 [2] 王学滨. 考虑应变梯度及刚度劣化的剪切带局部变形分析[J]. 工程力学, 2006, 23(10): 101-106. (WANG Xuebin. Analysis of localized deformation in shear band considering degraded stiffness based on gradient-dependent plasticity[J]. Engineering Mechanics, 2006, 23(10): 101-106.(in Chinese) doi: 10.3969/j.issn.1000-4750.2006.10.020 [3] LAM D C C, YANG F, CHONG A C M, et al. Experiments and theory in strain gradient elasticity[J]. Journal of the Mechanics and Physics of Solids, 2003, 51(8): 1477-1508. doi: 10.1016/S0022-5096(03)00053-X [4] CHEN C Q, SHI Y, ZHANG Y S, et al. Size dependence of Young’s modulus in ZnO nanowires[J]. Physical Review Letters, 2006, 96(7): 075505. doi: 10.1103/PhysRevLett.96.075505 [5] EOM K, PARK H S, YOON D S, et al. Nanomechanical resonators and their applications in biological/chemical detection: nanomechanics principles[J]. Physics Reports, 2011, 503(4/5): 115-163. [6] 秦江, 黄克智, 黄永刚. 采用特征线方法对混合硬化情况下基于变形机制的应变梯度工程塑性理论的研究[J]. 工程力学, 2009, 26(9): 176-185. (QIN Jiang, HWANG Kehchih, HUANG Yonggang. A study on the conventional theory of mechanism-based strain gradient plasticity for mixed hardening by the method of characteristics[J]. Engineering Mechanics, 2009, 26(9): 176-185.(in Chinese) [7] ASKES H, AIFANTIS E C. Gradient elasticity in statics and dynamics: an overview of formulations, length scale identification procedures, finite element implementations and new results[J]. International Journal of Solids and Structures, 2011, 48(13): 1962-1990. doi: 10.1016/j.ijsolstr.2011.03.006 [8] WANG L, HU H. Flexural wave propagation in single-walled carbon nanotubes[J]. Physical Review B, 2005, 71(19): 195412. doi: 10.1103/PhysRevB.71.195412 [9] WANG L, HU H, GUO W. Validation of the non-local elastic shell model for studying longitudinal waves in single-walled carbon nanotubes[J]. Nanotechnology, 2006, 17(5): 1408-1415. doi: 10.1088/0957-4484/17/5/041 [10] ANSARI R, SAHMANI S, ARASH B. Nonlocal plate model for free vibrations of single-layered graphene sheets[J]. Physics Letters A, 2010, 375(1): 53-62. doi: 10.1016/j.physleta.2010.10.028 [11] KHAKALO S, NIIRANEN J. Anisotropic strain gradient thermoelasticity for cellular structures: plate models, homogenization and isogeometric analysis[J]. Journal of the Mechanics and Physics of Solids, 2020, 134: 103728. doi: 10.1016/j.jmps.2019.103728 [12] 王碧蓉, 邓子辰, 徐晓建, 等. 剪力非局部因子对双壁碳纳米管中弯曲波频散特性的影响[J]. 应用数学和力学, 2014, 35(5): 478-486. (WANG Birong, DENG Zichen, XU Xiaojian, et al. Effects of nonlocal shear factor on flexural wave dispersion in double-walled carbon nanotubes[J]. Applied Mathematics and Mechanics, 2014, 35(5): 478-486.(in Chinese) doi: 10.3879/j.issn.1000-0887.2014.05.002 [13] 郑晓静, 周又和. 集中载荷作用下弹性地基圆薄板大挠度问题的精确解[J]. 力学学报, 1988, 20(2): 161-172. (ZHENG Xiaojing, ZHOU Youhe. On the exact solution to large deflection problem of circular plate on elastic foundation under a concentrated load[J]. Acta Mechanica Sinica, 1988, 20(2): 161-172.(in Chinese) [14] 陈玲玲, 杨旭, 刘洋, 等. 全应变梯度挠曲电纳米梁有限单元法研究[J]. 计算力学学报, 2020, 37(5): 545-552. (CHEN Lingling, YANG Xu, LIU Yang, et al. Research on finite element method of nanobeam considering flexoelectricity based on general strain gradient elasticity theory[J]. Chinese Journal of Computional Mechanics, 2020, 37(5): 545-552.(in Chinese) doi: 10.7511/jslx20191104002 [15] MINDLIN R D. Micro-structure in linear elasticity[J]. Archive for Rational Mechanics and Analysis, 1964, 16(1): 51-78. doi: 10.1007/BF00248490 [16] ASKES H, SUIKER A S J, SLUYS L J. A classification of higher-order strain-gradient models-linear analysis[J]. Archive of Applied Mechanics, 2002, 72(2): 171-188. [17] POLIZZOTTO C. A second strain gradient elasticity theory with second velocity gradient inertia, part Ⅰ: constitutive equations and quasi-static behavior[J]. International Journal of Solids and Structures, 2013, 50(24): 3749-3765. doi: 10.1016/j.ijsolstr.2013.06.024 [18] PAPARGYRI-BESKOU S, GIANNAKOPOULOS A E, BESKOS D E. Variational analysis of gradient elastic flexural plates under static loading[J]. International Journal of Solids and Structures, 2010, 47(20): 2755-2766. [19] VENTSEL E, KROUTHAMMER T. Thin Plate and Shells[M]. New York: Marcel Dekker, 2001. [20] XU X J, DENG Z C. Effects of strain and higher order inertia gradients on wave propagation in single-walled carbon nanotubes[J]. Physica E: Low-Dimensional Systems and Nanostructures, 2015, 72: 101-110. doi: 10.1016/j.physe.2015.04.011 -
计量
- 文章访问数: 786
- HTML全文浏览量: 311
- PDF下载量: 126
- 被引次数: 0
渝公网安备50010802005915号