Analysis and Simulation of Natural Frequencies of Slightly Curved Pipes
-
摘要:
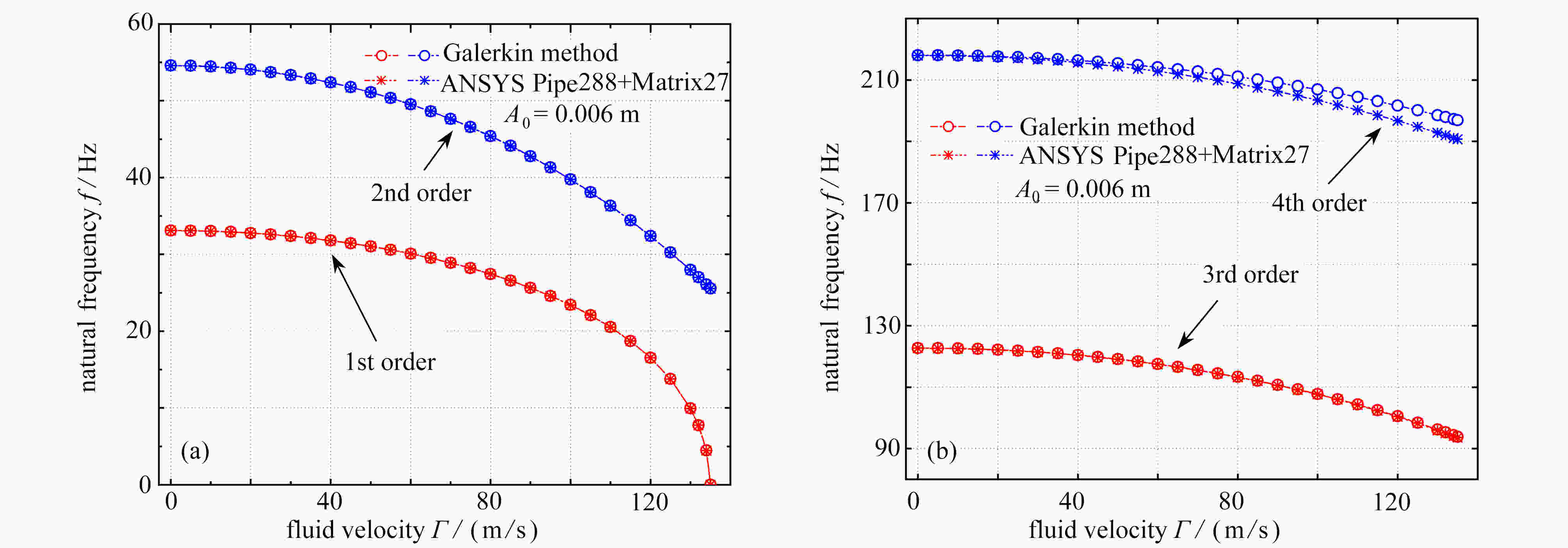
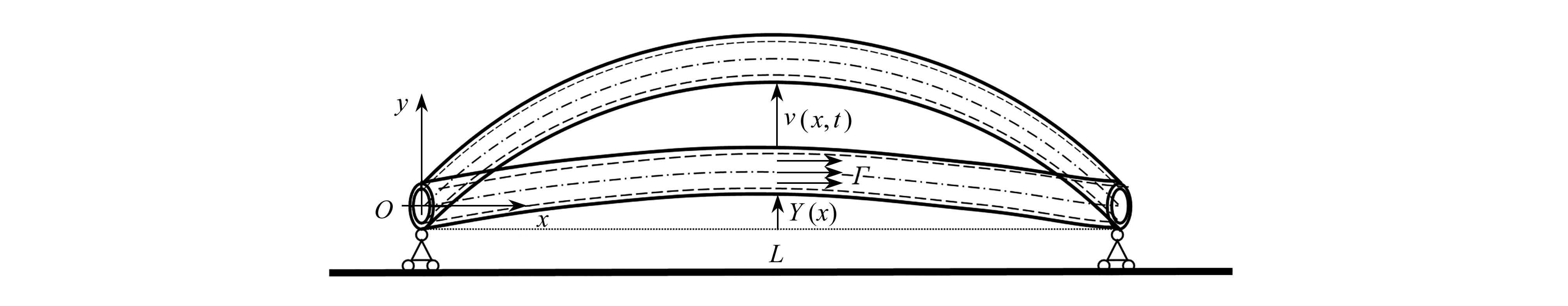
首次建立了基于Timoshenko梁理论的微曲输流管道横向振动的动力学模型,并分析了流体流动影响下微曲管道横向自由振动的固有特征。采用广义Hamilton原理,导出了考虑流体影响的微曲管道横向振动的控制方程,通过Galerkin截断对控制方程离散化,再由广义本征值问题得到管道横向振动的固有频率,并研究了液体流速和弯曲幅度对管道横向固有振动特征的影响。发展了基于等效刚度和等效阻尼方法的考虑流体影响的微曲管道振动分析的有限元仿真计算方法,并通过有限元软件实现数值仿真,验证了Galerkin截断的分析结果以及所建立的Timoshenko微曲管道动力学模型的有效性。研究表明,流体的流速以及管道的弯曲幅度对管道横向振动固有频率均有显著影响。
-
关键词:
- 管道 /
- Timoshenko /
- 固有频率 /
- Galerkin截断 /
- 数值仿真
Abstract:For the transverse vibration of slightly curved pipes, a dynamic mechanical model based on the Timoshenko beam theory was established for the 1st time. The natural vibration characteristics of slightly curved pipes under the influence of the fluid flow were analyzed. With the generalized Hamiltonian principle, the governing equation of the transverse vibration of slightly curved pipes under the fluid-structure coupling effect was derived. Based on the Galerkin truncation, the natural frequencies of slightly curved pipes were obtained with the generalized eigenvalue method. Effects of the fluid velocity and the initial deflection on the natural vibration characteristics of the pipe were studied. The equivalent stiffness and damping method-based finite element simulation of the natural vibration of the slightly curved pipe was developed. Then through the finite element numerical simulation, the results of the Galerkin truncation method and the effectiveness of the Timoshenko model were verified. The work shows that, both the fluid velocity and the initial deflection have significant effects on the natural frequencies of slightly curved pipes.
-
Key words:
- pipe /
- Timoshenko /
- natural frequency /
- Galerkin truncation /
- numerical simulation
-
表 1 1Cr18Ni9输流管道与流体参数
Table 1. Physical parameters of the 1Cr18Ni9 pipe and fluid
parameter value pipe span L/m 1 pipe density $ {\rho _{\text{p}}} $/(kg/m3) 7 930 outer diameter D/m 0.006 pipe thickness h/m 0.000 6 Young’s modulus E/GPa 194.0 moment of inertia Ib/m4 3.756 × 10−11 Poisson’s ratio μ 0.3 fluid density $ {\rho _{\text{f}}} $/(kg/m3) 872.0 -
[1] 武新丽, 罗维东, 吴冬, 等. 压力管道破坏的形式、原因与防止[J]. 中国科技信息, 2005(19): 29, 46. (WU Xinli, LUO Weidong, WU Dong, et al. The form, cause and prevention of pressure pipe damage[J]. China Science and Technology Information, 2005(19): 29, 46.(in Chinese)WU Xinli, LUO Weidong, WU Dong, et al. The form, cause and prevention of pressure pipe damage[J]. China Science and Technology Information, 2005(19): 29, 46. (in Chinese) [2] 郑哲敏. 管道的振动[J]. 清华大学学报, 1956(1): 32-36, 286. (ZHENG Zheming. Analysis of pipe vibrations[J]. Journal of Tsinghua University, 1956(1): 32-36, 286.(in Chinese)ZHENG Zheming. Analysis of pipe vibrations[J]. Journal of Tsinghua University, 1956(1): 32-36, 286. (in Chinese)) [3] 朱竑祯, 王纬波, 殷学文, 等. 基于分层模型的功能梯度输流管道耦合振动[J]. 振动与冲击, 2019, 38(20): 203-209. (ZHU Hongzhen, WANG Weibo, YIN Xuewen, et al. Coupled vibration of functionally graded pipes conveying fluid based on a multi-layered model[J]. Journal of Vibration and Shock, 2019, 38(20): 203-209.(in Chinese)ZHU Hongzhen, WANG Weibo, YIN Xuewen, et al. Coupled vibration of functionally graded pipes conveying fluid based on a multi-layered model[J]. Journal of Vibration and Shock, 2019, 38(20): 203-209. (in Chinese)) [4] YAMASHITA K, YAGYU K, YABUNO H. Nonlinear interactions between unstable oscillatory modes in a cantilevered pipe conveying fluid[J]. Nonlinear Dynamics, 2019, 98(4): 2927-2938. doi: 10.1007/s11071-019-05236-7 [5] MAO X Y, DING H, CHEN L Q. Steady-state response of a fluid-conveying pipe with 3:1 internal resonance in supercritical regime[J]. Nonlinear Dynamics, 2016, 86(2): 795-809. doi: 10.1007/s11071-016-2924-9 [6] TAN X, DING H, CHEN L Q. Nonlinear frequencies and forced responses of pipes conveying fluid via a coupled Timoshenko model[J]. Journal of Sound and Vibration, 2019, 455: 241-255. doi: 10.1016/j.jsv.2019.05.019 [7] 肖斌, 周玉龙, 高超, 等. 考虑流体附加质量的输流管道振动特性分析[J]. 振动与冲击, 2021, 40(15): 182-188. (XIAO Bin, ZHOU Yulong, GAO Chao, et al. Analysis of vibration characteristics of pipeline with fluid added mass[J]. Journal of Vibration and Shock, 2021, 40(15): 182-188.(in Chinese)XIAO Bin, ZHOU Yulong, GAO Chao, et al. Analysis of vibration characteristics of pipeline with fluid added mass[J]. Journal of Vibration and Shock, 2021, 40(15): 182-188. (in Chinese)) [8] 张挺, 林震寰, 林通, 等. 内激励型振荡衰减流作用下输流管道动力不稳定分析[J]. 振动与冲击, 2021, 40(3): 284-290. (ZHANG Ting, LIN Zhenhuan, LIN Tong, et al. Dynamic instability analysis of pipeline conveying fluid under action of internally excited oscillation attenuation flow[J]. Journal of Vibration and Shock, 2021, 40(3): 284-290.(in Chinese)ZHANG Ting, LIN Zhenhuan, LIN Tong, et al. Dynamic instability analysis of pipeline conveying fluid under action of internally excited oscillation attenuation flow[J]. Journal of Vibration and Shock, 2021, 40(3): 284-290. (in Chinese)) [9] GAO P X, YU T, ZHANG Y L, et al. Vibration analysis and control technologies of hydraulic pipeline system in aircraft: a review[J]. Chinese Journal of Aeronautics, 2021, 34(4): 83-114. doi: 10.1016/j.cja.2020.07.007 [10] LI Q, LIU W, LU K, et al. Flow-induced buckling statics and dynamics of imperfect pipes[J]. Archive of Applied Mechanics, 2021, 91: 4553-4569. doi: 10.1007/s00419-021-02023-y [11] ZHOU K, NI Q, CHEN W, et al. Static equilibrium configuration and nonlinear dynamics of slightly curved cantilevered pipe conveying fluid[J]. Journal of Sound and Vibration, 2021, 496: 115711. [12] DING H, JIN J C, CHEN L Q. Nonlinear vibration isolation for fluid-conveying pipes using quasi-zero stiffness characteristics[J]. Mechanical Systems and Signal Processing, 2019, 121: 675-688. doi: 10.1016/j.ymssp.2018.11.057 [13] TAN X, DING H, SUN J Q, et al. Primary and super-harmonic resonances of Timoshenko pipes conveying high-speed fluid[J]. Ocean Engineering, 2020, 203: 107258. doi: 10.1016/j.oceaneng.2020.107258 [14] YE S Q, DING H, WEI S, et al. Non-trivial equilibriums and natural frequencies of a slightly curved pipe conveying supercritical fluid[J]. Ocean Engineering, 2021, 227: 108899. doi: 10.1016/j.oceaneng.2021.108899 [15] AKINTOYE O O, OYEDIRAN A A. The effect of various boundary conditions on the nonlinear dynamics of slightly curved pipes under thermal loading[J]. Applied Mathematical Modelling, 2020, 87: 332-350. doi: 10.1016/j.apm.2020.06.019 [16] LI Y D, YANG Y R. Nonlinear vibration of slightly curved pipe with conveying pulsating fluid[J]. Nonlinear Dynamics, 2017, 88(4): 2513-2529. doi: 10.1007/s11071-017-3393-5 [17] 刘燕, 张伟, 龚涛涛. 轴向可伸缩复合材料悬臂梁的非线性振动研究[J]. 动力学与控制学报, 2020, 18(4): 19-25. (LIU Yan, ZHANG Wei, GONG Taotao. Modeling and numerical analysis of an axially moving composite laminated cantilever beam[J]. Journal of Dynamics and Control, 2020, 18(4): 19-25.(in Chinese)LIU Yan, ZHANG Wei, GONG Taotao. Modeling and numerical analysis of an axially moving composite laminated cantilever beam[J]. Journal of Dynamics and Control, 2020, 18(4): 19-25. (in Chinese)) [18] 王松松, 郭翔鹰, 王帅博. 变截面Z型折叠机翼振动特性的有限元与实验分析[J]. 动力学与控制学报, 2020, 18(6): 84-89. (WANG Songsong, GUO Xiangying, WANG Shuaibo. Finite element and experimental analysis of vibration characteristics of variable cross-section Z-form folding wing[J]. Journal of Dynamics and Control, 2020, 18(6): 84-89.(in Chinese)WANG Songsong, GUO Xiangying, WANG Shuaibo. Finite element and experimental analysis of vibration characteristics of variable cross-section Z-form folding wing[J]. Journal of Dynamics and Control, 2020, 18(6): 84-89. (in Chinese)) [19] 李占营, 王建军, 邱明星. 航空发动机空间管路系统的流固耦合振动特性[J]. 航空动力学报, 2016, 31(10): 2346-2352. (LI Zhanying, WANG Jianjun, QIU Mingxing. Dynamic characteristics of aero-engine pipe system considering fluid-structure coupling[J]. Journal of Aerospace Power, 2016, 31(10): 2346-2352.(in Chinese)LI Zhanying, WANG Jianjun, QIU Mingxing. Dynamic characteristics of aero-engine pipe system considering fluid-structure coupling[J]. Journal of Aerospace Power, 2016, 31(10): 2346-2352. (in Chinese)) [20] 李继世, 张大义, 王立, 等. 考虑流体介质影响的管路模态特性分析[J]. 航空动力学报, 2019, 34(3): 671-677. (LI Jishi, ZHANG Dayi, WANG Li, et al. Modal characteristics analysis for pipelines considering influence of fluid medium[J]. Journal of Aerospace Power, 2019, 34(3): 671-677.(in Chinese)LI Jishi, ZHANG Dayi, WANG Li, et al. Modal characteristics analysis for pipelines considering influence of fluid medium[J]. Journal of Aerospace Power, 2019, 34(3): 671-677. (in Chinese)) [21] 胡效东, 梁泽华, 宗丹丹, 等. 湿模态管道振动特性研究[J]. 机械设计与制造, 2021(8): 300-304. (HU Xiaodong, LIANG Zehua, ZONG Dandan, et al. Study on vibration characteristics of wet-mode pipeline[J]. Machinery Design & Manufacture, 2021(8): 300-304.(in Chinese) doi: 10.3969/j.issn.1001-3997.2021.08.067HU Xiaodong, LIANG Zehua, ZONG Dandan, et al. Study on vibration characteristics of wet-mode pipeline[J]. Machinery Design & Manufacture, 2021(8): 300-304. (in Chinese)) doi: 10.3969/j.issn.1001-3997.2021.08.067 [22] 田晓洁, 谢大帅, 刘贵杰, 等. 基于ANSYS的气液两相流海洋立管流固耦合特性分析[J]. 振动与冲击, 2021, 40(7): 260-267. (TIAN Xiaojie, XIE Dashuai, LIU Guijie, et al. Analysis of fluid-structure interaction characteristics of gas-liquid two-phase flow marine riser based on ANSYS[J]. Journal of Vibration and Shock, 2021, 40(7): 260-267.(in Chinese)TIAN XiaoJie, XIE Dashuai, LIU Guijie, et al. Analysis of fluid-structure interaction characteristics of gas-liquid two-phase flow marine riser based on ANSYS[J]. Journal of Vibration and Shock, 2021, 40(7): 260-267. (in Chinese)) -




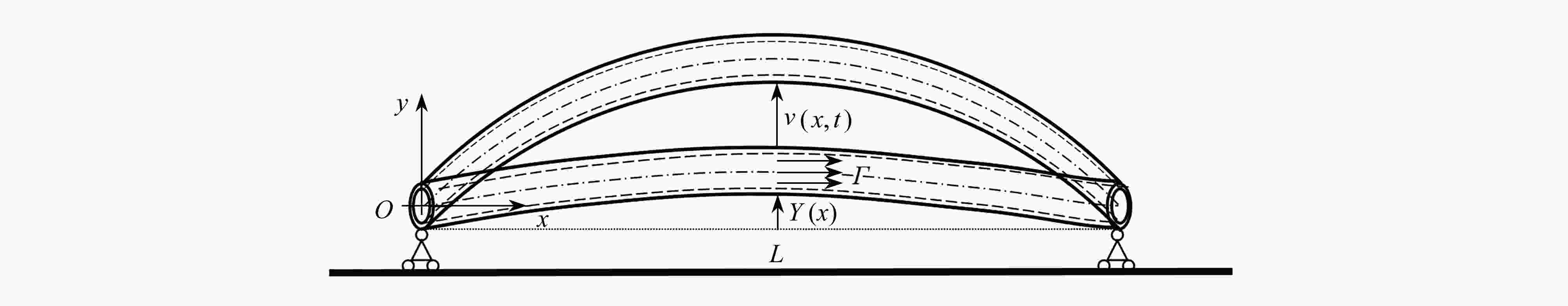
 下载:
下载:
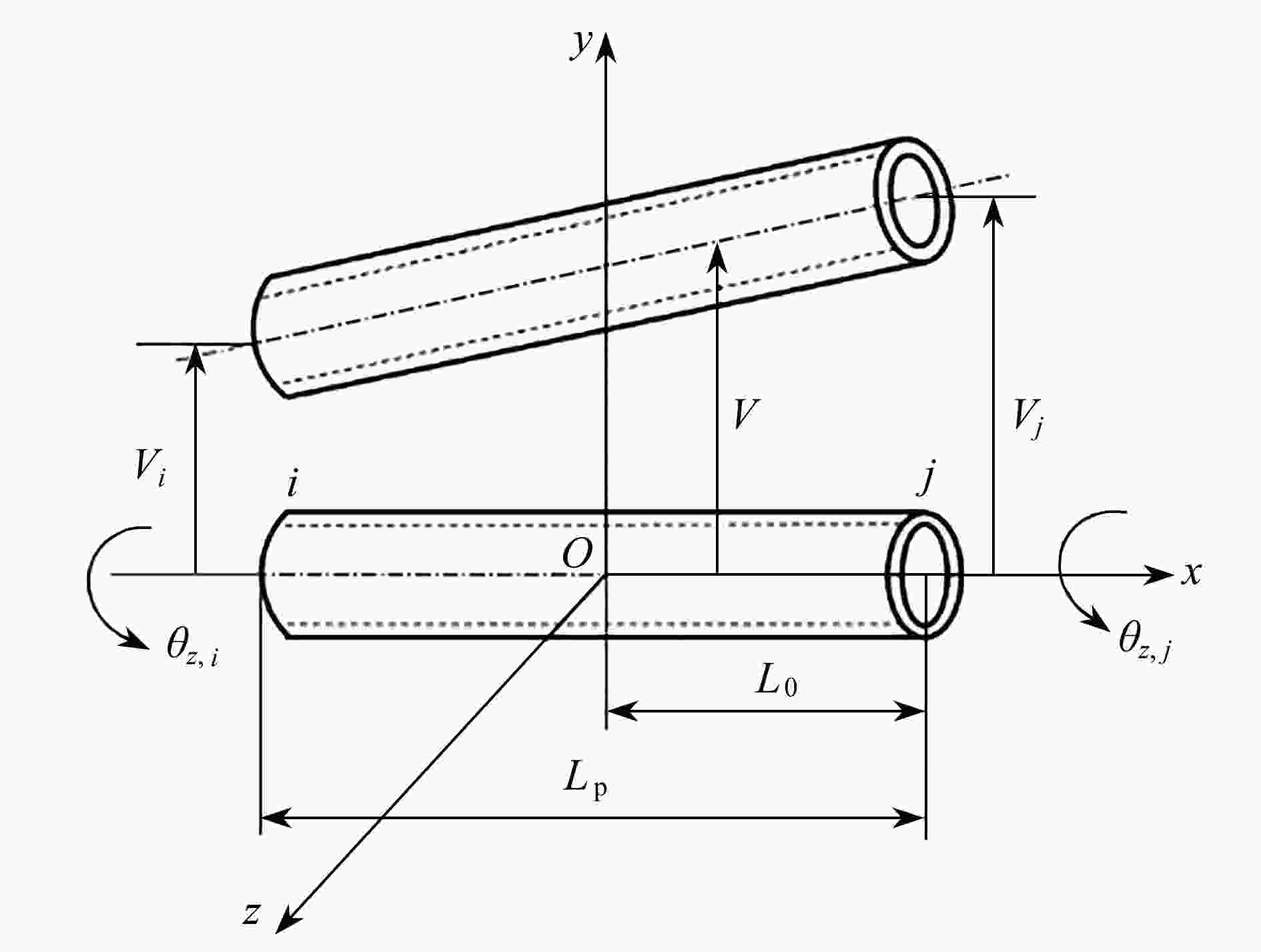
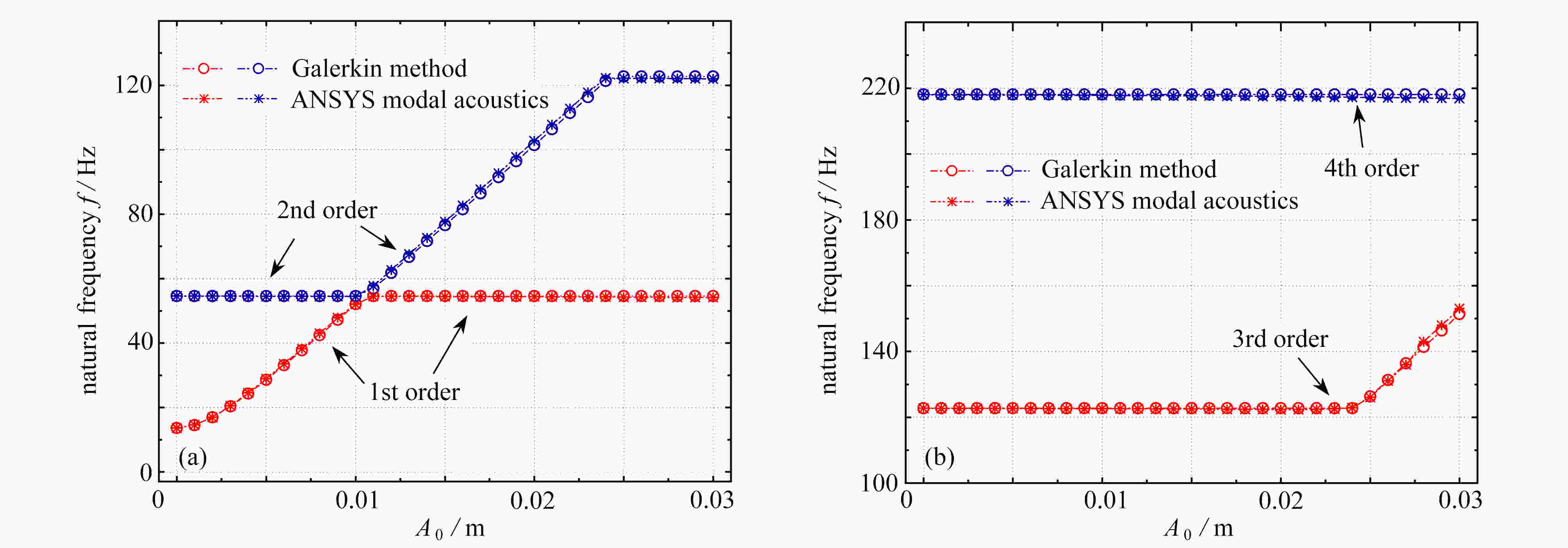
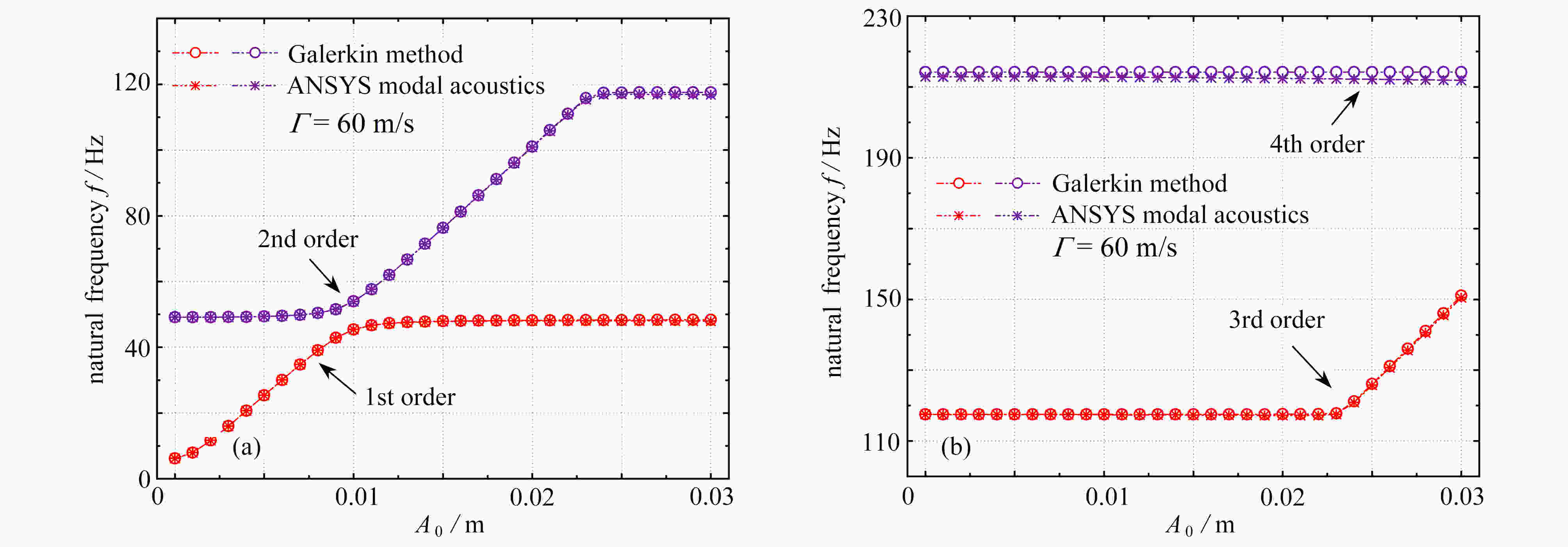


 渝公网安备50010802005915号
渝公网安备50010802005915号