Beat Vibration of X-Configuration Tension Nonlinear Systems Under Resonance Excitation
-
摘要:
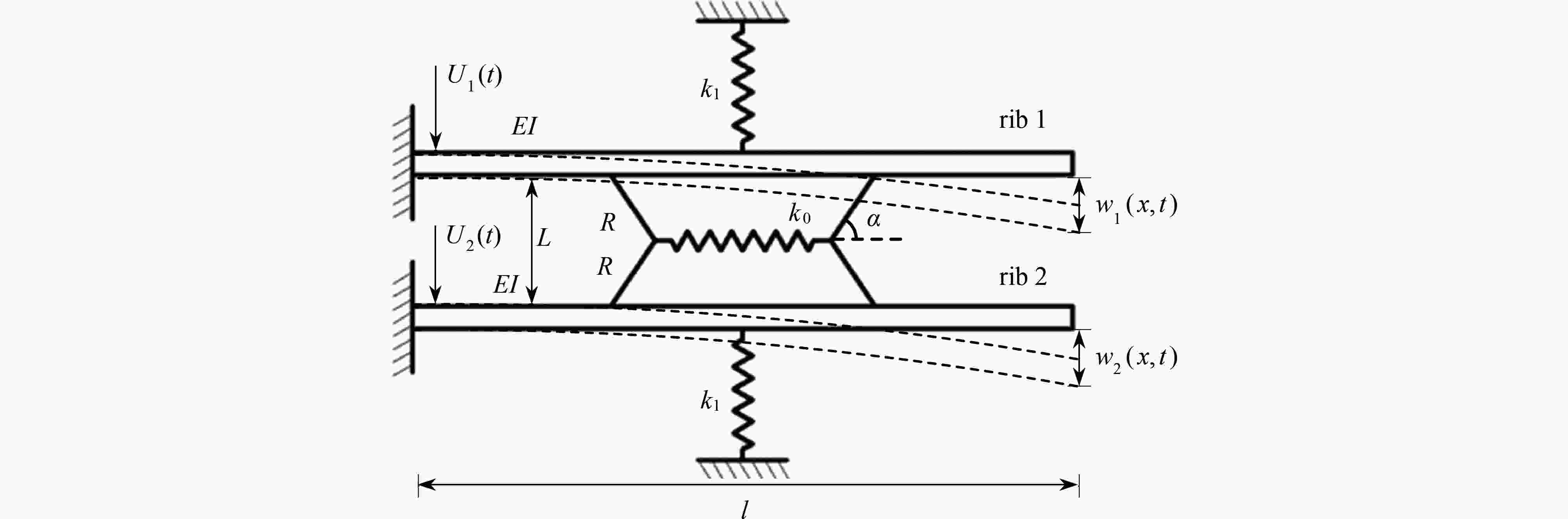
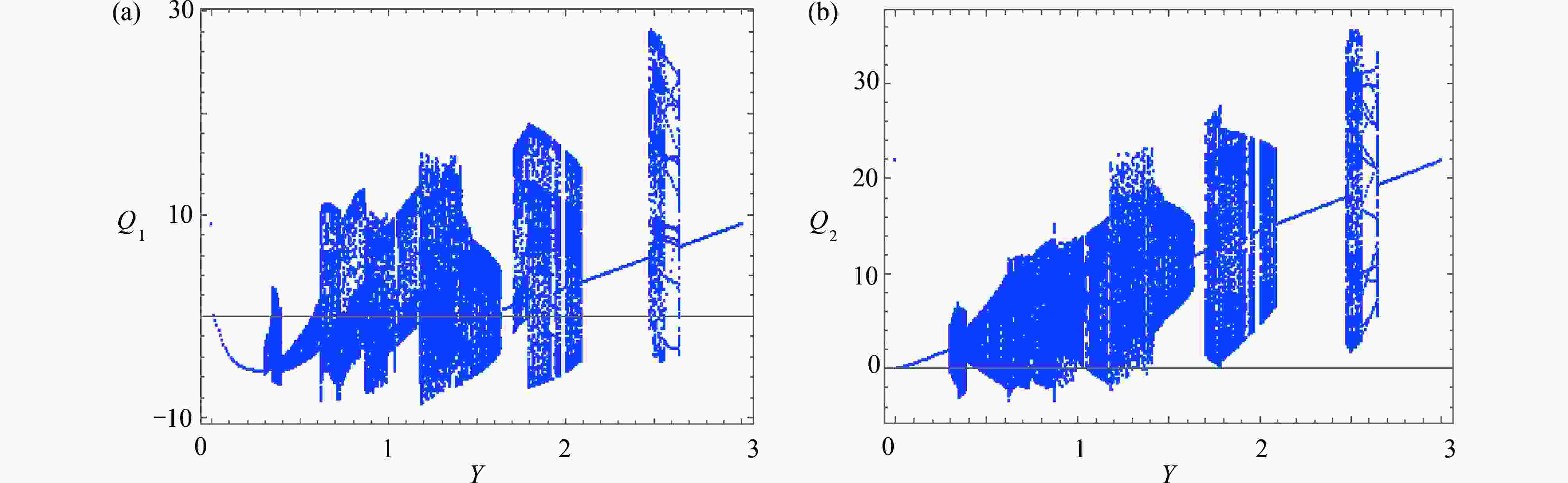
圆形太阳翼因收纳比高、供电能力强等特点受到人们的广泛重视。作为大尺寸薄膜结构，为了调节薄膜张力，通常会引入由绳和弹簧组成的张力调节装置，其力学特性具有强非线性特征，但目前还未有研究讨论其影响。该文提出了一种研究张力影响的机理模型，并利用Lagrange能量法建立了系统二自由度非线性动力学方程，以某工程样机为例，研究了张力机构出现肋板不对称时系统在共振激励下的响应。研究表明，激励幅值变化对系统拍振响应特点有重要影响，使其出现了混沌、概周期以及多倍周期等现象。这些结果对张力机构参数设计有重要参考作用。
Abstract:The circular membrane solar array has attracted extensive attention due to its high storage ratio and strong power supply capability. In order to adjust the tension of large film structures, a tension adjusting device composed of ropes and springs is usually introduced, and its mechanical characteristics are highly nonlinear, with the effects rarely studied yet. Aimed at the tension adjustment, a mechanism model was proposed. The nonlinear dynamics equation for the 2DOF system was established with the Lagrangian energy method. With an engineering prototype as the example, the responses of the tension mechanism with unsymmetrical ribs under resonance excitation were studied. The results show that, the change of the excitation amplitude has an important influence on the characteristics of the beat response of the system. Consequently, the responses of the system exhibit chaotic, almost periodic and multifold periodic phenomena. The research makes an important reference to the parameter design of tension mechanisms.
-
Key words:
- tension adjusting unit /
- nonlinearity /
- resonance /
- beat vibration
-
图 2 激励幅值为0.3线性系统的响应：(a) 肋板1时间历程图；(b) 肋板2时间历程图；(c) 肋板1频谱图；(d) 肋板2频谱图
Figure 2. With an excitation amplitude of 0.3, the linear system responses：(a) the time history diagram of rib 1; (b) the time history diagram of rib 2; (c) the spectrum diagram of rib 1; (d) the spectrum diagram of rib 2
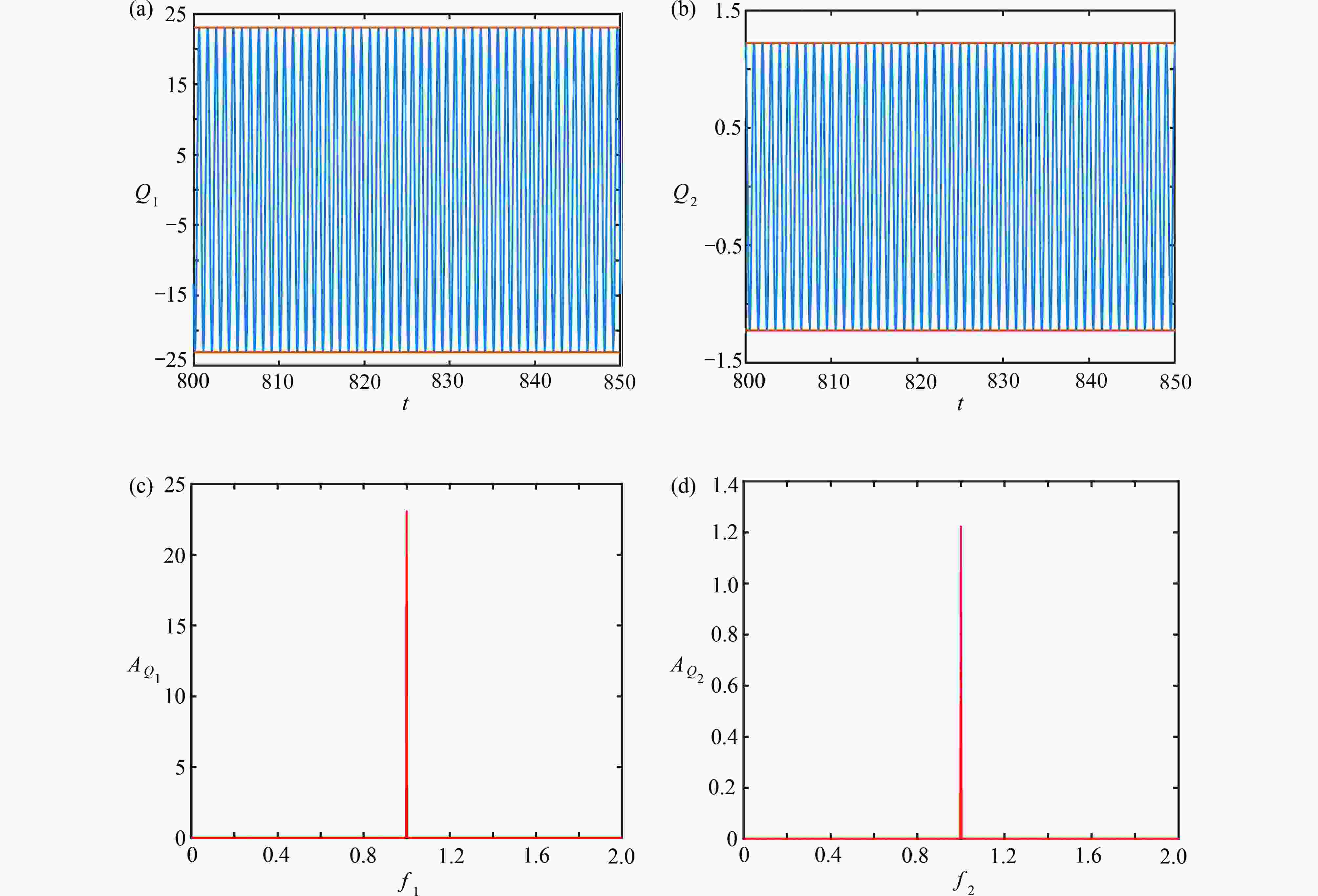
图 3 激励幅值为0.3非线性系统的响应：(a) 肋板1时间历程图；(b) 肋板2时间历程图；(c) 肋板1 Poincaré截面图；(d) 肋板2 Poincaré截面图；(e) 肋板1频谱图；(f) 肋板2频谱图；(g) 肋板1 Hilbert包络谱；(h) 肋板2 Hilbert包络谱
Figure 3. With an excitation amplitude of 0.3, the nonlinear system responses: (a) the time history diagram of rib 1; (b) the time history diagram of rib 2; (c) the Poincaré section diagram of rib 1; (d) the Poincaré section diagram of rib 2; (e) the spectrum diagram of rib 1; (f) the spectrum diagram of rib 2; (g) the Hilbert envelope spectrum diagram of rib 1; (h) the Hilbert envelope spectrum diagram of rib 2
图 4 激励幅值改变下肋板分岔图：(a) 肋板1分岔图；(b) 肋板2分岔图
Figure 4. With a changing excitation amplitude, the bifurcation diagrams of the lower ribs: (a) the bifurcation diagram of rib 1; (b) the bifurcation diagram of rib 2
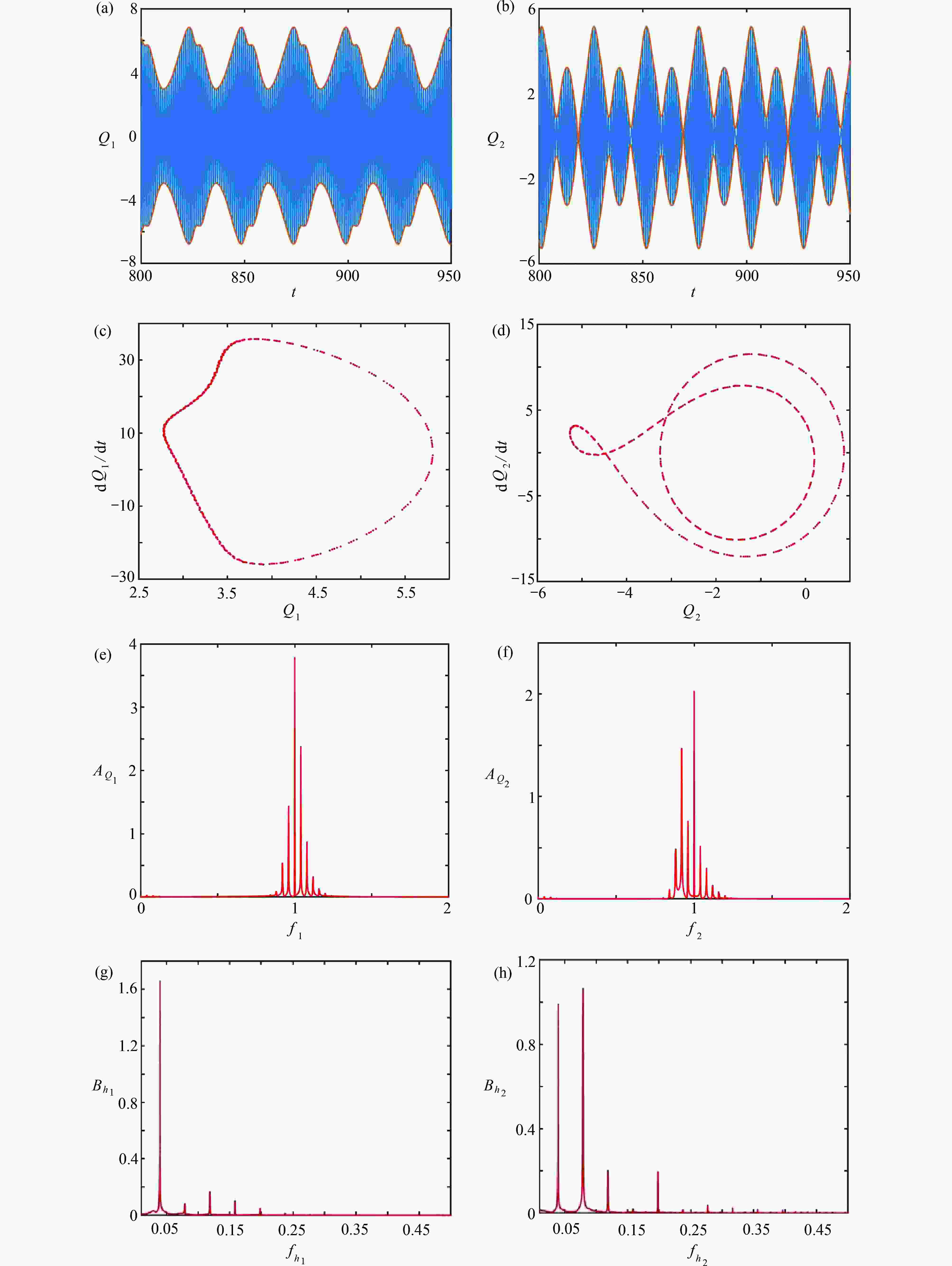
图 5 激励幅值为0.67结构非对称系统的响应：(a) 肋板1时间历程图；(b) 肋板2时间历程图；(c) 肋板1 Poincaré截面图；(d) 肋板2 Poincaré截面图；(e) 肋板1频谱图；(f) 肋板2频谱图；(g) 肋板1 Hilbert包络谱；(h) 肋板2 Hilbert包络谱
Figure 5. With an excitation amplitude of 0.67, the asymmetric-structure system responses: (a) the time history diagram of rib 1; (b) the time history diagram of rib 2; (c) the Poincaré section diagram of rib 1; (d) the Poincaré section diagram of rib 2; (e) the spectrum diagram of rib 1; (f) the spectrum diagram of rib 2; (g) the Hilbert envelope spectrum diagram of rib 1; (h) the Hilbert envelope spectrum diagram of rib 2
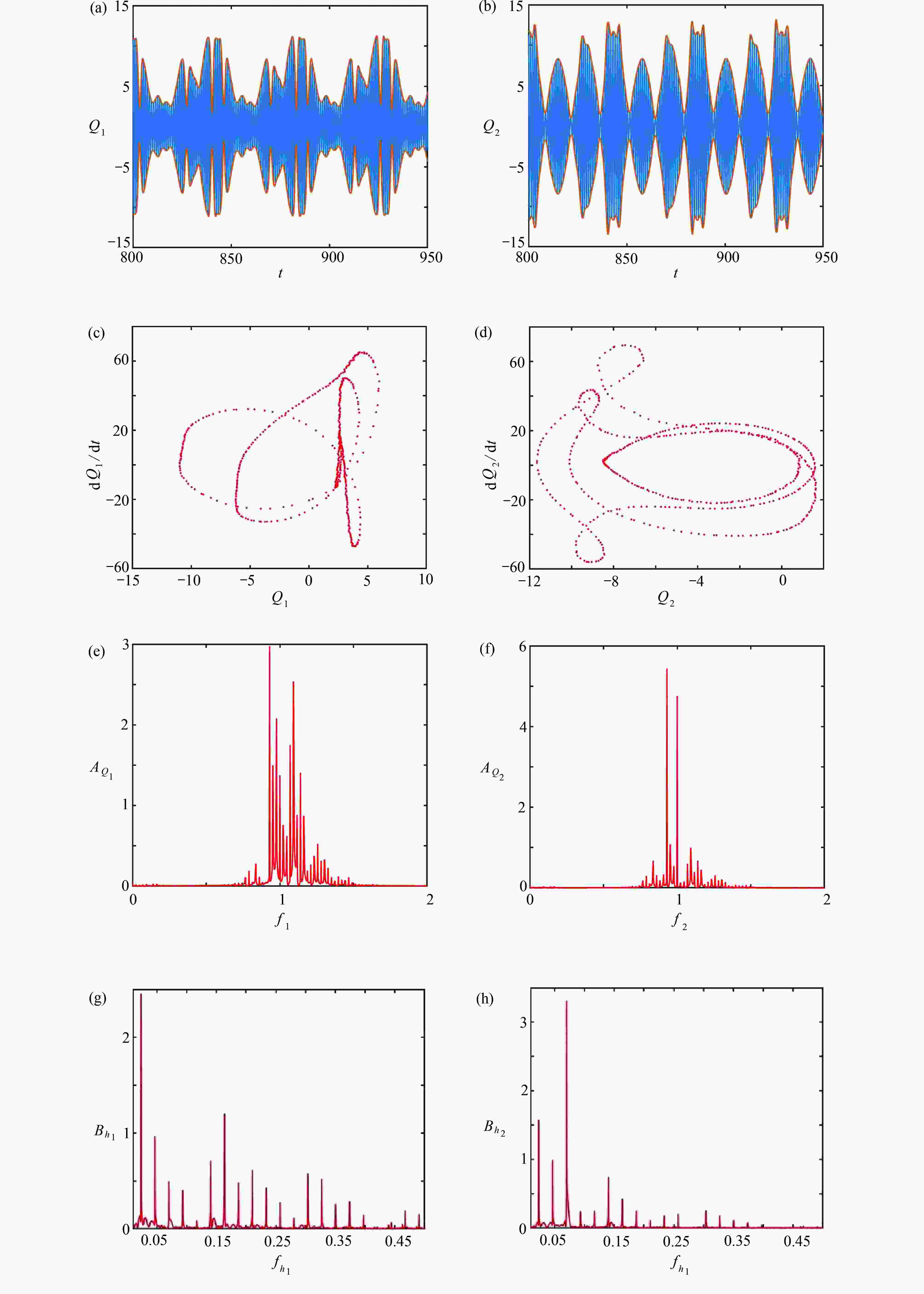
图 6 激励幅值为0.72结构非对称系统的响应：(a) 肋板1时间历程图；(b) 肋板2时间历程图；(c) 肋板1 Poincaré截面图；(d) 肋板2 Poincaré截面图；(e) 肋板1频谱图；(f) 肋板2频谱图；(g) 肋板1 Hilbert包络谱；(h) 肋板2 Hilbert包络谱
Figure 6. With an excitation amplitude of 0.72, the asymmetric-structure system responses: (a) the time history diagram of rib 1; (b) the time history diagram of rib 2; (c) the Poincaré section diagram of rib 1; (d) the Poincaré section diagram of rib 2; (e) the spectrum diagram of rib 1; (f) the spectrum diagram of rib 2; (g) the Hilbert envelope spectrum diagram of rib 1; (h) the Hilbert envelope spectrum diagram of rib 2
图 7 激励幅值为2.66结构非对称系统的响应：(a) 肋板1时间历程图；(b) 肋板2时间历程图；(c) 肋板1 Poincaré截面图；(d) 肋板2 Poincaré截面图；(e) 肋板1频谱图；(f) 肋板2频谱图；(g) 肋板1 Hilbert包络谱；(h) 肋板2 Hilbert包络谱
Figure 7. With an excitation amplitude of 2.66, the asymmetric-structure system responses: (a) the time history diagram of rib 1; (b) the time history diagram of rib 2; (c) the Poincaré section diagram of rib 1; (d) the Poincaré section diagram of rib 2; (e) the spectrum diagram of rib 1; (f) the spectrum diagram of rib 2; (g) the Hilbert envelope spectrum diagram of rib 1; (h) the Hilbert envelope spectrum diagram of rib 2
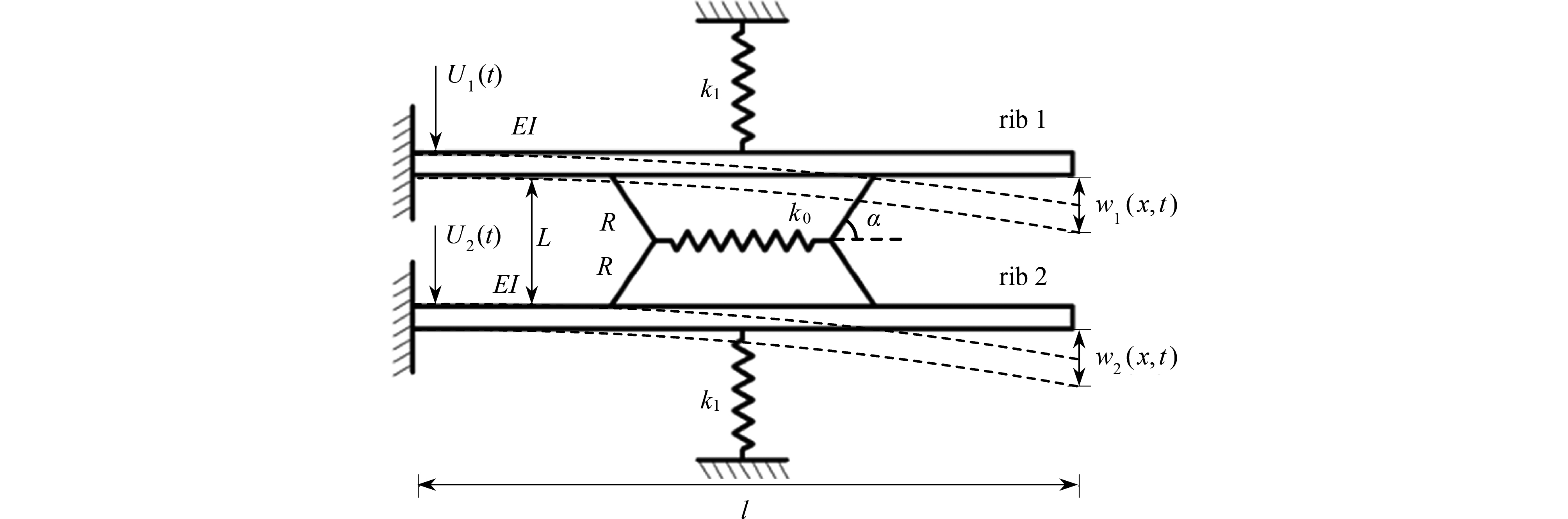
表 1 模型参数取值
Table 1. Values of model parameters
parameter value rib plate length l /m 2.9 elastic modulus E /Pa 7.2 × 1010 rib plate width e /m 0.005 rib plate 1 height h1 /m 0.04 rib plate 2 height h2 /m 0.05 material density ρ/(kg/m3) 435 tension spring stiffness k0/(N/m) 5.59 grounding spring stiffness k1/(N/m) 2900 structural damping C /(N·s·m-1) 0.366 
下载: 导出CSV
-
[1] 周志清, 吴跃民, 王举, 等. 圆形太阳翼发展现状及趋势[J]. 航天器工程, 2015, 24(6): 116-122. (ZHOU Zhiqing, WU Yuemin, WANG Ju, et al. Development and trend of circular solar array[J]. Spacecraft Engineering, 2015, 24(6): 116-122.(in Chinese) doi: 10.3969/j.issn.1673-8748.2015.06.017ZHOU Zhiqing, WU Yuemin, WANG Ju, et al. Development and trend of circular solar array[J]. Spacecraft Engineering, 2015, 24(6): 116-122. (in Chinese)) doi: 10.3969/j.issn.1673-8748.2015.06.017 [2] 温登哲, 陈予恕. 机动飞行时航空发动机的双转子动力学研究综述[C]//中国振动工程学会, 中国力学学会. 第十三届全国非线性振动暨第十届全国非线性动力学和运动稳定性学术会议摘要集. 2011: 6.WEN Dengzhe, CHEN Yushu. A review of the research on two-rotor dynamics of aeroengines in maneuvering flight[C]//Chinese Society of Vibration Engineering, The Chinese Society of Theoretical and Applied Mechanics. Proceedings of the 10th International Conference on Nonlinear Dynamics and Motion Stability. 2011: 6. (in Chinese) [3] 陈茉莉, 李舜酩, 温卫东, 等. 多源拍振分析方法与试验[J]. 振动、测试与诊断, 2011, 31(2): 202-206, 267. (CHEN Moli, LI Shunming, WEN Weidong, et al. Analysis and experiment on multi-source beat vibration[J]. Journal of Vibration, Measurement and Diagnosis, 2011, 31(2): 202-206, 267.(in Chinese)CHEN Moli, LI Shunming, WEN Weidong, et al. Analysis and experiment on multi-source beat vibration[J]. Journal of Vibration, Measurement and Diagnosis, 2011, 31(2): 202-206, 267. (in Chinese)) [4] 廖明夫, 于潇, 王四季, 等. 双转子系统的振动[J]. 机械科学与技术, 2013, 32(4): 475-480. (LIAO Mingfu, YU Xiao, WANG Siji, et al. The vibration features of a twin spool rotor system[J]. Mechanical Science and Technology for Aerospace Engineering, 2013, 32(4): 475-480.(in Chinese)LIAO Mingfu, YU Xiao, WANG Siji, et al. The vibration features of a twin spool rotor system[J]. Mechanical Science and Technology for Aerospace Engineering, 2013, 32(4): 475-480. (in Chinese)) [5] 韩军, 高德平, 胡绚, 等. 航空发动机双转子系统的拍振分析[J]. 航空学报, 2007, 28(6): 1369-1373. (HAN Jun, GAO Deping, HU Xuan, et al. Research on beat vibration of dual-rotor for aero-engine[J]. Acta Aeronautica et Astronautica Sinica, 2007, 28(6): 1369-1373.(in Chinese) doi: 10.3321/j.issn:1000-6893.2007.06.017HAN Jun, GAO Deping, HU Xuan, et al. Research on beat vibration of dual-rotor for aero-engine[J]. Acta Aeronautica et Astronautica Sinica, 2007, 28(6): 1369-1373. (in Chinese)) doi: 10.3321/j.issn:1000-6893.2007.06.017 [6] ZENG S, WANG X X. Unbalance identification and field balancing of dual rotors system with slightly different rotating speeds[J]. Journal of Sound and Vibration, 1999, 220(2): 343-351. doi: 10.1006/jsvi.1998.1955 [7] 高天. 机动飞行环境下航空发动机转子系统瞬态动力学特性研究[D]. 博士学位论文. 天津: 天津大学, 2021.GAO Tian. Research on transient dynamic characteristics of aeroengine rotor systems under maneuvering flight environment[D]. PhD Thesis. Tianjin: Tianjin University, 2021. (in Chinese) [8] CAETANO E, CUNHA A, GATTULLI V, et al. Cable-deck dynamic interactions at the International Guadiana Bridge: onsite measurements and finite element modelling[J]. Structural Control and Health Monitoring, 2008, 15(3): 237-264. doi: 10.1002/stc.241 [9] 孙测世, 赵珧冰, 康厚军, 等. 斜拉桥的多重内共振及其耦合过程研究[J]. 振动与冲击, 2018, 37(10): 87-93. (SUN Ceshi, ZHAO Yaobing, KANG Houjun, et al. Multiple internal resonances and coupling process of cable-stayed bridge[J]. Journal of Vibration and Shock, 2018, 37(10): 87-93.(in Chinese)SUN Ceshi, ZHAO Yaobing, KANG Houjun, et al. Multiple internal resonances and coupling process of cable-stayed bridge[J]. Journal of Vibration and Shock, 2018, 37(10): 87-93. (in Chinese)) [10] PARK J, LEE J, AHN S, et al. Reduced ride comfort caused by beating idle vibrations in passenger vehicles[J]. International Journal of Industrial Ergonomics, 2017, 57: 74-79. doi: 10.1016/j.ergon.2016.12.003 [11] 朱剑涛, 刘晨, 朱位, 等. 星箭组合体主动段飞行中拍频振动分析[C]//中国振动工程学会, 南京航空航天大学机械结构力学及控制国家重点实验室. 第十二届全国振动理论及应用学术会议论文集. 2017: 9.ZHU Jiantao, LIU Chen, ZHU Wei, et al. Analysis of beat frequency vibration characteristics of active flight section of satellite-rocket combination[C]//Chinese Society of Vibration Engineering, State Key Laboratory of Mechanics and Control of Mechanical Structures, Nanjing University of Aeronautics and Astronautics. Proceedings of the 12th National Conference on Vibration Theory and Application. 2017: 9. (in Chinese) [12] 练继建, 李成业, 刘昉, 等. 环境激励下二滩拱坝拍振机理的研究[J]. 振动与冲击, 2012, 31(3): 1-7.LIAN Jijian, LI Chengye, LIU Fang, et al. Beat vibration of ertan arch dam under ambient excitation[J]. Journal of Vibration and Shock, 2012, 31(3): 1-7. (in Chinese) [13] ZHANG Junshi, CHEN Hualing. Voltage-induced beating vibration of a dielectric elastomer membrane[J]. Nonlinear Dynamics, 2020, 100: 2225-2239. doi: 10.1007/s11071-020-05678-4 [14] ENDO H, SUZUKI H. Beating vibration phenomenon of a very large floating structure[J]. Journal of Marine Science and Technology, 2018, 23(3): 662-677. doi: 10.1007/s00773-017-0502-6 [15] KIM S H, LEE C W, LEE J M. Beat characteristics and beat maps of the King Seong-Deok Divine Bell[J]. Journal of Sound and Vibration, 2005, 281(1/2): 21-44. [16] KIM S H, SOETEL W, LEE J M. Analysis of the beating response of bell type structures[J]. Journal of Environmental Research, 1994, 173(4): 517-536. [17] 高辉, 徐龙祥. 主动磁悬浮轴承系统拍振现象分析[J]. 机械工程学报, 2011, 47(13): 104-112. (GAO Hui, XU Longxiang. Analysis of beat vibration for active magnetic bearing system[J]. Journal of Mechanical Engineering, 2011, 47(13): 104-112.(in Chinese)GAO Hui, XU Longxiang. Analysis of beat vibration for active magnetic bearing system. Journal of mechanical engineering, 2011, 47(13): 104-112. (in Chinese)) -
计量
- 文章访问数: 578
- HTML全文浏览量: 276
- PDF下载量: 92
- 被引次数: 0
渝公网安备50010802005915号