Research on Constraint Following Control of Flexible Joint Manipulators Based on Singular Perturbation
-
摘要: 针对二连杆柔性关节机械臂，提出了一种基于奇异摄动理论和Udwadia-Kalaba(U-K)方法的控制方法. 设计步骤主要分为两步：第一，基于奇异摄动法对系统进行降阶，把系统拆分为快、慢系统，不仅降低了求解系统的阶次，而且克服了系统柔性；第二，基于U-K方法设计了快、慢系统的状态反馈约束跟随控制律，能使快、慢系统约束跟随误差收敛到零，即使系统初始不满足约束条件，该方法不需要借助Lagrange乘子和伪广义速度等辅助变量，可以同时处理完整约束和非完整约束. 将以上方法运用在二连杆柔性关节机械臂系统中，解决了二连杆柔性关节机械臂的柔性振荡和约束跟随的问题. 使用MATLAB进行仿真，并且与传统PID控制进行了对比，验证了所提出的方法的有效性与优越性.Abstract: For the control of 2-link flexible joint manipulators, a control method based on the singular perturbation theory and the Udwadia-Kalaba (U-K) method was proposed. The control design was implemented by 2 steps. First, the system order was reduced based on the singular perturbation method and the system was divided into fast and slow sub-systems, to simplify the solution process and overcome the system flexibility. Second, the state feedback constraint following control law for the fast and slow sub-systems was designed with the U-K method, to make the constraint following errors of the fast and slow sub-systems converge to zero, even if the system can't initially satisfy the constraints. The proposed method can deal with holonomic and nonholonomic servo constraints at the same time without the auxiliary variables of the Lagrange multiplier and the pseudo generalized velocity. The method was applied to 2-link flexible joint manipulator systems to solve the flexible oscillation and constraint following problems. Through simulations on MATLAB, and was compared with the traditional PID control, to verify the effectiveness and superiority.
-
图 5 转杆1的角速度$ \dot{q}^{(2)}$
Figure 5. The angular velocity $ \dot{q}^{(2)}$ of rotary rod 1
图 6 转杆2的角速度$ \dot{q}^{(4)}$
Figure 6. The angular velocity $ \dot{q}^{(4)}$ of rotary rod 2
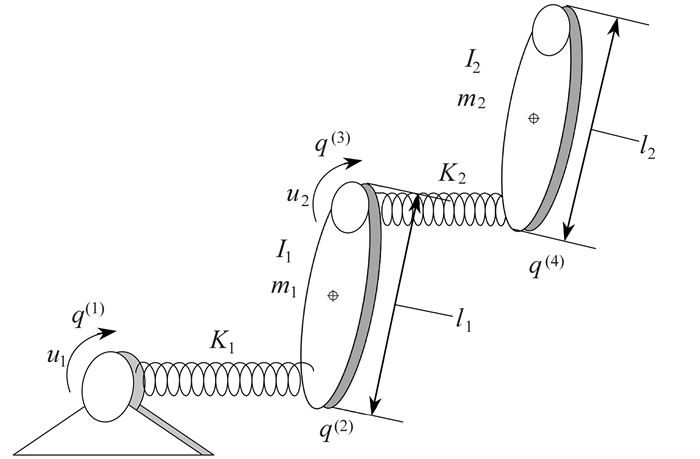
表 1 二连杆柔性机械臂参数
Table 1. Parameters of the 2-link flexible manipulator
parameter symbol value moment of inertia of joint 1 J1/(kg·m2) 0.135 elastic coefficient of joint 1 K1/(N·m/rad) 10 000 moment of inertia of joint 2 J2/(kg·m2) 0.150 elastic coefficient of joint 2 K2/(N·m/rad) 10 000 mass of link 1 m1/kg 8 length of link 1 l1/m 0.6 center position of link 1 lc1/m 0.3 mass of link 2 m2/kg 8 length of link 2 l2/m 0.6 center position of link 2 lc2/m 0.3 
下载: 导出CSV
-
[1] YANG C G, JIANG Y M, HE W, et al. Adaptive parameter estimation and control design for robot manipulators with finite-time convergence[J]. IEEE Transactions on Industrial Electronics, 2018, 65(10): 8112-8123. doi: 10.1109/TIE.2018.2803773 [2] 刘建昌, 苗宇. 基于神经网络补偿的机械臂轨迹控制策略的研究[J]. 控制与决策, 2005, 20(7): 732-736. https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC200507002.htmLIU Jianchang, MIAO Yu. Research on trajectory control strategy of manipulator based on neural network compensation[J]. Control and Decision, 2005, 20(7): 732-736. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC200507002.htm [3] 陈强, 丁科新, 南余荣. 带有输出约束的柔性关节机械臂预设性能自适应控制[J]. 控制与决策, 2021, 36(2): 387-394. https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC202102014.htmCHEN Qiang, DING Kexin, NAN Yurong. Prescribed performance adaptive control of flexible-joint manipulators with output constraints[J]. Control and Decision, 2021, 36(2): 387-394. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KZYC202102014.htm [4] YOO S J, PARK J B, CHOI Y H. Adaptive output feedback control of flexible-joint robots using neural networks: dynamic surface design approach[J]. IEEE Transactions on Neural Networks, 2008, 19(10): 1712-1726. doi: 10.1109/TNN.2008.2001266 [5] LING S, WANG H Q, LIU P X. Adaptive fuzzy dynamic surface control of flexible-joint robot systems with input saturation[J]. CAA Journal of Automatica Sinica, 2019, 6(1): 97-107. doi: 10.1109/JAS.2019.1911330 [6] LIU X, ZHAO F, GE S S, et al. End-effector force estimation for flexible-joint robots with global friction approximation using neural networks[J]. IEEE Transactions on Industrial Informatics, 2019, 15(3): 1730-1741. doi: 10.1109/TII.2018.2876724 [7] 刘华平, 孙富春, 何克忠, 等. 奇异摄动控制系统: 理论与应用[J]. 控制理论与应用, 2003, 20(1): 1-7. https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY200501017.htmLIU Huaping, SUN Fuchun, HE Kezhong, et al. Survey of singularly perturbed control systems: theory and applications[J]. Control Theory & Applications, 2003, 20(1): 1-7. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY200501017.htm [8] SPONG M W. Modeling and control of elastic joint robots[J]. Journal of Dynamic Systems Measurement and Control, 1987, 109(4): 310-318. doi: 10.1115/1.3143860 [9] 洪昭斌, 陈力. 柔性空间机械臂基于奇异摄动法的鲁棒跟踪控制和柔性振动主动控制[J]. 工程力学, 2010, 27(8): 191-198. https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201008034.htmHONG Zhaobin, CHEN Li. Robust control and active vibration control of space flexible manipulator by singular perturbation approach[J]. Engineering Mechanics, 2010, 27(8): 191-198. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-GCLX201008034.htm [10] 刘华山, 金元林, 程新, 等. 力矩输入有界的柔性关节机器人轨迹跟踪控制[J]. 控制理论与应用, 2019, 36(6): 983-992. https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201906017.htmLIU Huashan, JIN Yuanlin, CHENG Xin, et al. Trajectory tracking control for flexible-joint robot manipulators with bounded torque inputs[J]. Control Theory & Applications, 2019, 36(6): 983-992. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY201906017.htm [11] KALABA R E, UDWADIA F E. Equations of motion for nonholonomic, constrained dynamical systems via Gauss's principle[J]. Journal of Applied Mechanics, 1993, 60(3): 662-668. [12] KALABA R, UDWADIA F. Analytical dynamics with constraint forces that do work in virtual displacements[J]. Applied Mathematics and Computation, 2001, 121(2/3): 211-217. http://ame-www.usc.edu/bio/udwadia/papers/Analytical_dynamics_with_constraint_forces.pdf [13] UDWADIA F E, KALABA R E, PHOHOMSIRI P. Mechanical systems with nonideal constraints: explicit equations without the use of generalized inverses[J]. Journal of Applied Mechanics, 2004, 71(5): 615-621. http://pdfs.semanticscholar.org/05dd/803f963ad29631268112a90d36548a857466.pdf [14] WANICHANON T, CHO H, UDWADIA F E. An approach to the dynamics and control of uncertain multi-body systems[J]. Procedia IUTAM, 2015, 13: 43-52. http://www.onacademic.com/detail/journal_1000037990750810_022a.html [15] KOGANTI P B, UDWADIA F E. Unified approach to modeling and control of rigid multibody systems[J]. Journal of Guidance, Control, and Dynamics, 2016, 39(12): 2683-2698. http://ruk.usc.edu/bio/udwadia/papers/Unified%20Approach%20to%20Modeling%20and%20Control%20of%20Rigid%20Multibody%20Systems.pdf [16] CHEN Y H. Constraint-following servo control design for mechanical systems[J]. Journal of Vibration and Control, 2009, 15(3): 369-389. http://www.onacademic.com/detail/journal_1000037457536410_0164.html [17] CHEN Y H, ZHANG X R. Adaptive robust approximate constraint-following control for mechanical systems[J]. Journal of the Franklin Institute, 2010, 347(1): 69-86. http://www.sciencedirect.com/science?_ob=ShoppingCartURL&_method=add&_eid=1-s2.0-S0016003209001392&originContentFamily=serial&_origin=article&_ts=1432638248&md5=e2e5e3313172278551e81d64accf2fc5 [18] 李旻, 熊亮, 尹辉, 等. 含不等式约束的欠驱动系统约束跟随控制[J]. 控制理论与应用, 2020, 37(9): 1971-1982. https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY202009011.htmLI Min, XIONG Liang, YIN Hui, et al. Constraint-following control for underactuated systems with inequality constraints[J]. Control Theory & Applications, 2020, 37(9): 1971-1982. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-KZLY202009011.htm [19] YIN H, CHEN Y H, YU D. Controlling an underactuated two-wheeled mobile robot: a constraint-following approach[J]. Journal of Dynamic Systems Measurement and Control, 2019, 141(7): 1002-1011. [20] 韩江, 汪鹏, 董方方, 等. 基于Udwadia-Kalab方法的并联机器人鲁棒伺服约束控制[J]. 应用数学和力学, 2021, 42(3): 264-274. doi: 10.21656/1000-0887.410197HAN Jiang, WANG Peng, DONG Fangfang, et al. Robust servo constrained control of parallel robots based on the Udwadia-Kalaba method[J]. Applied Mathematics and Mechanics, 2021, 42(3): 264-274. (in Chinese) doi: 10.21656/1000-0887.410197 [21] 韩江, 汪鹏, 董方方, 等. 基于Udwadia-Kalaba方法的平面冗余并联机器人建模与轨迹跟踪控制[J]. 应用数学和力学, 2020, 41(11): 1183-1196. doi: 10.21656/1000-0887.400363HAN Jiang, WANG Peng, DONG Fangfang, et al. Modeling and control of planar redundant parallel robots based on the Udwadia-Kalaba method[J]. Applied Mathematics and Mechanics, 2020, 41(11): 1183-1196. (in Chinese) doi: 10.21656/1000-0887.400363 [22] ZHAO X M, CHEN Y H, ZHAO H, et al. Udwadia-Kalaba equation for constrained mechanical systems: formulation and applications[J]. Chinese Journal of Mechanical Engineering, 2018, 31(6): 11-24. doi: 10.1186/s10033-018-0310-x/fulltext.html [23] HAN J, CHEN Y H, ZHAO X M, et al. Optimal design for robust control of uncertain flexible joint manipulators: a fuzzy dynamical system approach[J]. International Journal of Control, 2018, 91(4): 947-951. http://www.onacademic.com/detail/journal_1000039833815510_e483.html -
图(11) / 表(1)
计量
- 文章访问数: 525
- HTML全文浏览量: 171
- PDF下载量: 65
- 被引次数: 0
渝公网安备50010802005915号