横向非均匀温度场作用的FGM夹层圆板热屈曲分析

龚雪蓓; 赵伟东; 郭冬梅

doi:10.21656/1000-0887.430094

横向非均匀温度场作用的FGM夹层圆板热屈曲分析

doi: 10.21656/1000-0887.430094

龚雪蓓^{1, 2,},
赵伟东^{1, 2, ,},
郭冬梅^{1, 2}

1.
青海大学土木工程学院，西宁 810016
2.
青海省建筑节能材料与工程安全重点实验室，西宁 810016

基金项目:

国家自然科学基金项目 52168053

详细信息

作者简介:
龚雪蓓(1999—)，女，硕士生(E-mail: 754928145@qq.com)

通讯作者:
赵伟东(1972—)，男，副教授，硕士，硕士生导师(通讯作者. E-mail: zhwd.xbl@163.com)

中图分类号: TU43
计量
- 文章访问数: 435
- HTML全文浏览量: 151
- PDF下载量: 45
- 被引次数: 0
出版历程
- 收稿日期: 2022-03-21
- 修回日期: 2022-05-05
- 刊出日期: 2023-04-01

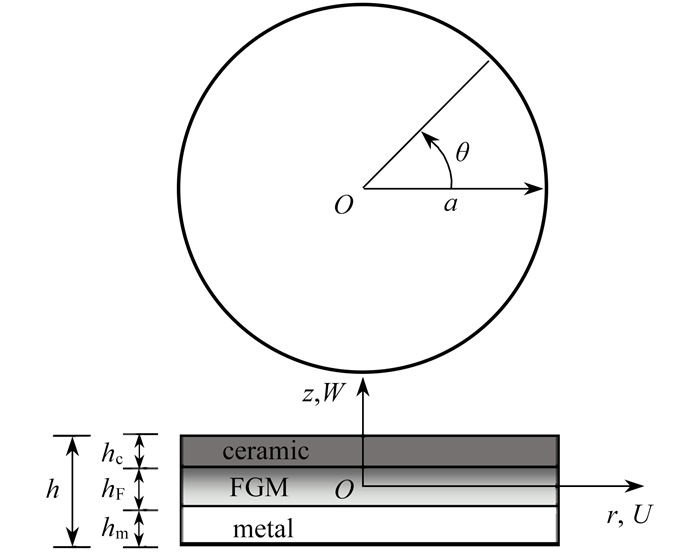
Thermal Buckling Analysis of FGM Sandwich Circular Plates Under Transverse Nonuniform Temperature Field Actions

GONG Xuebei^{1, 2
,},
ZHAO Weidong^{1, 2
, ,},
GUO Dongmei^{1, 2}

1.
School of Civil Engineering, Qinghai University, Xining 810016, P.R.China
2.
Qinghai Provincial Key Laboratory of Energy-Saving Building Materials and Engineering Safety, Xining 810016, P.R.China

摘要

摘要: 基于von Kármán几何非线性板理论，导出了受横向非均匀温度场作用的幂律型功能梯度材料(FGM)夹层圆板的位移型几何非线性控制方程. 考虑不可移夹紧边界条件，通过求解线性特征值问题，得到了系统的有量纲临界屈曲温度差解析公式. 另外，运用打靶法计算了非线性常微分方程两点边值问题. 考察了几何参数、组分材料特性、梯度指数、温度场参数和层厚比对FGM夹层圆板的临界屈曲温度差、热过屈曲平衡路径和平衡构形的影响. 当厚径比、梯度层相对厚度和梯度指数增加时，FGM夹层圆板临界屈曲温度差均单调增加；当半径和总厚度给定时，随FGM层相对厚度增加，FGM夹层圆板后屈曲变形显著减小.
- 功能梯度材料 /
- 夹层圆板 /
- 非均匀温度场 /
- 热屈曲 /
- 打靶法
Abstract: Based on the von Kármán geometric nonlinear plate theory, the displacement-type geometric nonlinear governing equations for FGM sandwich circular plates under transverse nonlinear temperature field actions were derived. With the immovable clamped boundary condition, the analytical formula for dimensional critical buckling temperature differences of the system was obtained from the solution of the linear eigenvalue problem. Moreover, the 2-point boundary value problem of ordinary differential equations was solved with the shooting method. The effects of geometric parameters, constituent material properties, gradient indexes, temperature field parameters and layer-thickness ratios on the critical buckling temperature differences, the thermal postbuckling equilibrium paths, and the buckling equilibrium configurations of FGM sandwich circular plates, were investigated. The results show that, with the increases of the thickness-radius ratio, the relative thickness of the FGM layer and the gradient index, the FGM sandwich circular plate's critical buckling temperature difference will increase monotonically. Given a fixed radius and a fixed total thickness, the postbuckling deformation of the FGM sandwich circular plate will decrease significantly with the relative thickness of the FGM layer.
- FGM /
- sandwich circular plate /
- nonuniform temperature field /
- thermal buckling /
- shooting method

HTML全文

图 1 FGM夹层圆板的几何和坐标系

Figure 1. Geometry and the coordinate system of FGM sandwich circular plates

下载: 全尺寸图片幻灯片

图 2 不同的温度级数项数目对应的FGM夹层圆板热屈曲平衡路径

Figure 2. Thermal buckling equilibrium paths of FGM sandwich circular plates corresponding to different numbers of temperature series terms

下载: 全尺寸图片幻灯片

图 3 厚径比对FGM夹层圆板的热过屈曲平衡路径的影响

Figure 3. Effects of thickness-radius ratios on thermal postbuckling equilibrium paths of FGM sandwich circular plates

下载: 全尺寸图片幻灯片

图 4 梯度指数对FGM夹层圆板的热过屈曲平衡路径的影响

Figure 4. Effects of gradient indexes on thermal postbuckling equilibrium paths of FGM sandwich circular plates

下载: 全尺寸图片幻灯片

图 5 层厚比对FGM夹层圆板的热过屈曲平衡路径的影响

Figure 5. Effects of the layer-thickness ratios on thermal postbuckling equilibrium paths of FGM sandwich circular plates

下载: 全尺寸图片幻灯片

图 6 不同厚度下FGM夹层圆板的热过屈曲平衡构形

Figure 6. Thermal postbuckling equilibrium configurations of FGM sandwich circular plates with different thicknesses

下载: 全尺寸图片幻灯片

图 7 不同层厚比的FGM夹层圆板的热过屈曲平衡构形

Figure 7. Thermal postbuckling equilibrium configurations of FGM sandwich circular plates with different layer-thickness ratios

下载: 全尺寸图片幻灯片

图 8 不同梯度指数的FGM夹层圆板的热过屈曲平衡构形

Figure 8. Thermal postbuckling equilibrium configurations of FGM sandwich circular plates with different gradient indices

下载: 全尺寸图片幻灯片

表 1 金属和陶瓷组分的材料特性

Table 1. Material properties of metal and ceramic

material property	aluminum(Al)	ceramic(Al₂O₃)
elastic modulus E/GPa	70	380
thermal expansion coefficient α/℃^-1	2.3×10^-5	7.4×10^-6
thermal conductivity K/(W/mK)	204	10.4
Poisson’s ratio μ	0.3	0.3

下载: 导出CSV

表 2 具有不同厚径比和梯度指数的FGM圆板在T_l=0 ℃时对应的临界屈曲温度差ΔT_cr

Table 2. Critical buckling temperature difference ΔT_cr of FGM circular plates with different thickness-radius ratios and gradient indexes for T_l=0 ℃

k		h/a
k		0.05	0.04	0.03	0.02	0.01
0	solution in ref. [24]	635.828	405.821	228.898	101.590	25.433
0	present solution	636.000	407.040	228.960	101.760	25.440
0.5	solution in ref. [24]	475.230	304.146	171.083	76.037	19.009
0.5	present solution	475.061	304.039	171.021	76.009	19.002
1	solution in ref. [24]	384.600	246.153	138.456	61.536	15.384
1	present solution	384.453	246.050	138.433	61.512	15.378

下载: 导出CSV

参考文献(24)

[1]	NAJAFIZADEH M M, ESLAMI M R. First-order-theory-based thermoelastic stability of functionally graded material circular plates[J]. AIAA Journal, 2012, 40(7): 1444-1450.
[2]	REDDY J N, CHIN C D. Thermomechanical analysis of functionally graded cylinders and plates[J]. Journal of Thermal Stresses, 1998, 21(6): 593-626. doi: 10.1080/01495739808956165
[3]	SHEN H S. Nonlinear bending response of functionally graded plates subjected to transverse loads and in thermal environments[J]. International Journal of Mechanical Sciences, 2002, 44(3): 561-584. doi: 10.1016/S0020-7403(01)00103-5
[4]	VAN DO V N, CHANG K H, LEE C H. Post-buckling analysis of FGM plates under in-plane mechanical compressive loading by using a mesh-free approximation[J]. Archive of Applied Mechanics, 2019, 89(7): 1421-1446. doi: 10.1007/s00419-019-01512-5
[5]	MA L S, WANG T J. Nonlinear bending and post-buckling of a functionally graded circular plate under mechanical and thermal loading[J]. International Journal of Solids and Structures, 2003, 40(13/14): 3311-3330.
[6]	ZHANG D G, ZHOU H M. Mechanical and thermal post-buckling analysis of FGM rectangular plates with various supported boundaries resting on nonlinear elastic foundations[J]. Thin-Walled Structures, 2015, 89: 142-151.
[7]	LEE W H, HAN S C, PARK W T. A refined higher order shear and normal deformation theory for E-, P-, and S-FGM plates on Pasternak elastic foundation[J]. Composite Structures, 2015, 122: 330-342. doi: 10.1016/j.compstruct.2014.11.047
[8]	陈明飞, 刘坤鹏, 靳国永, 等. 面内功能梯度三角形板等几何面内振动分析[J]. 应用数学和力学, 2020, 41(2): 156-170. doi: 10.21656/1000-0887.400171 CHEN Mingfei, LIU Kunpeng, JIN Guoyong, et al. Isogeometric in-plane vibration analysis of functionally graded triangular plates[J]. Applied Mathematics and Mechanics, 2020, 41(2): 156-170. (in Chinese) doi: 10.21656/1000-0887.400171
[9]	SHEN H S, LI S R. Postbuckling of sandwich plates with FGM face sheets and temperature-dependent properties[J]. Composites (Part B): Engineering, 2008, 39(2): 332-344. doi: 10.1016/j.compositesb.2007.01.004
[10]	ZENKOUR A M, SOBHY M. Thermal buckling of various types of FGM sandwich plates[J]. Composite Structures, 2010, 93(1): 93-102. doi: 10.1016/j.compstruct.2010.06.012
[11]	WANG Z X, SHEN H S. Nonlinear analysis of sandwich plates with FGM face sheets resting on elastic foundations[J]. Composite Structures, 2011, 93(10): 2521-2532. doi: 10.1016/j.compstruct.2011.04.014
[12]	ALIBEIGLOO A. Thermo elasticity solution of sandwich circular plate with functionally graded core using generalized differential quadrature method[J]. Composite Structures, 2016, 136: 229-240. doi: 10.1016/j.compstruct.2015.10.012
[13]	MAHI A, BEDIA E A A, TOUNSI A. A new hyperbolic shear deformation theory for bending and free vibration analysis of isotropic, functionally graded, sandwich and laminated composite plates[J]. Applied Mathematical Modelling, 2015, 39(9): 2489-2508. doi: 10.1016/j.apm.2014.10.045
[14]	LI D, DENG Z, XIAO H, et al. Bending analysis of sandwich plates with different face sheet materials and functionally graded soft core[J]. Thin-Walled Structures, 2018, 122: 8-16. doi: 10.1016/j.tws.2017.09.033
[15]	VAN DO V N, LEE C H. Numerical investigation on post-buckling behavior of FGM sandwich plates subjected to in-plane mechanical compression[J]. Ocean Engineering, 2018, 170: 20-42. doi: 10.1016/j.oceaneng.2018.10.007
[16]	ZHAO W. Nonlinear axisymmetric thermomechanical response of FGM circular plates[J]. Journal of the Brazilian Society of Mechanical Sciences and Engineering, 2020, 42(7): 3-10.
[17]	HUANG C L, SANDMAN B E. Large amplitude vibrations of a rigidly clamped circular plate[J]. International Journal of Non-Linear Mechanics, 1971, 6(4): 451-468. doi: 10.1016/0020-7462(71)90043-6
[18]	LI S R, ZHANG J H, ZHAO Y G. Nonlinear thermomechanical post-buckling of circular FGM plate with geometric imperfection[J]. Thin-Walled Structures, 2007, 45(5): 528-536. doi: 10.1016/j.tws.2007.04.002
[19]	VAN DO V N, LEE C H. Nonlinear thermal buckling analyses of functionally graded circular plates using higher-order shear deformation theory with a new transverse shear function and an enhanced mesh-free method[J]. Acta Mechanica, 2018, 229: 3787-3811. doi: 10.1007/s00707-018-2190-7
[20]	REDDY J N, WANG C M, KITIPORNCHAI S. Axisymmetric bending of functionally graded circular and annular plates[J]. European Journal of Mechanics A: Solids, 1999, 18(2): 185-199. doi: 10.1016/S0997-7538(99)80011-4
[21]	王雪, 赵伟东. 功能梯度梁在热-机械荷载作用下的几何非线性分析[J]. 应用数学和力学, 2019, 40(5): 508-517. doi: 10.21656/1000-0887.390201 WANG Xue, ZHAO Weidong. Geometrically nonlinear analysis of functionally graded beam under thermomechanical loading[J]. Applied Mathematics and Mechanics, 2019, 40(5): 508-517. (in Chinese) doi: 10.21656/1000-0887.390201
[22]	李世荣, 苏厚德, 程昌钧. 热环境中粘贴压电层功能梯度材料梁的自由振动[J]. 应用数学和力学, 2009, 30(8): 907-918. doi: 10.3879/j.issn.1000-0887.2009.08.003 LI Shirong, SU Houde, CHENG Changjun. Free vibration of functionally graded material beams with surface-bonded piezoelectric layers in thermal environment[J]. Applied Mathematics and Mechanics, 2009, 30(8): 907-918. (in Chinese) doi: 10.3879/j.issn.1000-0887.2009.08.003
[23]	LIS R, BATRA R C, MA L S. Vibration of thermally post-buckled orthotropic circular plate[J]. Journal of Thermal Stresses, 2007, 30(1): 43-57. doi: 10.1080/01495730600897161
[24]	NAJAFIZADEH M M, HEDAYATI B. Refined theory for thermoelastic stability of functionally graded circular plates[J]. Journal of Thermal Stresses, 2004, 27(9): 857-880. doi: 10.1080/01495730490486532