Lattice Boltzmann Study on the Motion of Dual Droplets in Microchannels With Contact Angle Hysteresis
-
摘要:
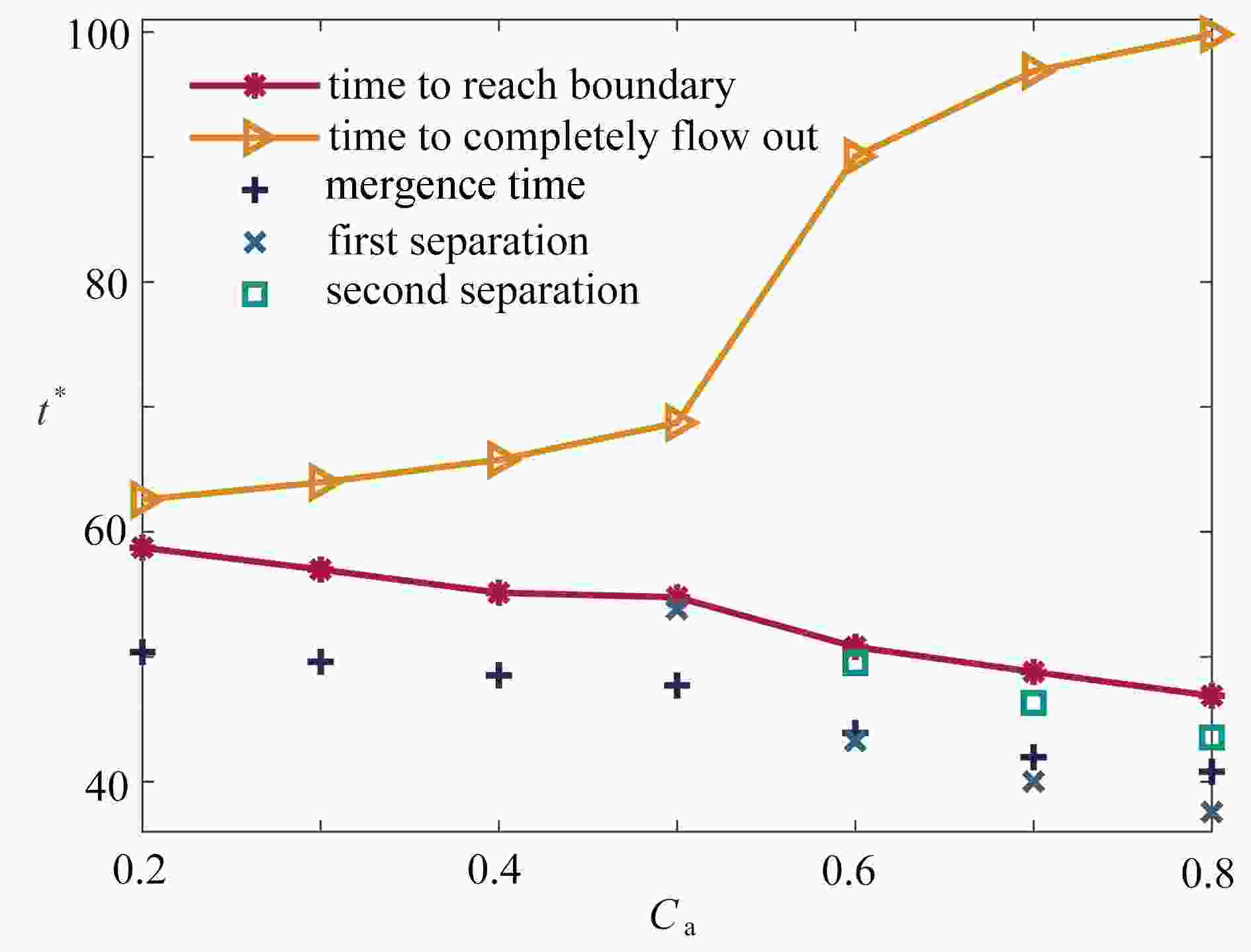
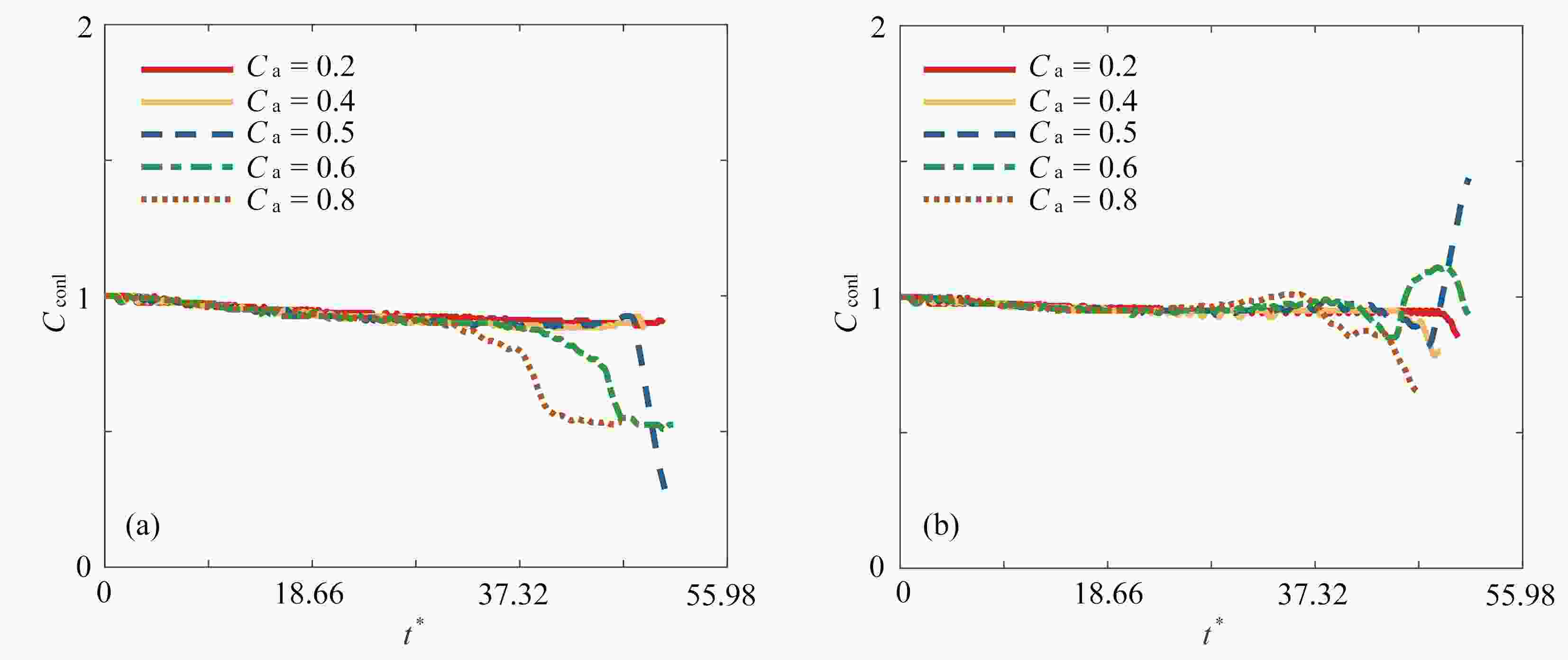
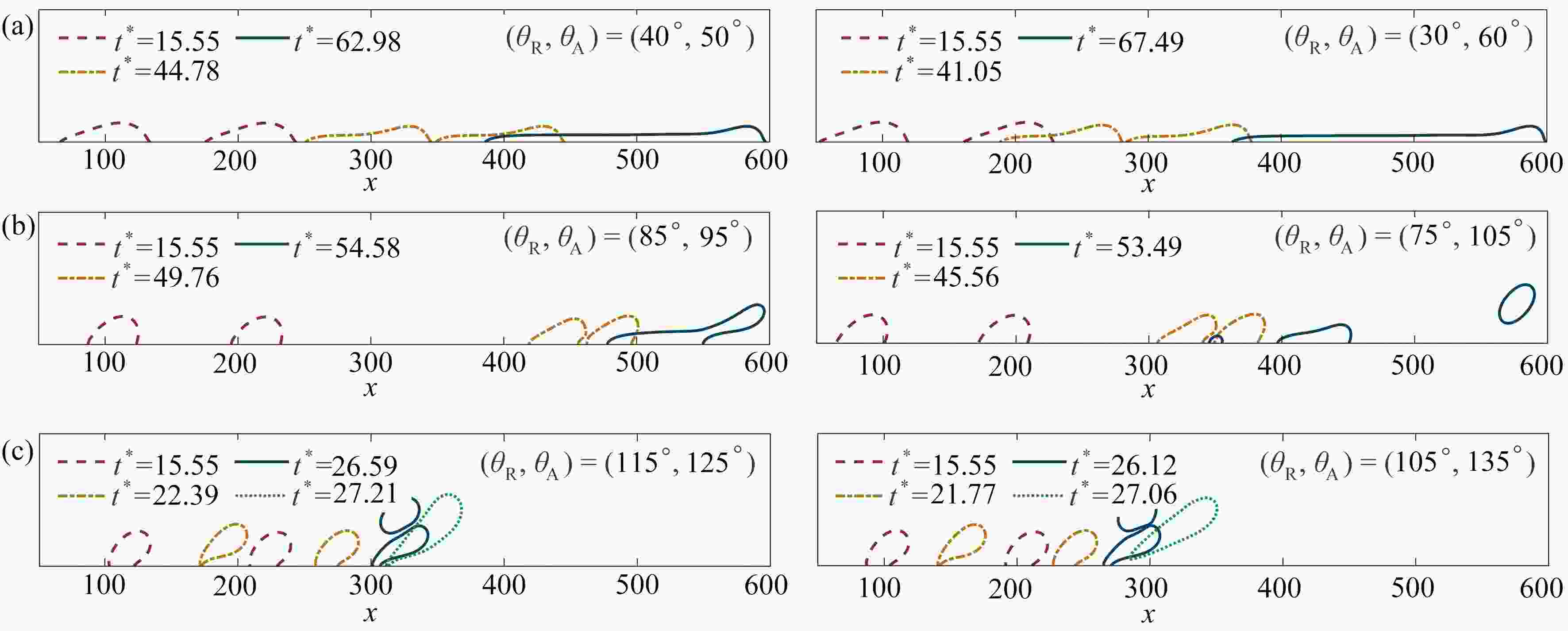
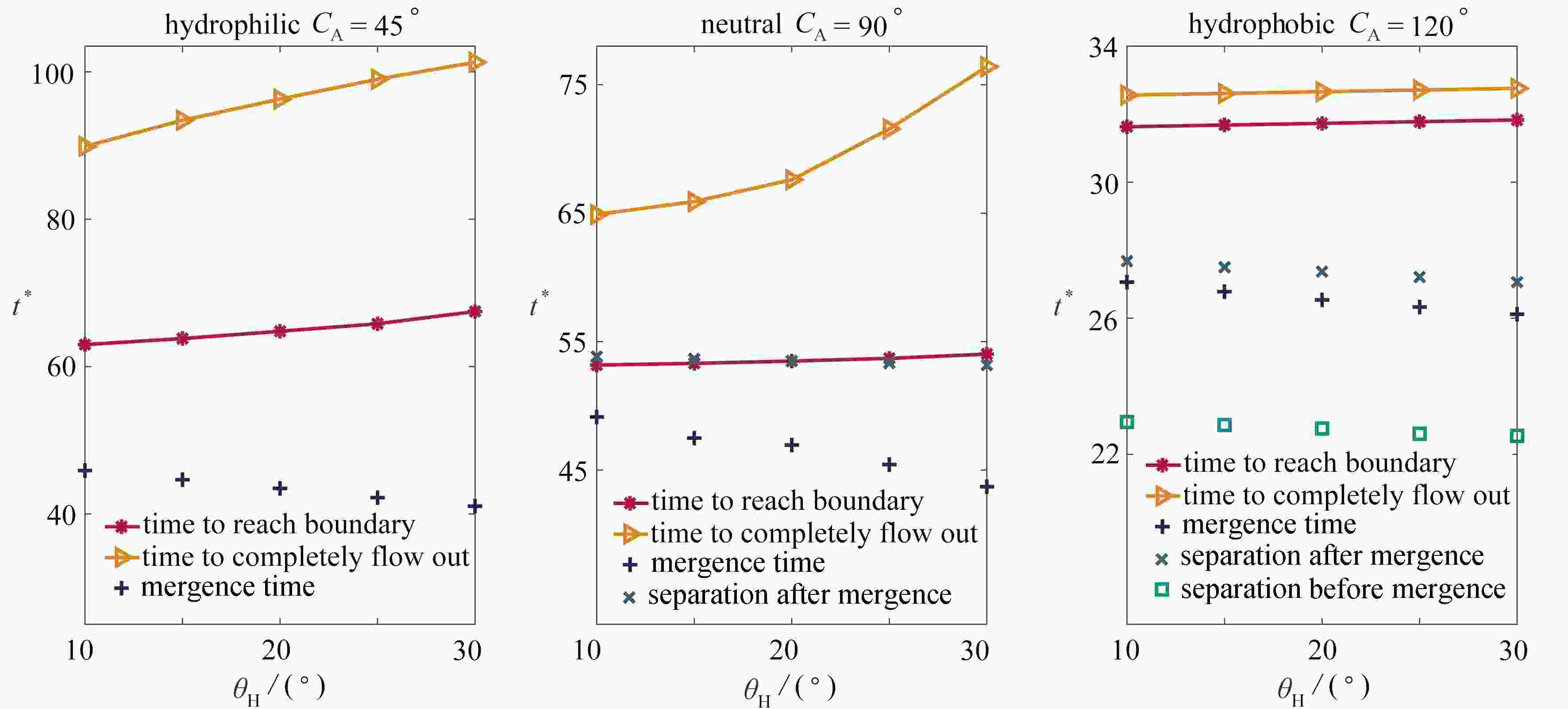
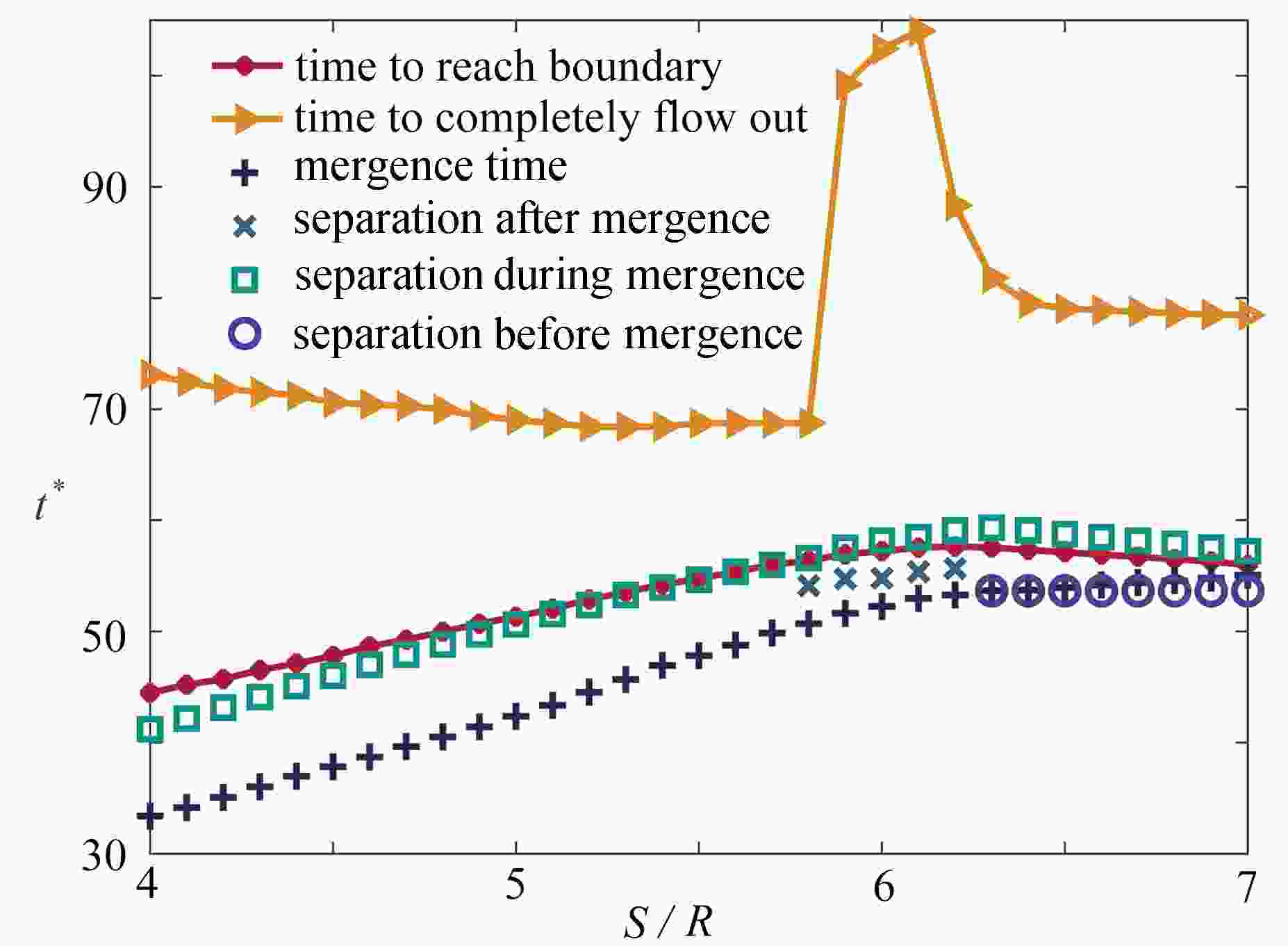
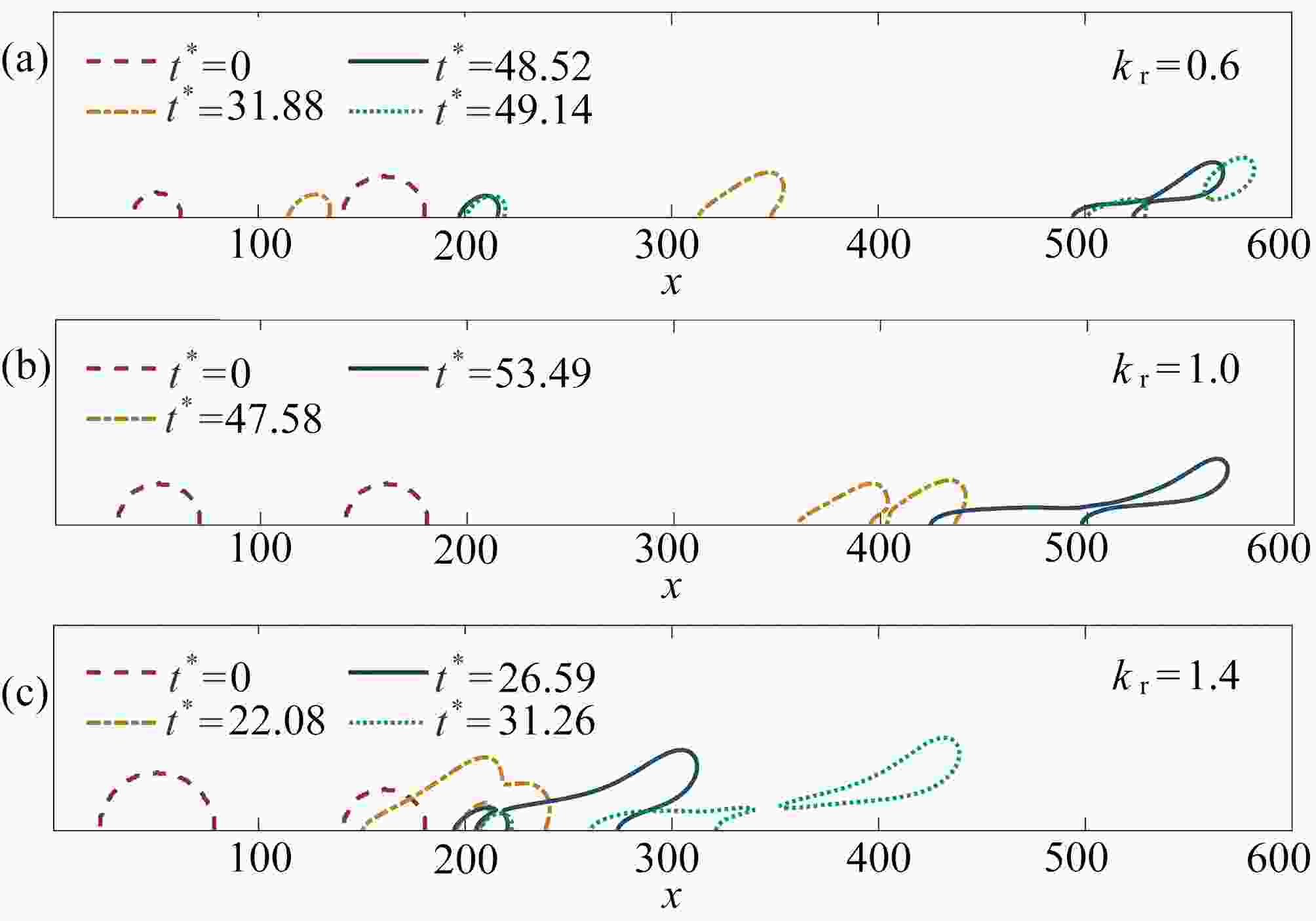
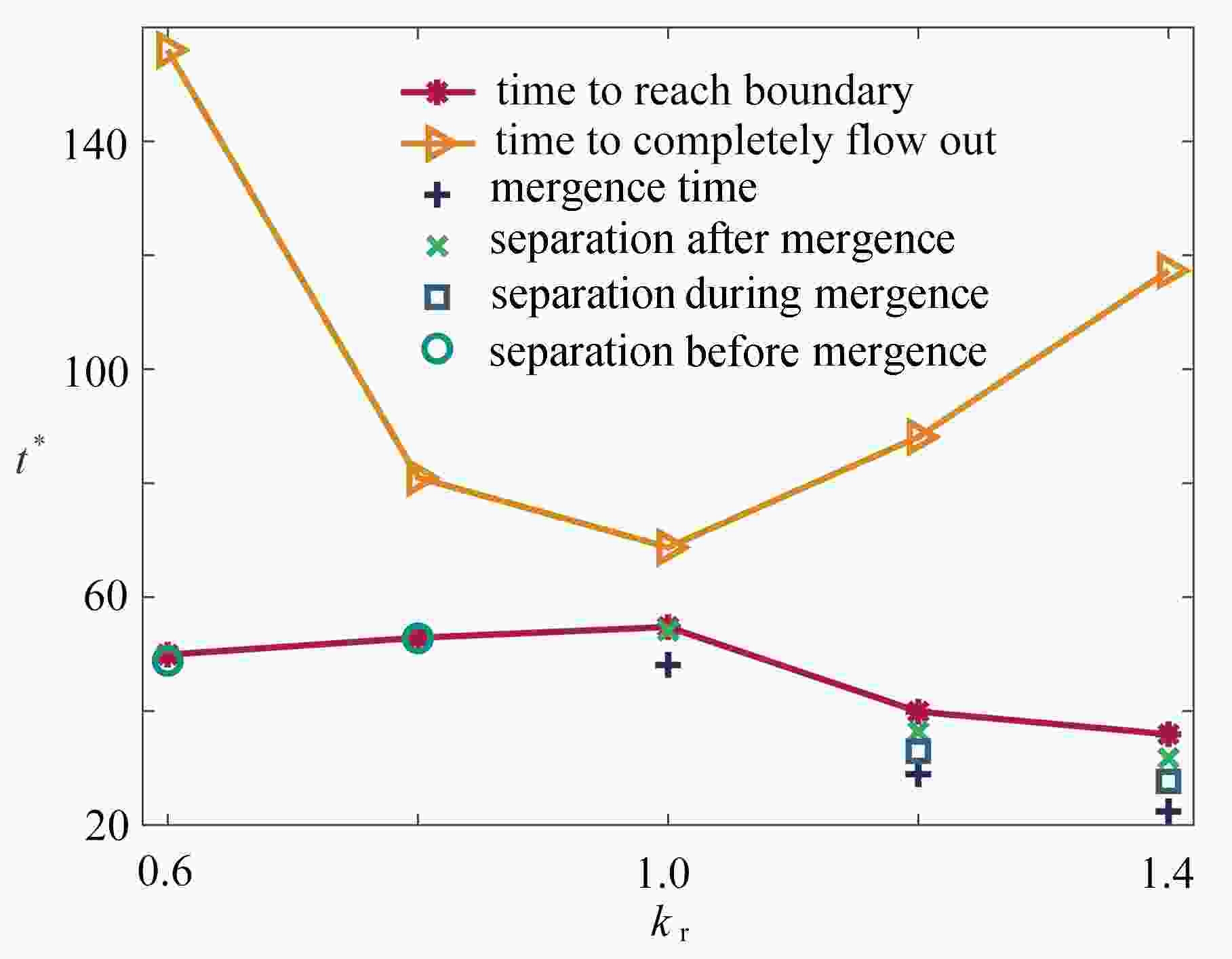
接触角滞后表现为流体在非理想固体表面上运动时前进接触角和后退接触角不同，是两相流体在润湿表面上流动的重要现象。该文采用改进的伪势格子Boltzmann (LB)多组分模型，并与几何润湿边界条件相结合，研究了两个液滴在具有接触角滞后性微通道表面上的运动行为，主要研究了通道内特征数、通道表面性质以及液滴初始参数的影响。研究结果表明：毛细数的增大有助于液滴的移动，然而并不利于液滴的排出，且毛细数的增加对上游液滴的影响大于其对下游液滴的影响；另一方面，接触角滞后性窗口越大，液滴运动和形变更迟缓，但形变程度更明显，两液滴更早地发生合并，但更晚地排出管道；液滴间距的增加使液滴的运动行为在不同阶段表现为不同的模式，但都导致通道中残留小液滴，使得液滴排出通道的时间增加。研究结果还表明：上游液滴和下游液滴的相对尺寸差距越大，越不利于液滴排出管道。
-
关键词:
- 接触角滞后性 /
- 格子Boltzmann方法 /
- 液滴相互作用 /
- 剪切流
Abstract:The contact angle hysteresis is defined as the difference between the advancing and receding contact angles, and is an important phenomenon in the two-phase flow on the wet surface. An improved pseudo-potential lattice Boltzmann (LB) multiphase flow model, combined with geometric wetting boundary condition, was employed to study the motion behavior of dual droplets in microchannels with contact angle hysteresis. The effects of the capillary number, the wettability, the contact angle hysteresis window width, the initial distance between the two droplets and the relative size of the droplets on the dynamic behavior of the droplets in the microchannel, were investigated. The research results show that, the increase of the capillary number is conducive to the movement of droplets, but not conducive to the discharge of droplets from the microchannel, and the influence of the capillary number on the upstream droplet is greater than that on the downstream droplet. On the other hand, the larger the contact angle hysteresis window is, the slower the droplet motion and deformation will be, but the more obvious the deformation will be, and the earlier the two droplets will merge but the later they will discharge from the microchannel. In addition, with the increase of the initial distance between the two droplets, the droplet motion mode will differ among different stages, but the duration of the droplet discharge will extend. Correspondingly, the larger the relative size difference between upstream and downstream droplets is, the more difficultly the droplets will discharge from the microchannel.
-
Key words:
- contact angle hysteresis /
- lattice Boltzmann method /
- droplet interaction /
- shear flow
-
图 4 微通道表面上液滴示意图
Figure 4. A schematic diagram of a droplet on the lower surface of the microchannel
图 5 双液滴在不同时刻的瞬时流型：(a)
$ C_{\rm{a}}=0.2 $ ；(b)$ C_{\rm{a}}=0.5 $ ；(c)$ C_{\rm{a}}=0.8 $ 注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同。
Figure 5. Instantaneous flow patterns of two droplets at different moments: (a)
$ C_{\rm{a}}=0.2 $ ; (b)$ C_{\rm{a}}=0.5 $ ; (c)$ C_{\rm{a}}=0.8 $ 
图 6 不同毛细数下液滴状态变化时间
Figure 6. Droplet state change time under different capillary numbers
图 7 不同毛细数下的上、下游液滴液-壁接触长度随时间的变化：(a) 上游液滴；(b) 下游液滴
Figure 7. Droplet-wall contact lengths under different capillary numbers: (a) upstream droplets; (b) downstream droplets
图 8 不同滞后性窗口下两液滴的瞬时流型：(a) CA= 45°；(b) CA= 90°；(c) CA= 120°
Figure 8. Instantaneous flow patterns of two droplets under different hysteresis windows: (a) CA= 45°; (b) CA= 90°; (c) CA= 120°
图 9 在不同滞后性窗口下的上、下游液滴液-壁接触长度随时间的变化：(a)上游液滴；(b)下游液滴
Figure 9. Variations of liquid-wall contact lengths with time under different hysteresis windows: (a) upstream droplets; (b) downstream droplets
图 10 不同滞后性窗口下液滴形态变化时刻
Figure 10. Droplet state change moments under different hysteresis windows
图 11 两液滴在
$ S=4R $ ，$6R$ ，$7R$ 下的瞬时流型Figure 11. Instantaneous flow patterns of two droplets for
$S=4R, 6R,7R$ 
图 12 不同间距下液滴形态变化时刻
Figure 12. Droplet state change moments under different drops distance
图 13 两液滴在
$ k_{\rm{r}}=0.6 $ ，$1.0$ ，$1.4$ 下的瞬时流型Figure 13. Instantaneous flow patterns of two droplets for
$ k_{\rm{r}}=0.6,1.0,1.4 $ 
图 14 不同相对大小下的上、下游液滴接触长度随时间的变化：(a) 上游液滴；(b)下游液滴
Figure 14. Variations of liquid-wall contact lengths with time under different droplet relative sizes: (a) upstream droplets; (b) downstream droplets
图 15 不同初始液滴相对大小下液滴状态变化时刻
Figure 15. Droplet state change moments under different initial droplet relative sizes
表 1 网格无关性
Table 1. Grid independence
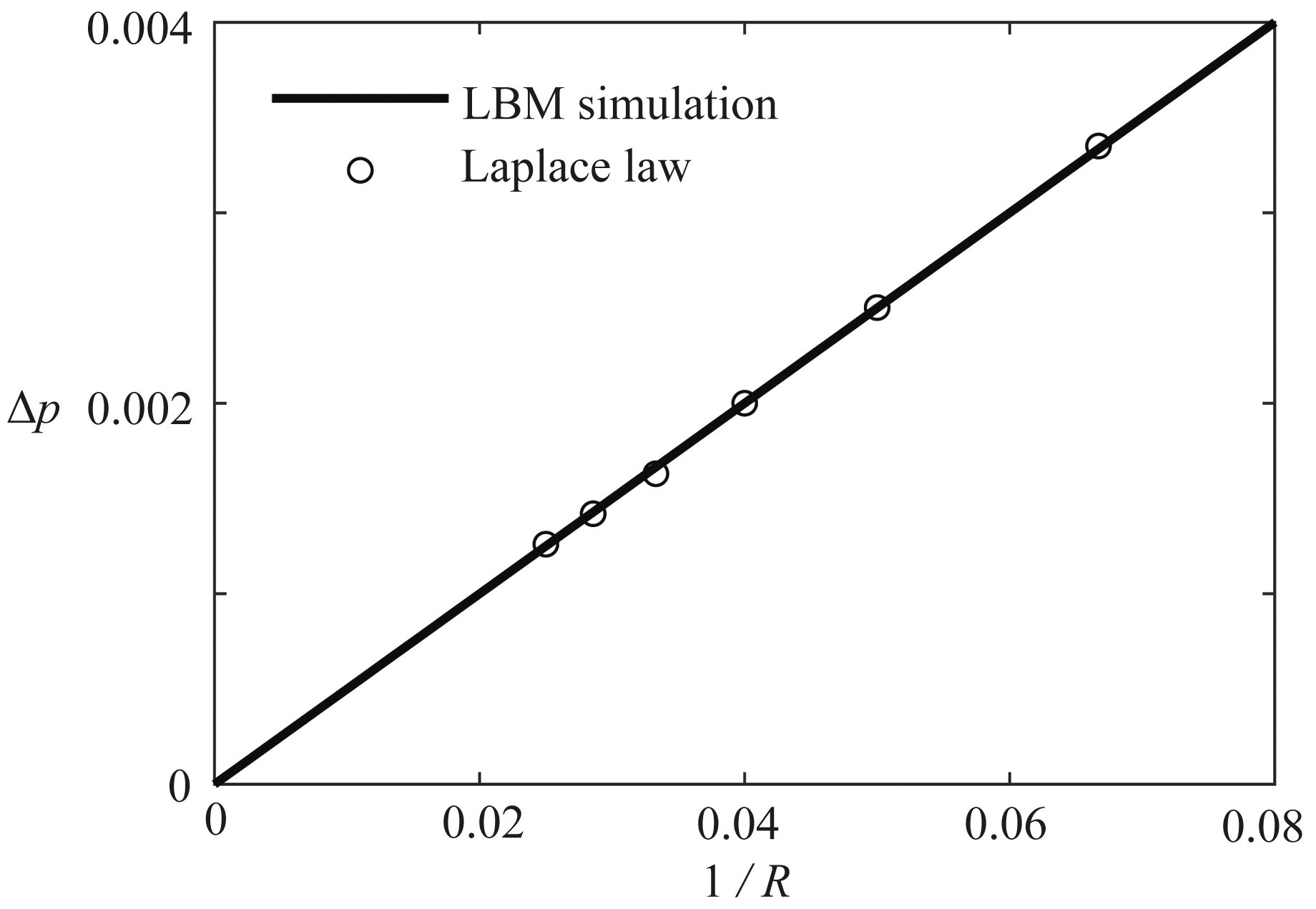
$ L\times 2H $ $ D $ $ t_{\rm{a}} $ $ t_{\rm{b}} $ $ 300\times100 $ 8 53.5321 67.7234 $ 600\times200 $ 6 54.4856 68.5080 $ 1\;200\times400 $ 6 54.4832 68.5093 
下载: 导出CSV
-
[1] GUPTA A K, BASU S. Deformation of an oil droplet on a solid substrate in simple shear flow[J]. Chemical Engineering Science, 2008, 63(22): 5496-5502. [2] LIU Y, FAN J W, LIU L L, et al. Numerical simulation of residual oil flooded by polymer solution in microchannels[J]. Geofluids, 2018, 2018: 8947839. [3] QIN C Z, RENSINK D, HASSANIZADEH S M, et al. Direct simulation of liquid water dynamics in the gas channel of a polymer electrolyte fuel cell[J]. Journal of the Electrochemical Society, 2012, 159(4): B434-B443. [4] ISMAIL M S, HUGHES K J, INGHAM D B, et al. Effects of anisotropic permeability and electrical conductivity of gas diffusion layers on the performance of proton exchange membrane fuel cells[J]. Applied Energy, 2012, 95: 50-63. [5] YANG X G, ZHANG F Y, LUBAWY A L, et al. Visualization of liquid water transport in a PEFC[J]. Journal of Power Sources, 2004, 7(11): A408-A411. [6] CHO S C, WANG Y, CHEN K S. Droplet dynamics in a polymer electrolyte fuel cell gas flow channel: forces, deformation and detachment Ⅱ: comparisons of analytical solution with numerical and experimental results[J]. Journal of Power Sources, 2012, 210: 191-197. [7] ZHAN Z G, WANG C, FU W G, et al. Visualization of water transport in a transparent PEMFC[J]. International Journal of Hydrogen Energy, 2012, 37(1): 1094-1105. [8] SEEVARATNAM G K, DING H, MICHEL O, et al. Laminar flow deformation of a droplet adhering to a wall in a channel[J]. Chemical Engineering Science, 2010, 65(15): 4523-4534. [9] SCHLEIZER A D, BONNECAZE R T. Displacement of a two-dimensional immiscible droplet adhering to a wall in shear and pressure-driven flows[J]. Journal of Fluid Mechanics, 1999, 383: 29-54. [10] HAO L, CHENG P. Lattice Boltzmann simulations of liquid droplet dynamic behavior on a hydrophobic surface of a gas flow channel[J]. Journal of Power Sources, 2009, 190: 435-446. [11] ZHU X, SUI P C, DJILALI N. Three-dimensional numerical simulations of water droplet dynamics in a PEMFC gas channel[J]. Journal of Power Sources, 2012, 181(1): 101-115. [12] MONDAL B, JIAO K, LI X. Three-dimensional simulation of water droplet movement in PEM fuel cell flow channels with hydrophilic surfaces[J]. International Journal of Energy Research, 2011, 35(13): 1200-1212. [13] RAMAN K A, BIRGERSSON E, SUI Y, et al. Electrically induced droplet ejection dynamics under shear flow[J]. Physics of Fluids, 2020, 32(3): 032103. [14] CRISTINI V, TAN Y C. Theory and numerical simulation of droplet dynamics in complex flows: a review[J]. Lab on a Chip, 2004, 4(4): 257-264. [15] ZHANG K X, LI Z, MAXEY M, et al. Self-cleaning of hydrophobic rough surfaces by coalescence-induced wetting transition[J]. Langmuir, 2019, 35(6): 2431-2442. [16] HAN B, YU J, MENG H. Lattice Boltzmann simulations of liquid droplets development and interaction in a gas channel of a proton exchange membrane fuel cell[J]. Journal of Power Sources, 2012, 202: 175-183. [17] 李家宇, 曾忠, 乔龙. 相场方法模拟液滴的动态润湿行为[J]. 应用数学和力学, 2019, 40(9): 957-967LI Jiayu, ZENG Zhong, QIAO Long. Numerical simulation of droplets’ dynamic wetting process with the phase field method[J]. Applied Mathematics and Mechanics, 2019, 40(9): 957-967.(in Chinese) [18] ERAL H B, T MANNETJE D, OH J M. Contact angle hysteresis: a review of fundamentals and applications[J]. Colloid and Polymer Science, 2013, 291(2): 247-260. [19] QIAN B A, BREUER K S. The motion, stability and breakup of a stretching liquid bridge with a receding contact line[J]. Journal of Fluid Mechanics, 2011, 666: 554-572. [20] DAI Q, HUANG W, WANG X. Contact angle hysteresis effect on the thermocapillary migration of liquid droplets[J]. Journal of Colloid and Interface Science, 2018, 515: 32-38. [21] YANG J P, MA X, FEI L L, et al. Effects of hysteresis window on contact angle hysteresis behaviour at large Bond number[J]. Journal of Colloid and Interface Science, 2020, 556(3): 327-337. [22] FANG C, HIDROVO C, WANG F M, et al. 3-D numerical simulation of contact angle hysteresis for microscale two phase flow[J]. International Journal of Multiphase Flow, 2008, 34(7): 690-705. [23] DUSSAN E B. On the ability of drops to stick to surfaces of solids Ⅲ: the influences of the motion of the surrounding fluid on dislodging drops[J]. Journal of Fluid Mechanics, 1987, 174: 381-397. [24] 柴振华, 郭照立, 施保昌. 利用多松弛格子Boltzmann 方法预测多孔介质的渗透率[J]. 工程热物理学报, 2010, 31(1): 107-109CHAI Zhenhua, GUO Zhaoli, SHI Baochang. Prediction of permeability in porous media with multi-relaxation-time lattice Boltzmann method[J]. Journal of Engineering Thermophysics, 2010, 31(1): 107-109.(in Chinese) [25] 谢驰宇, 张建影, 王沫然. 液滴在固体平表面上均匀蒸发过程的格子Boltzmann模拟[J]. 应用数学和力学, 2014, 35(3): 247-253XIE Chiyu, ZHANG Jianying, WANG Moran. Lattice Boltzmann simulation of droplet evaporation on flat solid surface[J]. Applied Mathematics and Mechanics, 2014, 35(3): 247-253.(in Chinese) [26] 张贝豪, 郑林. 倾斜多孔介质方腔内纳米流体自然对流的格子Boltzmann方法模拟[J]. 物理学报, 2020, 69(16): 164401 doi: 10.7498/aps.69.20200308ZHANG Beihao, ZHENG Lin. Numerical simulation of natural convection of nanofluids in an inclined square porous enclosure by lattice Boltzmann method[J]. Acta Physica Sinica, 2020, 69(16): 164401.(in Chinese) doi: 10.7498/aps.69.20200308 [27] BA Y, LIU H H, SUN J J, et al. Color-gradient lattice Boltzmann model for simulating droplet motion with contact-angle hysteresis[J]. Physical Review E, 2013, 88: 043306. [28] 许友生, 刘慈群, 俞慧丹. 多孔介质中两相驱离的格子Boltzmann模型新研究[J]. 应用数学和力学, 2002, 23(4): 353-358XU Yousheng, LIU Ciqun, YU Huidan. New studying of lattice Boltzmann method for two phase driven in porous media[J]. Applied Mathematics and Mechanics, 2002, 23(4): 353-358.(in Chinese) [29] SWIFT M R, ORLANDINI E, OSBORN W R, et al. Lattice Boltzmann simulations of liquid-gas and binary fluid systems[J]. Physical Review E, 1996, 54(5): 5041-5052. [30] LI Q X, CHAI Z H, SHI B C, et al. Deformation and breakup of a liquid droplet past a solid circular cylinder: a lattice Boltzmann study[J]. Physical Review E, 2014, 90: 043015. [31] 梁佳, 高明, 陈露, 等. 基于格子Boltzmann方法的液滴撞击具有不同润湿性孔板的研究[J]. 应用数学和力学, 2022, 43(1): 63-76LIANG Jia, GAO Ming, CHEN Lu, et al. Study on droplets impacting on orifice plates with different wettabilities based on the lattice Boltzmann method[J]. Applied Mathematics and Mechanics, 2022, 43(1): 63-76.(in Chinese) [32] EBADI A, HOSSEINALIPOUR S M. The collision of immiscible droplets in three-phase liquid systems: a numerical study using phase-field lattice Boltzmann method[J]. Chemical Engineering Research and Design, 2022, 178: 289-314. [33] YUE P T. Thermodynamically consistent phase-field modelling of contact angle hysteresis[J]. Journal of Fluid Mechanics, 2020, 899: A15. [34] PORTER M L, COON E T, KANG Q J, et al. Multicomponent interparticle potential lattice Boltzmann model for fluids with large viscosity ratios[J]. Physical Review E, 2012, 86(3): 036701. [35] SHAN X W. Analysis and reduction of the spurious current in a class of multiphase lattice Boltzmann models[J]. Physical Review E, 2006, 73(4): 047701. [36] KANG Q, ZHANG D, CHEN S. Displacement of a two-dimensional immiscible droplet in a channel[J]. Physics of Fluids, 2002, 14(9): 3203-3214. [37] DING H, SPELT P D M. Wetting condition in diffuse interface simulations of contact line motion[J]. Physical Review E, 2007, 75(4): 046708. [38] LIU H H, JU Y P, WANG N N, et al. Lattice Boltzmann modeling of contact angle and its hysteresis in two-phase flow with large viscosity difference[J]. Physical Review E, 2015, 92(3): 033306. [39] LADD A J C. Numerical simulations of particulate suspensions via a discretized Boltzmann equation part 1: theoretical foundation[J]. Journal of Fluid Mechanics, 1994, 271: 285-309. [40] WANG C, SHEN C Q, WU S C, et al. Hydrodynamic binary coalescence of droplets under air flow in a hydrophobic microchannel[J]. Chinese Physics B, 2019, 28(2): 024702. [41] ZHANG F Y, YANG X G, WANG C Y. Liquid water removal from a polymer electrolyte fuel cell[J]. Journal of the Electrochemical Society, 2005, 153(2): A225. [42] WU J, HUANG J J. Dynamic behaviors of liquid droplets on a gas diffusion layer surface: hybrid lattice Boltzmann investigation[J]. Journal of Applied Physics, 2015, 118(4): 044902. -
计量
- 文章访问数: 551
- HTML全文浏览量: 204
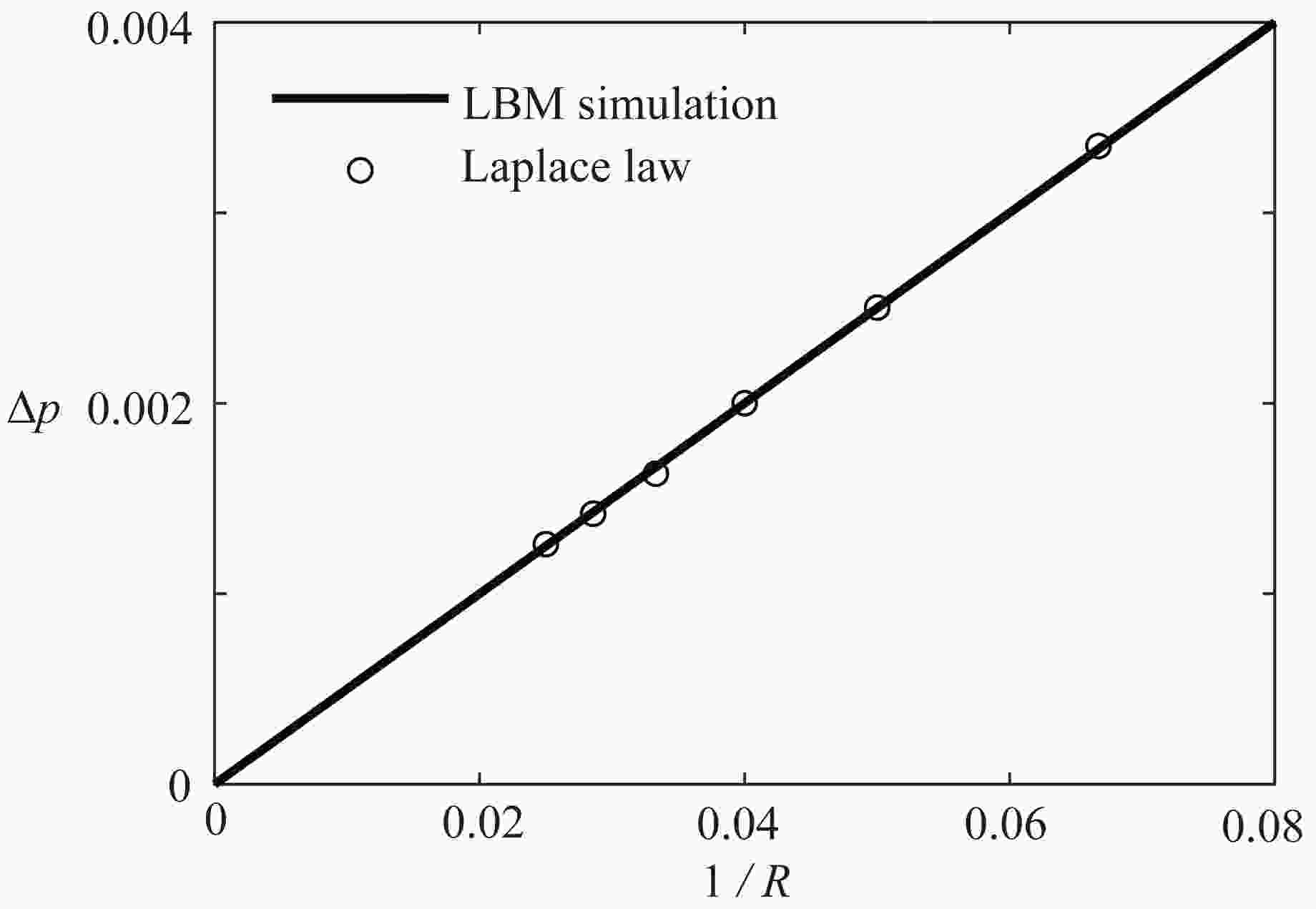
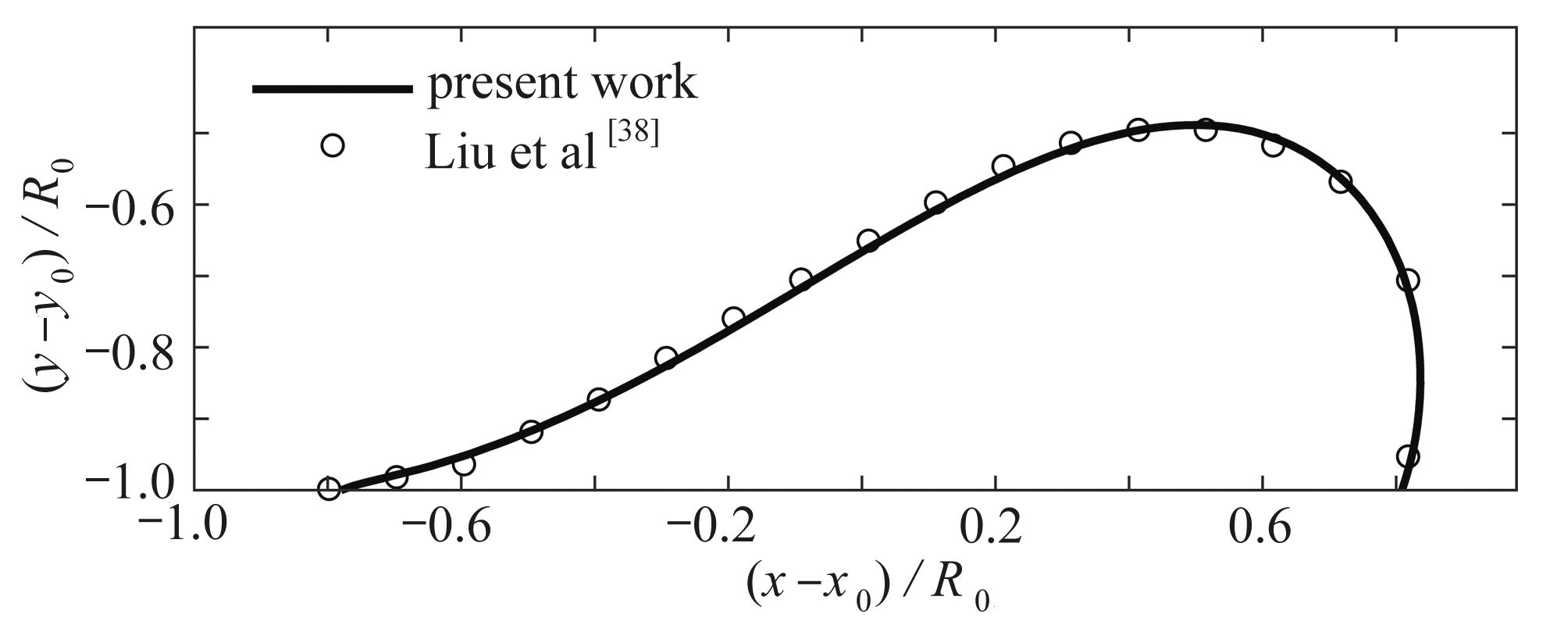
- PDF下载量: 61
- 被引次数: 0
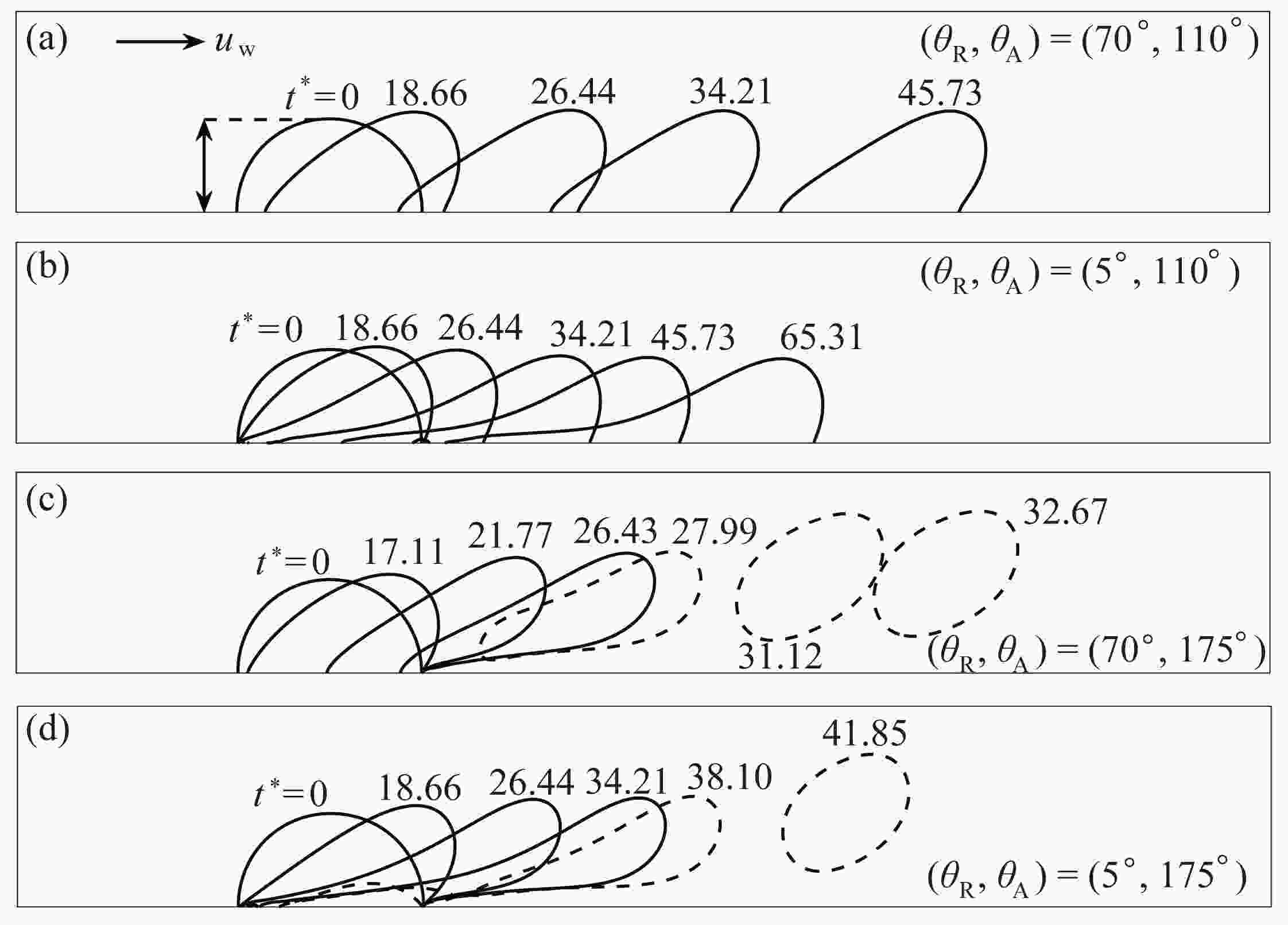
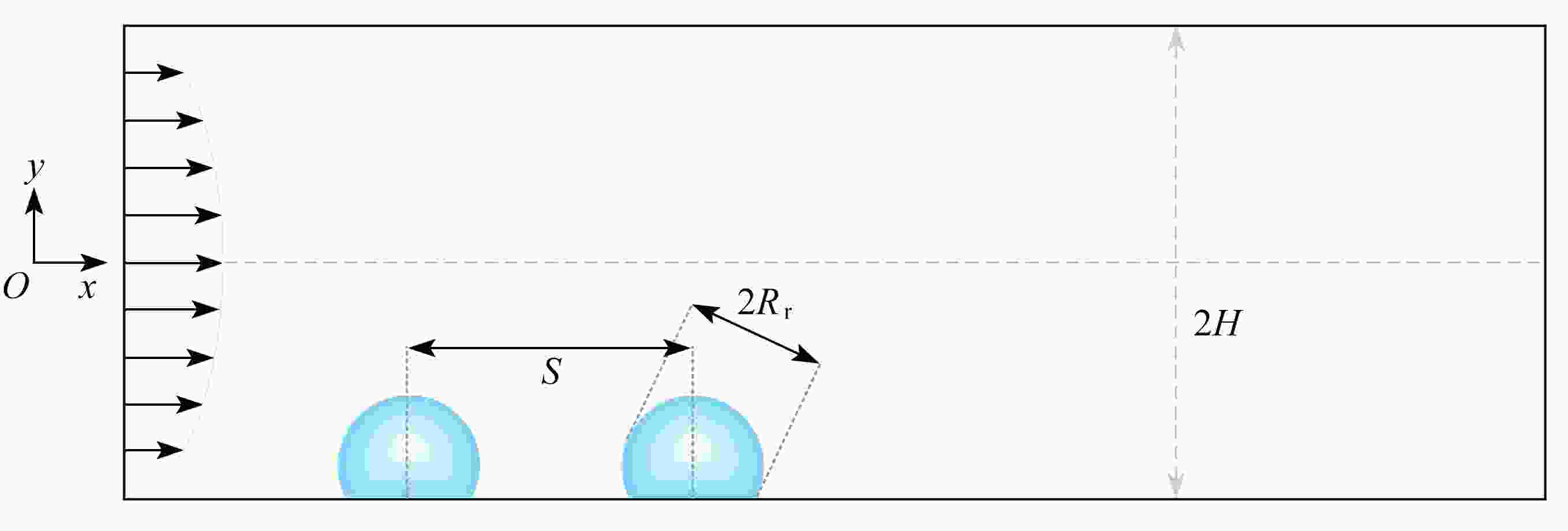
渝公网安备50010802005915号