Study on Collision Characteristics of Rotating Rod Strings in Annulus Fluid With Wellbores Based on Nested Grids
-
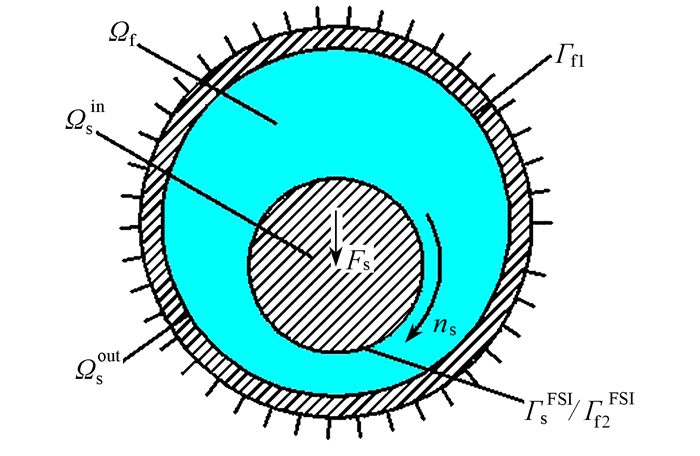
摘要: 针对浸没在流体中杆管柱间相互接触问题，基于嵌套网格技术，该文建立了环空流体内旋转杆柱与井筒间碰撞的数值求解方法. 将环空流体域分为相互嵌套的子区域：背景网格和组件网格，推导了各嵌套区域流场边界传递信息的插值计算公式，采用分域方法对环空流体域与杆柱固体域耦合进行求解. 通过静止流体中球形颗粒与壁面正、斜碰撞实验对比，验证该文数值方法的正确性. 研究了不同流体黏度、杆柱旋转速度条件下杆柱与井筒的碰撞特性，结果表明：1)杆柱与井筒碰撞的碰撞力、速度随黏度增大而降低，即杆柱与井筒碰撞的剧烈程度与流体黏度负相关；2)随着杆柱旋转速度增大，杆柱与井筒的碰撞力、速度也增大，即杆柱与井筒碰撞的剧烈程度与转速正相关.Abstract: To solve the contact problem between the rod string immersed in annulus fluid and the wellbore, a numerical solution method for the collision was established based on the nested grid technology. The annulus fluid domain was divided into 2 sub-domains: the background grid and the component grid. Then, the interpolation calculation formula for the flow field boundary transferred information in each nested domain was derived and the coupling between the annulus fluid domain and the rod solid domain was solved with the subdomain-based method. Through comparison of the frontal and oblique collision experiments on spherical particles and wall surface in stationary fluid, the correctness of the proposed numerical method was verified. The results show that, the force and velocity of the collision between the rod string and the wellbore decrease with the fluid viscosity, i.e., the collision intensity is negatively correlated with the fluid viscosity. Moreover, the force and velocity of the collision between the rod string and the wellbore increase with the rotation speed of the rod, i.e., the collision intensity is positively correlated with the rotation speed.
-
Key words:
- nested grid /
- annulus fluid /
- rotating rod string /
- collision
-
图 3 挖洞和重叠最小化后的嵌套网格
注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.
Figure 3. The nested mesh with a hole and minimal overlap
图 5 供体单元尺寸大于受体单元尺寸
Figure 5. The donor element size larger than the acceptor element size
图 6 供体单元尺寸小于受体单元尺寸
Figure 6. The donor element size smaller than the acceptor element size
图 7 环空流体域嵌套网格计算
Figure 7. The flowchart for the calculation of the annular fluid domain nested grid
图 9 流体中球形颗粒自由沉降有限元模型
Figure 9. The finite element model of free settlement of spherical particles in fluid
图 10 垂向速度vp为-0.7 m·s-1时的速度云图
Figure 10. Velocity contours for vertical velocity vp=-0.7 m·s-1
图 12 流体中球形颗粒与壁面碰撞有限元模型
Figure 12. The finite element model of collision between spherical particles and wall in fluid
图 13 恢复系数e与Stokes数S的关系曲线
Figure 13. Relation curves between recovery coefficient e and Stokes number S
图 14 球形颗粒碰撞过程中高度、速度随时间的变化曲线
Figure 14. Variation curves of the displacement and the velocity with time during spherical particle collision
图 15 颗粒与壁面正碰撞涡量场随时间的变化
Figure 15. Contours diagram of vorticity fields changing with time during frontal collision between particles and wall
图 16 流体中球形颗粒与壁面斜碰撞有限元模型
Figure 16. The finite element model of oblique collision between spherical particles and wall surface in fluid
图 17 无量纲化入射角和反射角关系曲线
Figure 17. Relation curves of the dimensionless incident angle and the rebound angle
图 18 颗粒与壁面斜碰撞涡量场随时间的变化
Figure 18. Contours of vorticity fields changing with time during oblique collision between particles and wall
图 20 旋转杆柱与井筒碰撞力随时间的变化
Figure 20. Variations of impact forces between the rotating rod string and the wellbore with time
图 21 环空流体随杆柱运动的涡量
Figure 21. Vorticity contours of annulus fluid moving with the rod string
图 22 不同流体黏度下，旋转杆柱与井筒碰撞力随时间的变化
Figure 22. Variations of the impact force between the rotating rod string and the wellbore with time under different fluid viscosities
图 23 不同流体黏度下杆柱上与井筒第1次碰撞点的运动轨迹
Figure 23. Trajectories of the 1st collision point between the rod string and the wellbore under different fluid viscosities
图 24 不同流体黏度下杆柱中心点速度随时间的变化
Figure 24. Variations of the center point velocity of the rod string with time under different fluid viscosities
图 25 不同转速下杆柱与井筒间碰撞力随时间的变化
Figure 25. Variations of collision force between rod string and wellbore with time at different rotational speeds
图 26 不同转速下杆柱上与井筒第1次碰撞点的运动轨迹
Figure 26. Motion trajectories of the 1st collision point between the rod string and the wellbore at different rotational speeds
图 27 不同转速下中心点速度随时间的变化
Figure 27. Center point velocities changing with time at different rotational speeds
表 1 球形颗粒物理参数
Table 1. Physical parameters of spherical particles
case sphere diameter D/mm granular material granule density ρs/(kg·m-3) 7 3 steel 7 800 
下载: 导出CSV
表 2 球形颗粒与流体的物理参数
Table 2. Physical parameters of spherical particles and fluid
case sphere diameter D/mm fluid density ρf/(kg·m-3) fluid viscosity μf/(N·s/m2) Stokes number S ① 3 965 0.1 5 ② 6 965 0.1 26 ③ 3 953 0.02 60 ④ 4 953 0.02 104 ⑤ 3 935 0.01 149 ⑥ 3 920 0.005 369 ⑦ 5 920 0.005 760 ⑧ 5 998 0.001 3 480
下载: 导出CSV
表 3 球形颗粒与流体的物理参数
Table 3. Physical parameters of spherical particles and fluid
sphere diameter D/mm recovery coefficient edry coefficient of sliding friction μdry sphere density ρs/(kg·m-3) fluid density ρf/(kg·m-3) fluid viscosity μf/(N·s/m2) 12.7 0.97 0.11 7 800 998 0.001
下载: 导出CSV
表 4 旋转杆柱和流体的物理参数
Table 4. Physical parameters of rotating rod and fluid
elasticity modulus E/Pa Poisson’s ratio υ density of the rod string ρs/(kg·m-3) fluid density ρf/(kg·m-3) fluid viscosity μf/(N·s/m2) rotating speed of the rod string V/(rad·s-1) 2.4×1011 0.3 7 800 998 0.005 25.12
下载: 导出CSV
表 5 网格无关性验证
Table 5. Grid independence verification
solid domain fluid domain number of grid 1 122 2 109 3 234 47 375 68 900 105 396 137 991 first impact force/N 1.37 2.31 2.4 0.825 0.716 0.554 0.561
下载: 导出CSV
-
[1] FABIAN D, RAUL G, SRINIVASAN N. Arbitrary Lagrangian-Eulerian method for Navier-Stokes equations with moving boundaries[J]. Computer Methods in Applied Mechanics and Engineering, 2004, 193(45/47) : 4819-4836. http://www.captura.uchile.cl/bitstream/handle/2250/1874/Duarte,%20F.pdf?sequence=1 [2] 王奇, 朱寅鑫, 牛培行, 等. 柔性扑翼翼型的气动性能仿真分析[J]. 应用数学和力学, 2022, 43(5): 586-596. doi: 10.21656/1000-0887.430155WANG Qi, ZHU Yinxin, NIU Peixing, et al. Simulation of aerodynamic performances of flexible flapping wing airfoils[J]. Applied Mathematics and Mechanics, 2022, 43(5): 586-596. (in Chinese) doi: 10.21656/1000-0887.430155 [3] TOGASHI F, ITO Y, NAKAHASHI K. Extensions of overset unstructured grids to multiple bodies in contact[J]. Journal of Aircraft, 2006, 43(1): 52-57. doi: 10.2514/1.540 [4] MILLER S T, CAMPBELL R L, ELSWORTH C W, et al. An overset grid method for fluid-structure interaction[J]. World Journal of Mechanics, 2014, 4(7): 217-237. doi: 10.4236/wjm.2014.47023 [5] 李映坤. 多物理场耦合计算方法研究及其在双脉冲发动机中的应用[D]. 博士学位论文. 南京: 南京理工大学, 2017.LI Yingkun. A study of numerical method for multi-physics field coupling and application to dual pulse motor[D]. PhD Thesis. Nanjing: Nanjing University of Science and Technology, 2017. (in Chinese) [6] 倪同兵. 旋翼(尾桨)气动噪声的主/被动抑制方法及机理研究[D]. 博士学位论文. 南京: 南京航空航天大学, 2018.NI Tongbing. Active/passive noise suppression method and mechanism researches on aeroacoustic of rotor(tail-rotor)[D]. PhD Thesis. Nanjing: Nanjing University of Aeronautics and Astronautics, 2018. (in Chinese) [7] 徐广. 新型桨尖弹性旋翼气动特性的Navier-Stokes方程数值模拟[D]. 博士学位论文. 南京: 南京航空航天大学, 2010.XU Guang. Numerical simulation on aerodynamic characteristics of elastic rotors with new tip shape by N-S equations[D]. PhD Thesis. Nanjing: Nanjing University of Aeronautics and Astronautics, 2010. (in Chinese) [8] 史美娇, 徐慧东, 张建文. 双侧弹性约束悬臂梁的非光滑擦边动力学[J]. 应用数学和力学, 2022, 43(6): 619-630. doi: 10.21656/1000-0887.420177SHI Meijiao, XU Huidong, ZHANG Jianwen. Non-smooth grazing dynamics for cantilever beams with bilateral elastic constraints[J]. Applied Mathematics and Mechanics, 2022, 43(6): 619-630. (in Chinese) doi: 10.21656/1000-0887.420177 [9] MENTER F R. Two-equation eddy-viscosity turbulence models for engineering applications[J]. AIAA Journal, 1994, 32(8): 1598. doi: 10.2514/3.12149 [10] 刘云贺. 流体-固体动力耦合理论及水利工程应用[D]. 博士学位论文. 西安: 西安交通大学, 2001.LIU Yunhe. Fluid-solid dynamic coupling theory and its application in hydraulic engineering[D]. PhD Thesis. Xi'an: Xi'an Jiaotong University, 2001. (in Chinese) [11] 黄宇, 阎超, 王文, 等. 混合重叠网格插值方法的改进及应用[J]. 北京航空航天大学学报, 2017, 43(2): 285-292. https://www.cnki.com.cn/Article/CJFDTOTAL-BJHK201702010.htmHUANG Yu, YAN Chao, WANG Wen, et al. An improved interpolation method for hybrid overset grid and its application[J]. Journal of Beijing University of Aeronautics and Astronautics, 2017, 43(2): 285-292. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-BJHK201702010.htm [12] VENKATAKRISHNAN V. On the accuracy of limiters and convergence to steady state solutions: 93-0880[R]. AIAA Paper, 1993. [13] BAKER T J. Interpolation from a cloud of points[C]//Proceedings of the 12th International Meshing Roundtable. Santa Fe, 2003: 55-63. [14] 杨明, 刘巨保, 岳欠杯, 等. 基于浸入边界-有限元法的流固耦合碰撞数值模拟方法[J]. 应用数学和力学, 2019, 40(8): 880-892. doi: 10.21656/1000-0887.400053YANG Ming, LIU Jubao, YUE Qianbei, et al. Numerical simulation of fluid-solid coupling collision based on the finite element immersed boundary method[J]. Applied Mathematics and Mechanics, 2019, 40(8): 880-892. (in Chinese) doi: 10.21656/1000-0887.400053 [15] 杨明. 固体间碰撞与流体耦合的数值分析方法研究[D]. 博士学位论文. 大庆: 东北石油大学, 2019.YANG Ming. Study on numerical analysis method of solid to solid collision coupling with fluid[D]. PhD Thesis. Daqing: Northeast Petroleum University, 2019. (in Chinese) [16] 邓创华. 流固耦合弱耦合算法研究[D]. 硕士学位论文. 武汉: 华中科技大学, 2012.DENG Chuanghua. A thesis submitted in partial fulfillment of the requirements for the degree of master of applied sociology[D]. Master Thesis. Wuhan: Huazhong University of Science and Technology, 2012. (in Chinese) [17] GONDRET P, LANCE M, PETIT L. Bouncing motion of spherical particles in fluids[J]. Physics of Fluids, 2002, 14(2): 643. doi: 10.1063/1.1427920 [18] JOSEPH G G, HUNT M L. Oblique particle-wall collisions in a liquid[J]. Journal of Fluid Mechanics, 2004, 510: 71-93. -
计量
- 文章访问数: 484
- HTML全文浏览量: 178
- PDF下载量: 47
- 被引次数: 0
渝公网安备50010802005915号