Stokes Flow in Cylindrical Containers With Rotating Ends
-
摘要:
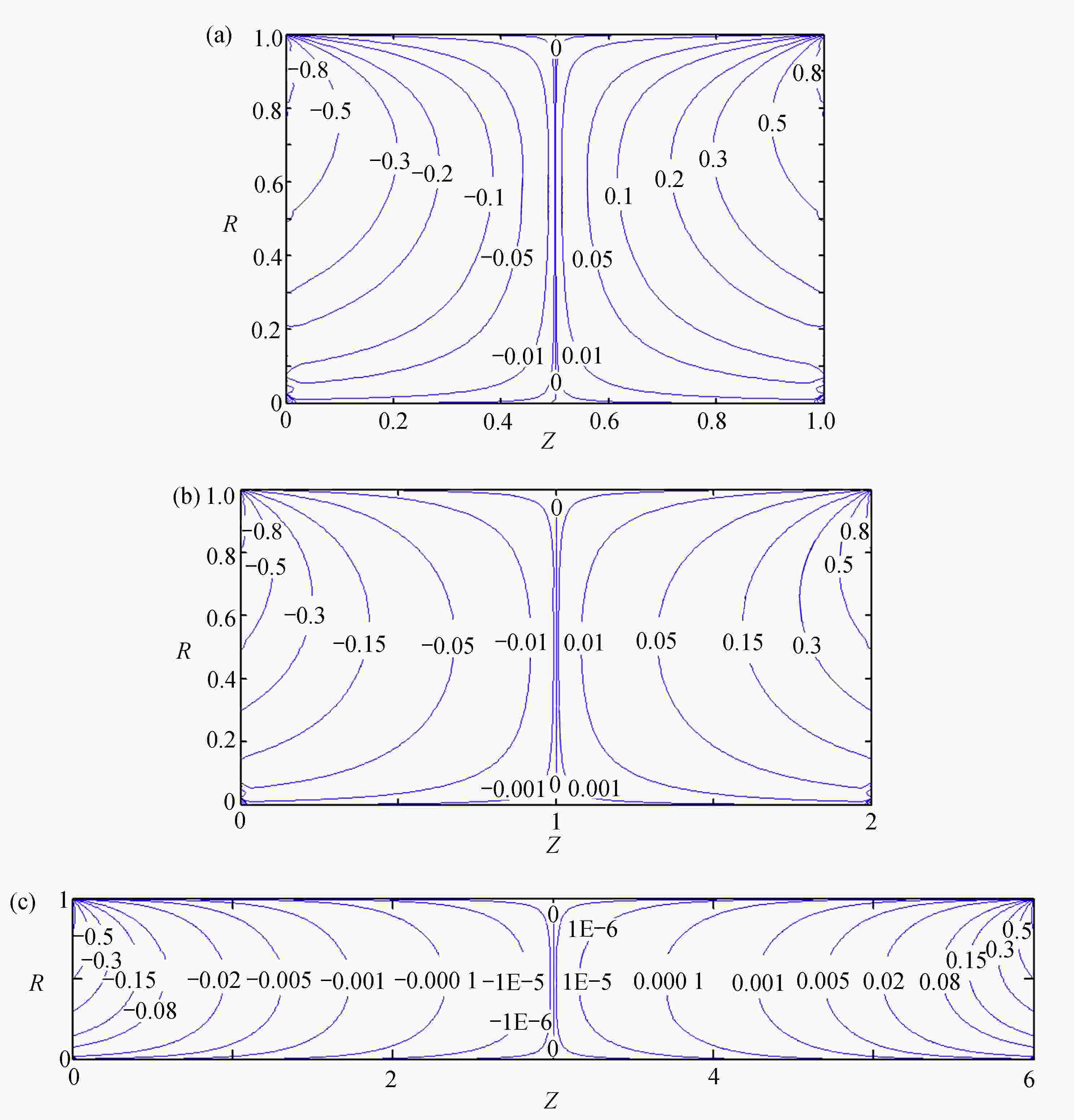
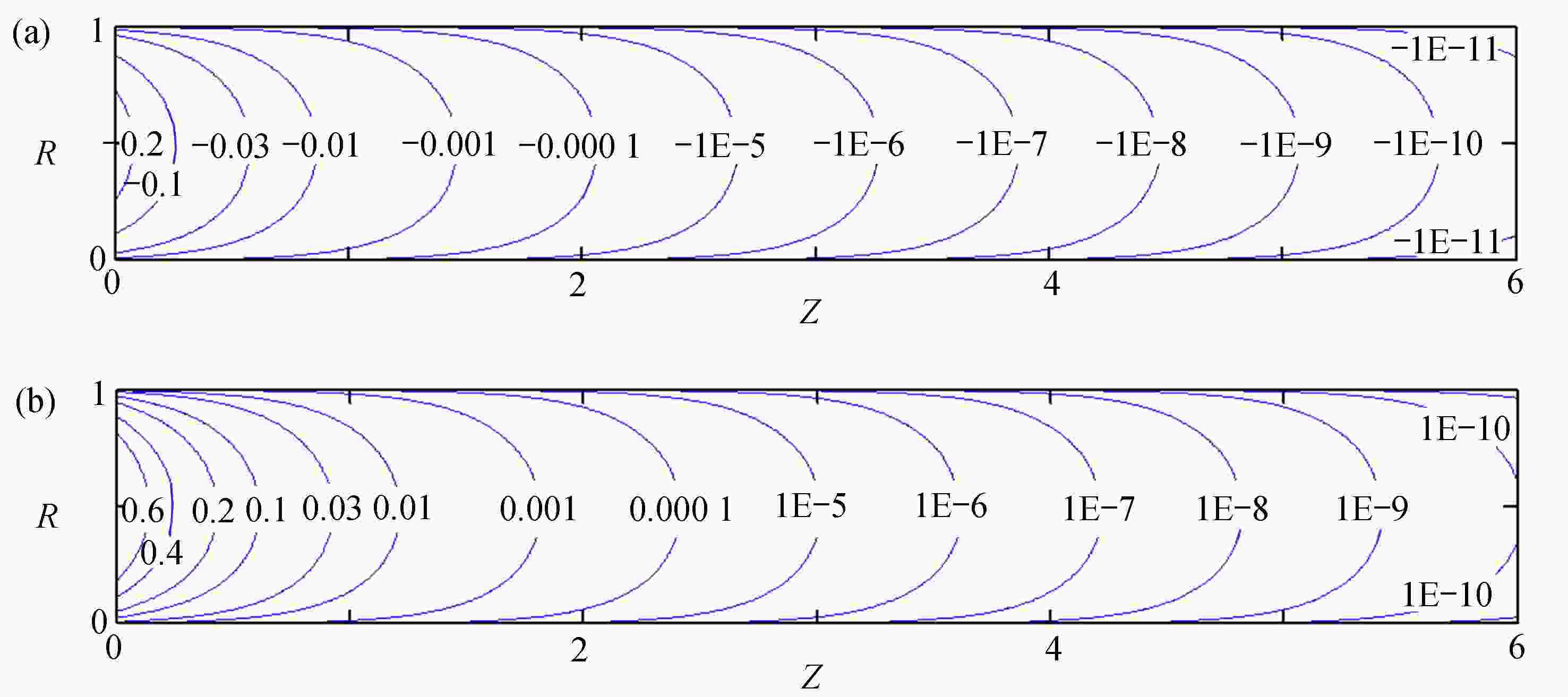
该文以端部旋转的圆柱形容器内的Stokes流为研究对象，根据流动的特点，将轴向坐标模拟为时间，则问题归结为Hamilton对偶方程的本征值和本征解问题。利用本征解空间的完备性和本征解之间的共轭辛正交关系，给出了问题解的展开形式，并建立了展开系数的数值求解方法。采用该方法研究了单端旋转、两端以相同或相反角速度旋转时不同外形比(容器的高度与半径之比)时圆柱形容器内流动速度和应力的分布情况，展示了不同边界条件下流场的一些特点。
Abstract:The Stokes flow in cylindrical containers with rotating ends was studied. Based on the characteristics of the flow, the problem was reduced to the eigenvalue and eigensolution problem of Hamiltonian dual equations with the axial coordinate simulated as the time scale. By means of the completeness of the symplectic eigensolution space and the adjoint symplectic orthogonality relationship between the eigensolutions, the expansion of the solution to the problem was obtained, and the numerical method for calculating the expansion coefficients was given. In the cases of one-end rotating, two-end rotating at the same or opposite angular velocity, the velocity and stress distributions of the flow in the cylindrical containers with different aspect ratios (of the length to the radius), were investigated. The velocity and stress distributions, and the characteristics of the flows under different boundary conditions were revealed.
-
Key words:
- Stokes flow /
- Hamilton /
- rotating end /
- cylindrical container
-
图 2 无限长容器内的流动：(a) 速度
${U_\theta }$ 等高线；(b) 应力$ {\bar \tau _{z\theta }} $ 等高线Figure 2. The flow in the semi-infinite cylindrical container: (a) the contours of velocity
${U_\theta }$ ; (b) the contours of stress$ {\bar \tau _{z\theta }} $ 
图 3 两端部以角速度
$\varOmega = 1$ 同向旋转时，不同外形比的容器内流动速度${U_\theta }$ 的等高线：(a) A=1；(b) A=2；(c) A=6Figure 3. The contours of velocity
${U_\theta }$ in cylindrical containers with two ends rotating at the same angular velocity$\varOmega = 1$ and different geometric aspect ratios: (a) A =1; (b) A=2; (c) A=6
图 4 两端部以角速度
$\varOmega = 1$ 反向旋转时，不同外形比的容器内流动速度${U_\theta }$ 的等高线： (a) A=1；(b) A=2；(c) A=6Figure 4. The contours of velocity
${U_\theta }$ in cylindrical containers with two ends counter rotating at angular velocity$\varOmega = 1$ and different geometric aspect ratios: (a) A=1; (b) A=2; (c) A=6
图 5 端部应力条件时，外形比为
$ A = 6 $ 的容器内的流动：(a) 速度${U_\theta }$ 等高线；(b) 应力$ {\bar \tau _{z\theta }} $ 等高线Figure 5. With stress condition at the end, the flow in the cylindrical container for
$ A = 6 $ : (a) the contours of velocity${U_\theta }$ ; (b) the contours of stress$ {\bar \tau _{z\theta }} $ -
[1] PAO H P. Numerical solution of the Navier-Stokes equations for flows in the disk-cylinder system[J]. Physics of Fluids, 1972, 15(1): 4-11. doi: 10.1063/1.1693752 [2] BERTELÀ M, GORI F. Laminar flow in a cylindrical container with a rotating cover[J]. Journal of Fluids Engineering-Transactions of the ASME, 1982, 104(1): 31-39. doi: 10.1115/1.3240849 [3] DUCK P W. On the flow between two rotating shrouded discs[J]. Computers & Fluids, 1986, 14(3): 183-196. [4] DIJKSTRA D, VAN HEIJST G J F. The flow between two finite rotating disks enclosed by a cylinder[J]. Journal of Fluid Mechanics, 1983, 128: 123-154. doi: 10.1017/S0022112083000415 [5] VOGEL H U. Experimentelle ergebnisse über die laminare strömung in einem zylindrischen gehäuse mit darin rotierender scheibe[D]. PhD Thesis. Göttingen: Max-Planck-Inst für Strömungsforschung, 1968. [6] ESCUDIER M P. Observation of the flow produced in a cylindrical container by a rotating endwall[J]. Experiments in Fluids, 1984, 2: 189-196. doi: 10.1007/BF00571864 [7] VALENTINE D T, JAHNKE C C. Flows induced in a cylinder with both end walls rotating[J]. Physics of Fluids, 1994, 6(8): 2702-2710. doi: 10.1063/1.868159 [8] GELFGAT A Y, BAR-YOSEPH P Z, SOLAN A. Stability of combined swirling flow with and without vortex breakdown[J]. Journal of Fluid Mechanics, 1996, 311(1): 1-36. [9] KAHOUADJI L, WITKOWSKI L M. Free surface due to a flow driven by a rotating disk inside a vertical cylindrical tank: axisymmetric configuration[J]. Physics of Fluids, 2014, 26: 072105. doi: 10.1063/1.4890209 [10] MUKHERJEE A, STEINBERG V. Von Kármán swirling flow between a rotating and a stationary smooth disk: experiment[J]. Physical Review Fluids, 2018, 3: 014102. doi: 10.1103/PhysRevFluids.3.014102 [11] LIM C W, XU X S. Symplectic elasticity: theory and applications[J]. Applied Mechanics Reviews, 2010, 63(5): 050802. doi: 10.1115/1.4003700 [12] 钟万勰. 弹性力学求解新体系[M]. 大连: 大连理工大学出版社, 1995.ZHONG Wanxie. A New Systematic Methodology for Theory of Elasticity[M]. Dalian: Dalian University of Technology Press, 1995. (in Chinese) [13] ZHONG W X. Duality System in Applied Mechanics and Optimal Control[M]. New York: Kluwer Academic Publishers, 2004. [14] 胡启平, 陈哲, 周娟. Hamilton力学下框筒结构剪滞翘曲位移模式研究[J]. 应用数学和力学, 2022, 43(4): 374-381HU Qiping, CHEN Zhe, ZHOU Juan. Research on shear lag warping displacement modes of frame-tube structures based on the Hamiltonian mechanics[J]. Applied Mathematics and Mechanics, 2022, 43(4): 374-381.(in Chinese) [15] 满淑敏, 高强, 钟万勰. 非完整约束Hamilton动力系统保结构算法[J]. 应用数学和力学, 2020, 41(6): 581-590MAN Shumin, GAO Qiang, ZHONG Wanxie. A structure-preserving algorithm for Hamiltonian systems with nonholonomic constraints[J]. Applied Mathematics and Mechanics, 2020, 41(6): 581-590.(in Chinese) [16] 张俊霖, 倪一文, 李庆东, 等. 吸湿老化影响下天然纤维增强复合圆柱壳屈曲分析的辛方法[J]. 应用数学和力学, 2021, 42(12): 1238-1247ZHANG Junlin, NI Yiwen, LI Qingdong, et al. A symplectic approach for buckling analysis of natural fiber reinforced composite shells under hygrothermal aging[J]. Applied Mathematics and Mechanics, 2021, 42(12): 1238-1247.(in Chinese) [17] 张鸿庆, 阿拉坦仓, 钟万勰. Hamilton体系与辛正交系的完备性[J]. 应用数学和力学, 1997, 18(3): 217-221ZHANG Hongqing, ALATANCANG, ZHONG Wanxie. The Hamiltonian system and completeness of symglectic orthogonal system[J]. Applied Mathematics and Mechanics, 1997, 18(3): 217-221.(in Chinese) -
下载:
计量
- 文章访问数: 667
- HTML全文浏览量: 292
- PDF下载量: 100
- 被引次数: 0
渝公网安备50010802005915号