功能梯度压电板柱面弯曲的弹性力学解

沈璐璐; 蔡方圆; 杨博

doi:10.21656/1000-0887.430224

功能梯度压电板柱面弯曲的弹性力学解

doi: 10.21656/1000-0887.430224

浙江理工大学建筑工程学院，杭州 310018

基金项目: 国家自然科学基金(11872336)；机械结构强度与振动国家重点实验室开放课题(SV2020-KF-13)

详细信息

作者简介:
沈璐璐（1990—），女，副教授，博士（E-mail：lulushen@zstu.edu.cn）

蔡方圆（1998—），女，硕士生（E-mail：13588750105@163.com）

杨博（1979—），男，教授，博士（通讯作者. E-mail：youngbo@zstu.edu.cn）

中图分类号: O343.1
计量
- 文章访问数: 668
- HTML全文浏览量: 321
- PDF下载量: 84
- 被引次数: 0
出版历程
- 收稿日期: 2022-07-06
- 录用日期: 2022-09-29
- 修回日期: 2022-09-29
- 网络出版日期: 2022-10-21
- 刊出日期: 2023-03-15

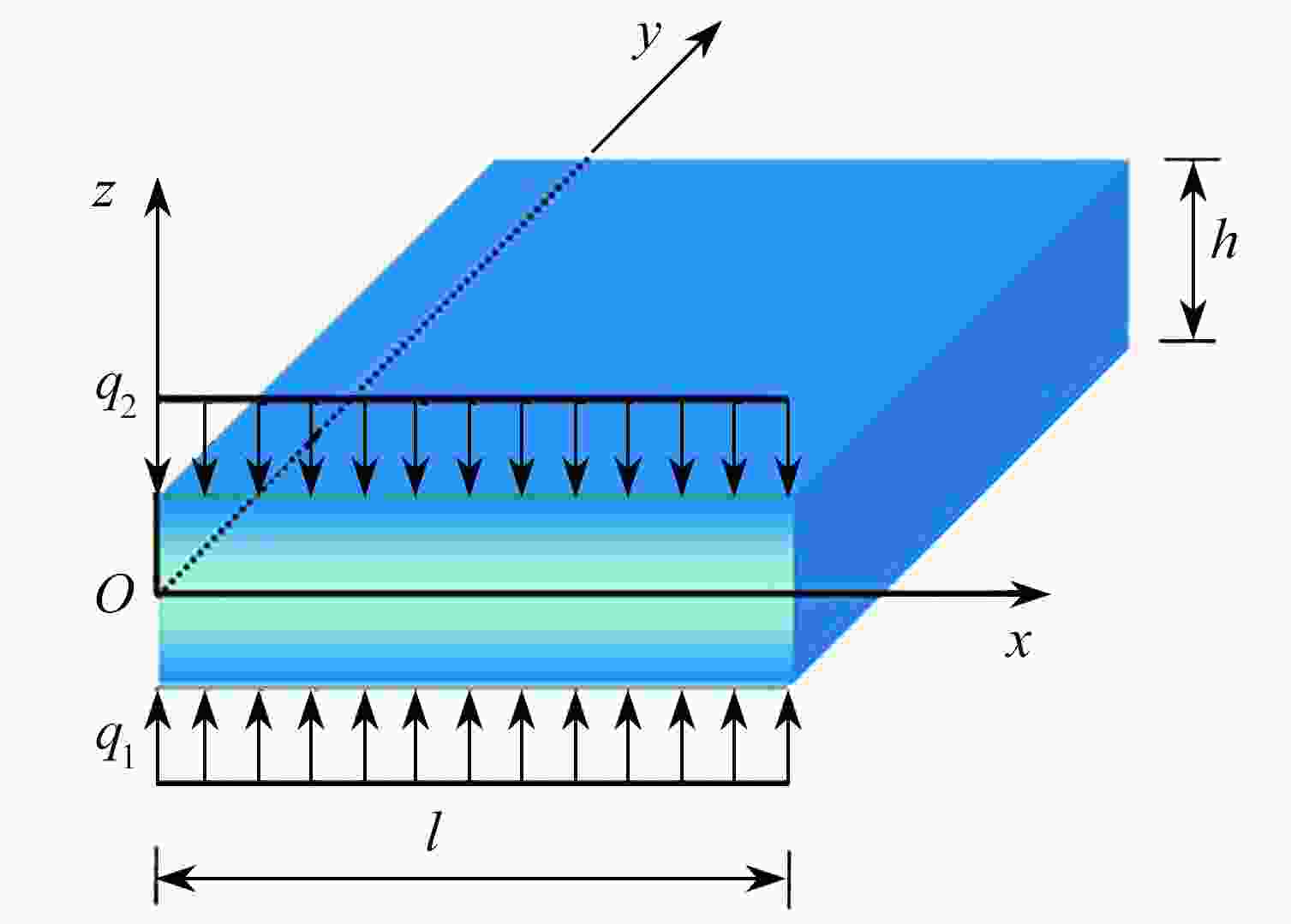
Elasticity Solutions for Cylindrical Bending of Functionally Graded Piezoelectric Material Plates

School of Civil Engineering, Zhejiang Sci-Tech University, Hangzhou 310018, P.R.China

摘要

摘要:
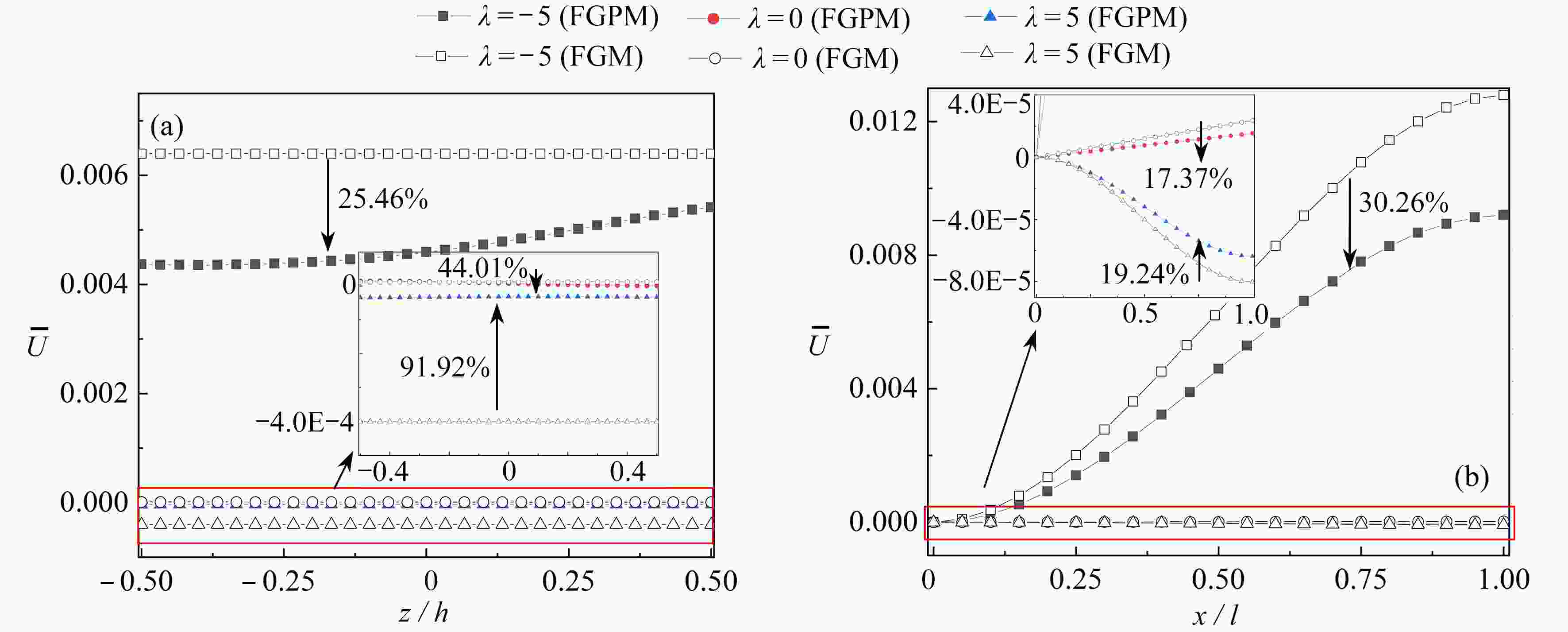
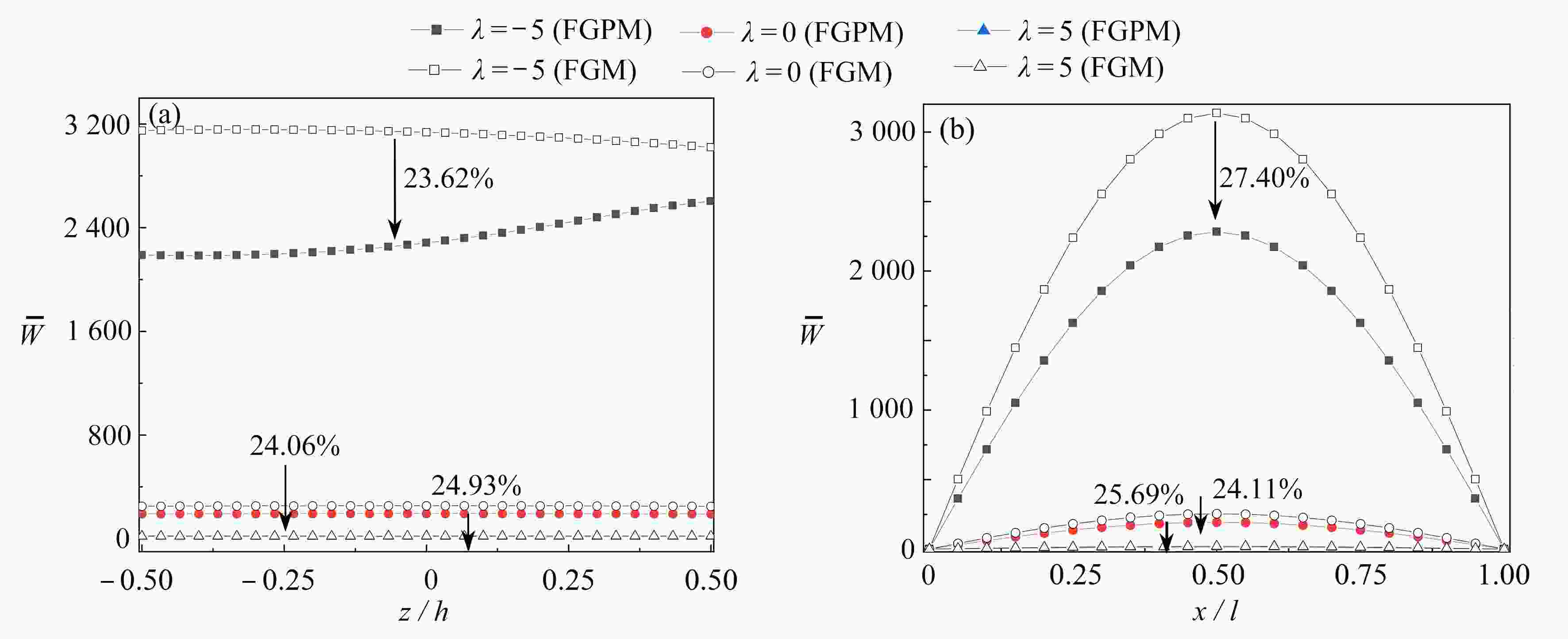
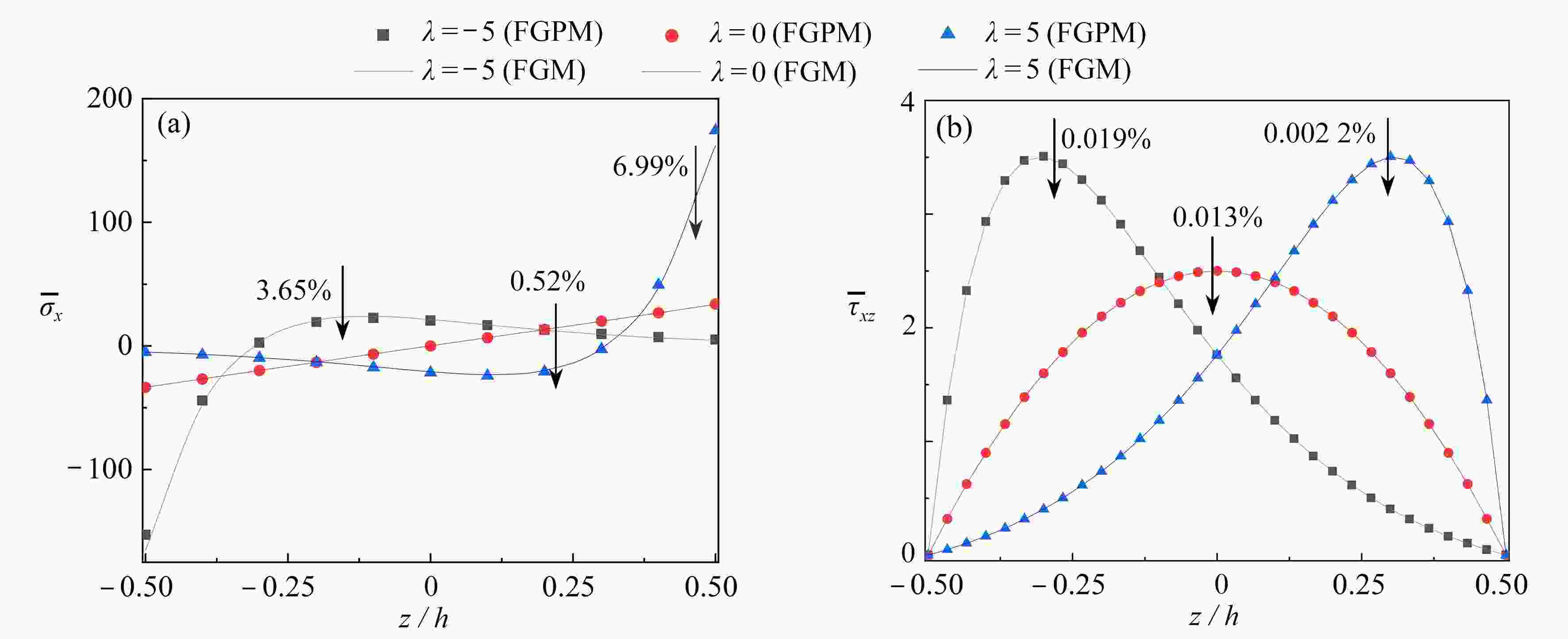
功能梯度压电材料（FGPM）同时兼具功能梯度材料和压电材料特性，可为多功能或智能化轻质结构设计提供支撑，在诸多领域有着广泛的应用前景。将Mian和Spencer功能梯度板理论由功能梯度弹性材料推广到功能梯度压电材料，解析研究了FGPM板的柱面弯曲问题，其中，材料弹性常数、压电和介电参数沿板厚方向可以任意连续变化。最终，给出了FGPM板受横向均布荷载作用下柱面弯曲问题的弹性力学解。通过算例分析，重点讨论了压电效应对FGPM板静力响应的影响。
- 压电效应 /
- Mian和Spencer板理论 /
- 柱面弯曲 /
- 弹性力学解
Abstract:
Functionally graded piezoelectric materials (FGPMs), combining the properties of functionally graded materials and piezoelectric materials, provides a new idea for multi-functional and intelligent lightweight components, and has broad application prospects in electronic devices. Based on the elastic and electric equilibrium equations, the Mian and Spencer functionally graded plate theory was extended from elastic materials to piezoelectric materials to study the cylindrical bending of FGPM plates, where the material elastic constants, piezoelectric and dielectric parameters were assumed to vary continuously and arbitrarily along the thickness direction. Accordingly, the elasticity solutions for cylindrical bending of FGPMs plates under the uniform transverse loading were obtained. Numerical examples were given to demonstrate the piezoelectric effects on the static responses of the presented FGPMs plates.
- piezoelectric effect /
- Mian and Spencer plate theory /
- cylindrical bending /
- elasticity solution

HTML全文

图 1 均布荷载作用下 FGPM 板示意图

Figure 1. Schematic diagram of the FGPM plate under uniform load

下载: 全尺寸图片幻灯片

图 2 无量纲位移$\bar U $对比图

Figure 2. Comparison of dimensionless displacement $\bar U $

下载: 全尺寸图片幻灯片

图 3 无量纲挠度$ \bar W $对比图

Figure 3. Comparison of dimensionless deflection $ \bar W $

下载: 全尺寸图片幻灯片

图 4 无量纲应力对比图

Figure 4. Comparison of dimensionless stress

下载: 全尺寸图片幻灯片

图 5 7种边界条件下的无量纲挠度分布图

Figure 5. Comparison of dimensionless displacement under 7 boundary conditions

下载: 全尺寸图片幻灯片

表 1 $ z = 0 $处的无量纲位移$ \bar W $对比

Table 1. Comparison of dimensionless displacement $\bar W $ at $ z = 0 $

x	0	0.1	0.2	0.3	0.4	0.5	0.6	0.7	0.8	0.9	1.0
this paper	0	61.162	115.183	157.221	183.821	192.924	183.819	157.223	115.184	61.159	0
FEM	0	61.164	114.669	156.379	182.810	191.863	182.822	156.384	114.670	61.164	0
errors δ/%	−	0.003	0.446	0.536	0.550	0.550	0.542	0.534	0.446	0.008	−

下载: 导出CSV

表 2 n=0，z=0处的无量纲位移w/h对比

Table 2. Comparison of dimensionless displacement at n=0，z=0

	x	0.1	0.2	0.3	0.4	0.5	0.6	0.7	0.8	0.9	1.0
ref. [22]	w/h	0.195	0.377	0.520	0.612	0.652	0.601	0.512	0.385	0.205	0
this paper	w/h	0.208	0.392	0.533	0.627	0.658	0.628	0.537	0.391	0.206	0

下载: 导出CSV

表 3 n=10，z=0处的无量纲位移w/h对比

Table 3. Comparison of dimensionless displacement at n=10，z=0

	x	0.1	0.2	0.3	0.4	0.5	0.6	0.7	0.8	0.9	1.0
ref. [22]	w/h	0.582	1.086	1.488	1.747	1.826	1.750	1.480	1.094	0.605	0
this paper	w/h	0.633	1.192	1.618	1.905	1.997	1.907	1.632	1.187	0.627	0

下载: 导出CSV

参考文献(23)

[1]	刘涛, 汪超, 刘庆运. 基于等几何方法的压电功能梯度板动力学及主动振动控制分析[J]. 工程力学, 2020, 37(12): 228-242 doi: 10.6052/j.issn.1000-4750.2020.04.0266 LIU Tao, WANG Chao, LIU Qingyun. Analysis for dynamics and active vibration control of piezoelectric functionally graded plates based on isogeometric method[J]. Engineering Mechanics, 2020, 37(12): 228-242.(in Chinese) doi: 10.6052/j.issn.1000-4750.2020.04.0266
[2]	韩杰才, 徐丽, 王保林, 等. 梯度功能材料的研究进展及展望[J]. 固体火箭技术, 2004, 27(3): 207-215 doi: 10.3969/j.issn.1006-2793.2004.03.012 HAN Jiecai, XU Li, WANG Baolin, et al. Progress and prospects of functional gradient materials[J]. Journal of Solid Rocket Technology, 2004, 27(3): 207-215.(in Chinese) doi: 10.3969/j.issn.1006-2793.2004.03.012
[3]	CARL C M, WU M K, MOY K. Piezoelectric ceramics with functional gradients: a new application in material design[J]. Journal of the American Ceramic Society, 1996, 79(3): 809-812.
[4]	杨博, 丁皓江, 陈伟球. 功能梯度板柱面弯曲的弹性力学解[J]. 应用数学和力学, 2008, 29(8): 905-910 doi: 10.3879/j.issn.1000-0887.2008.08.003 YANG Bo, DING Haojiang, CHEN Weiqiu. Elasticity solutions for functionally graded plates in cylindrial bending[J]. Applied Mathematics and Mechanics, 2008, 29(8): 905-910.(in Chinese) doi: 10.3879/j.issn.1000-0887.2008.08.003
[5]	边祖光. 功能梯度材料板壳结构的耦合问题研究[D]. 博士学位论文. 杭州: 浙江大学, 2005. BIAN Zuguang. On coupled problems of functionally graded materials plates and shells[D]. PhD Thesis. Hangzhou: Zhejiang University, 2005. (in Chinese)
[6]	ALMAJID A, TAYA M, HUDNUT S W, et al. Analysis of out-of-plane displacement and stress field in a piezocomposite plate with functionally graded microstructure[J]. International Journal of Solids and Structures, 2001, 38(19): 3377-3391. doi: 10.1016/S0020-7683(00)00264-X
[7]	ZHONG Z, SHANG E T. Three-dimensional exact analysis of a simply supported functionally gradient piezoelectric plate[J]. International Journal of Solids and Structures, 2003, 40(20): 5335-5352. doi: 10.1016/S0020-7683(03)00288-9
[8]	NAVAZI H M, HADDADPOUR H. Nonlinear cylindrical bending analysis of shear deformable functionally graded plates under different loadings using analytical methods[J]. International Journal of Mechanical Sciences, 2008, 50(12): 1650-1657. doi: 10.1016/j.ijmecsci.2008.08.010
[9]	TAHANI M, MIRZABABAEE S M. Non-linear analysis of functionally graded plates in cylindrical bending under thermomechanical loadings based on a layerwise theory[J]. European Journal of Mechanics A: Solids, 2008, 28(2): 248-256.
[10]	WANG Y, XU R Q, DING H J. Analytical solutions of functionally graded piezoelectric circular plates subjected to axisymmetric loads[J]. Acta Mechanica, 2010, 215(1/4): 287-305.
[11]	ZHONG Z, CHEN S, SHANG E. Analytical solution of a functionally graded plate in cylindrical bending[J]. Mechanics of Advanced Materials and Structures, 2010, 17(8): 595-602. doi: 10.1080/15376494.2010.517729
[12]	刘五祥, 仲政. 任意梯度分布功能梯度板的柱形弯曲分析[J]. 武汉理工大学学报, 2008, 30(4): 106-109 LIU Wuxiang, ZHONG Zheng. Analysis for functionally gradient plate of arbitrary gradient distribution in cylindrical bending[J]. Journal of Wuhan University of Technology, 2008, 30(4): 106-109.(in Chinese)
[13]	LI X Y, WU J, DING H J. 3D analytical solution for a functionally graded transversely isotropic piezoelectric circular plate under tension and bending[J]. International Journal of Engineering Science, 2011, 49(7): 664-676. doi: 10.1016/j.ijengsci.2011.03.001
[14]	LI Y S, PAN E. Static bending and free vibration of a functionally graded piezoelectric microplate based on the modified couple-stress theory[J]. International Journal of Engineering Science, 2015, 97: 40-59. doi: 10.1016/j.ijengsci.2015.08.009
[15]	ZENKOUR A M, HAFED Z S. Bending analysis of functionally graded piezoelectric plates via quasi-3D trigonometric theory[J]. Mechanics of Advanced Materials and Structures, 2020, 27(18): 1551-1562. doi: 10.1080/15376494.2018.1516325
[16]	DEHSARAJI M L, SAIDI A R, MOHAMMADI M. Bending analysis of thick functionally graded piezoelectric rectangular plates using higher-order shear and normal deformable plate theory[J]. Structural Engineering and Mechanics, 2020, 73(3): 259-269.
[17]	王平远, 李成, 姚林泉. 基于非局部应变梯度理论功能梯度纳米板的弯曲和屈曲研究[J]. 应用数学和力学, 2021, 42(1): 15-26 WANG Pingyuan, LI Cheng, YAO Linquan. Bending and buckling of functionally graded nanoplates based on the nonlocal strain gradient theory[J]. Applied Mathematics and Mechanics, 2021, 42(1): 15-26.(in Chinese)
[18]	刘旭, 姚林泉. 热环境中旋转功能梯度纳米环板的振动分析[J]. 应用数学和力学, 2020, 41(11): 1224-1236 LIU Xu, YAO Linquan. Vibration analysis of rotating functionally gradient nanocycle plates in thermal environment[J]. Applied Mathematics and Mechanics, 2020, 41(11): 1224-1236.(in Chinese)
[19]	MIAN M A, SPENCER A J M. Exact solutions for functionally graded and laminated elastic materials[J]. Journal of the Mechanics and Physics of Solids, 1998, 46(12): 2283-2295. doi: 10.1016/S0022-5096(98)00048-9
[20]	孙烨丽, 沈璐璐, 杨博. 功能梯度板中Griffith裂纹尖端应力场的三维解析研究[J]. 应用数学和力学, 2021, 42(1): 36-48 SUN Yeli, SHEN Lulu, YANG Bo. 3D analytical study of stress field at Griffith crack tip in functionally graded plates[J]. Applied Mathematics and Mechanics, 2021, 42(1): 36-48.(in Chinese)
[21]	仲政, 尚尔涛. 功能梯度热释电材料矩形板的三维精确分析[J]. 力学学报, 2003, 35(5): 542-552 doi: 10.3321/j.issn:0459-1879.2003.05.005 ZHONG Zheng, SHANG Ertao. Three dimensional exact analysis of functionally gradient piezothermoelectric material rectangular plate[J]. Acta Mechanica, 2003, 35(5): 542-552.(in Chinese) doi: 10.3321/j.issn:0459-1879.2003.05.005
[22]	NAVAZI H M, HADDADPOUR H, RASEKH M. An analytical solution for nonlinear cylindrical bending of functionally graded plates[J]. Thin-Walled Structures, 2006, 44(11): 1129-1137. doi: 10.1016/j.tws.2006.10.013
[23]	柳彬彬. 功能梯度压电材料矩形板热-电-机械耦合三维分析[D]. 硕士学位论文. 合肥: 合肥工业大学, 2007. LIU Binbin. Three dimensional analysis of thero-meehanical-electric behaviour of functionally gradient piezoelectric material rectangular plate[D]. Maste Thesis. Hefei: Hefei University of Technology, 2007. (in Chinese)

施引文献

资源附件(0)

访问统计

图(5) / 表(3)

计量

文章访问数: 668
HTML全文浏览量: 321
PDF下载量: 84
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

留言板

功能梯度压电板柱面弯曲的弹性力学解

doi: 10.21656/1000-0887.430224

作者简介:
沈璐璐（1990—），女，副教授，博士（E-mail：lulushen@zstu.edu.cn）

蔡方圆（1998—），女，硕士生（E-mail：13588750105@163.com）

杨博（1979—），男，教授，博士（通讯作者. E-mail：youngbo@zstu.edu.cn）

计量

Elasticity Solutions for Cylindrical Bending of Functionally Graded Piezoelectric Material Plates

计量

目录

留言板

功能梯度压电板柱面弯曲的弹性力学解

doi: 10.21656/1000-0887.430224

作者简介: 沈璐璐（1990—），女，副教授，博士 （E-mail：lulushen@zstu.edu.cn） 蔡方圆（1998—），女，硕士生 （E-mail：13588750105@163.com） 杨博（1979—），男，教授，博士（通讯作者. E-mail：youngbo@zstu.edu.cn）

计量

出版历程

Elasticity Solutions for Cylindrical Bending of Functionally Graded Piezoelectric Material Plates

计量

出版历程

目录

作者简介:
沈璐璐（1990—），女，副教授，博士（E-mail：lulushen@zstu.edu.cn）

蔡方圆（1998—），女，硕士生（E-mail：13588750105@163.com）

杨博（1979—），男，教授，博士（通讯作者. E-mail：youngbo@zstu.edu.cn）