Adaptive Evolution Analysis of a Predator-Prey Model With Group Defense
-
摘要:
基于适应性动力学的理论框架,该文研究了具有群体防御效应的功能反应函数的捕食-被捕食模型关于捕食者处理时间的进化问题。首先,考虑捕食者种群具有种间竞争的相互作用,研究单个捕食者种群能否通过进化分支分裂为两个策略不同的种群。其次,考虑研究当模型生态平衡态不稳定,系统出现周期振荡的极限环时,种群共存在进化上的稳定性。最后,与具有Holling-Ⅱ型功能反应函数的相关模型结论进行对比分析,通过分析猎物承载能力对可行策略的影响,揭示群体防御效应对捕食者进化策略的影响。
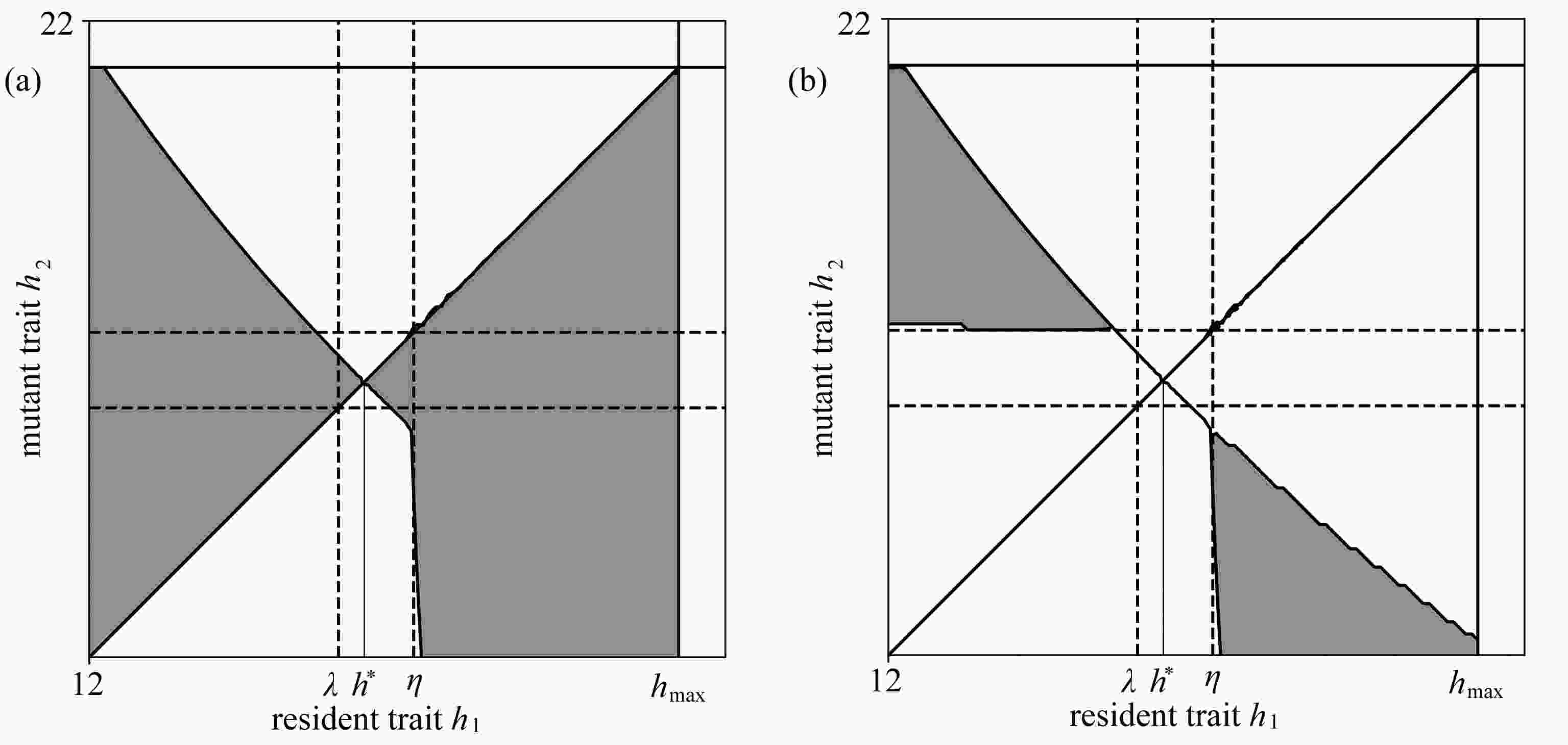
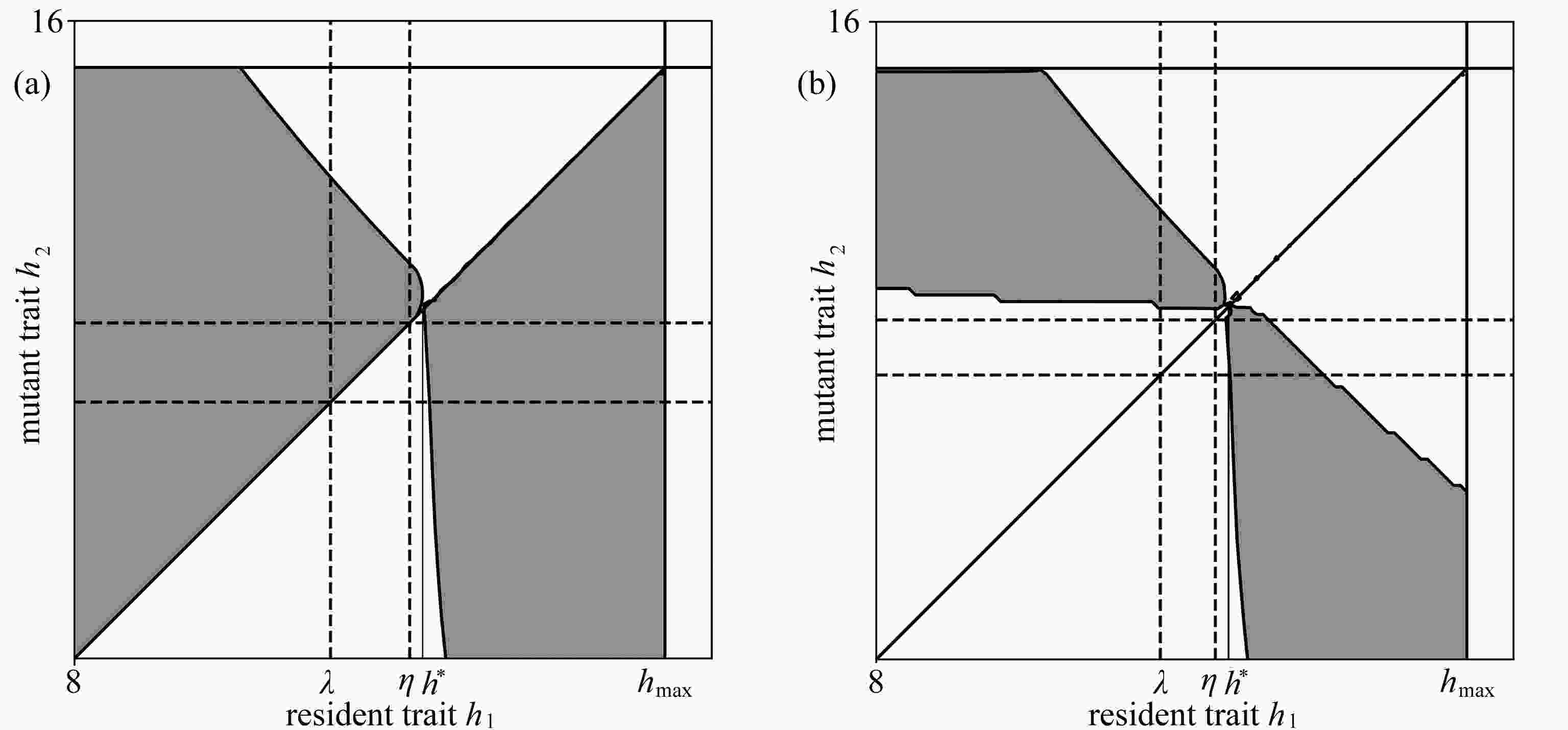
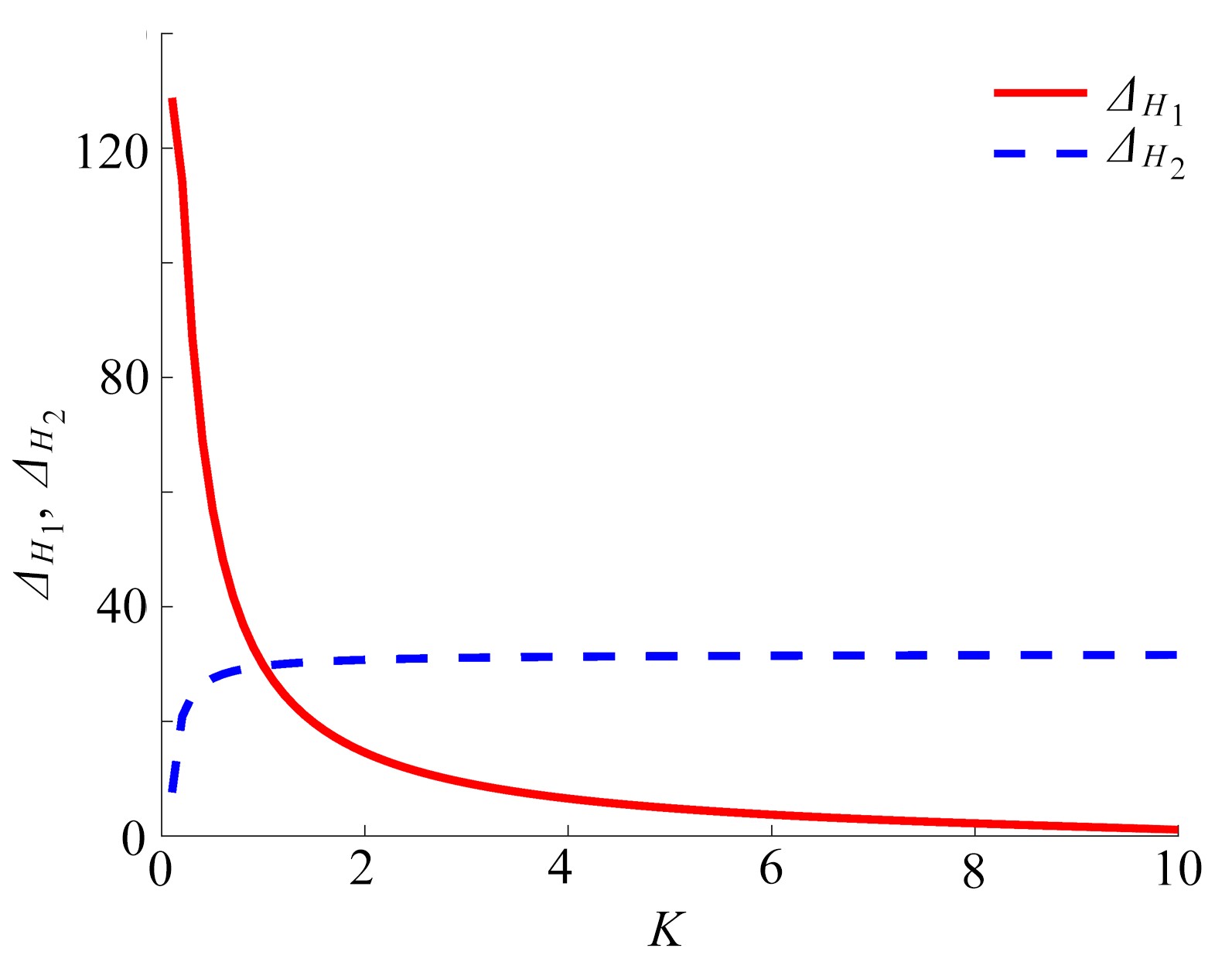
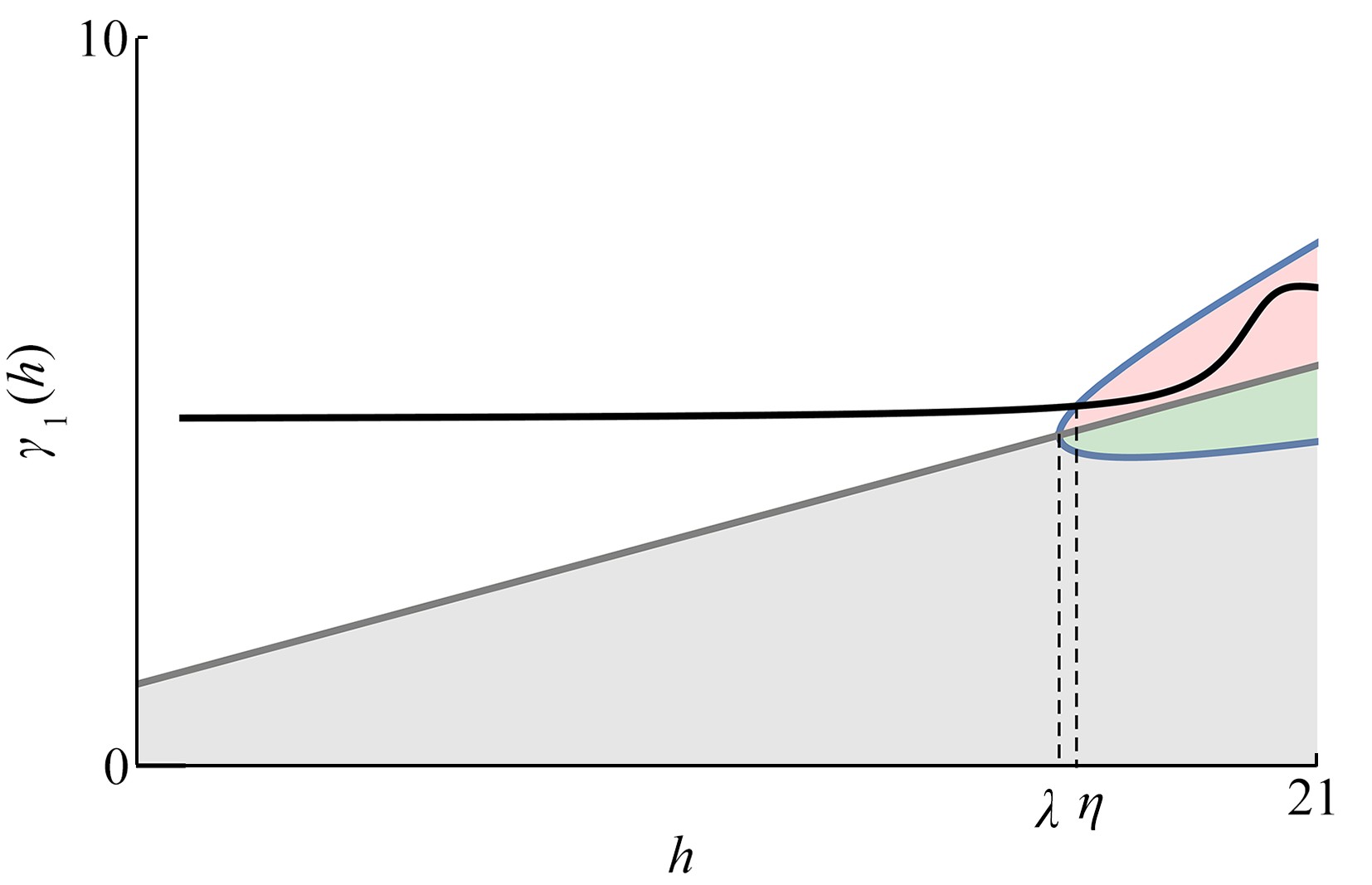
Abstract:Based on the theoretical framework of adaptive dynamics, the evolution of the predator-prey model with functional response of group defense effect on the predator handling time, was investigated. Firstly, in view of the interaction of predator populations with interspecific competition, the evolutionary conditions for a single predator population to split into 2 populations with different strategies through evolutionary branching were given. Secondly, when the ecological equilibrium of the model is unstable and the system has a limit cycle, the population will have strong coexistence under large mutation, but this coexistence will be evolutionarily unstable. Finally, the conclusions for the model with Holling-Ⅱ type functional response were compared. The results indicate that, with a sufficiently large prey carrying capacity, group defense effects can evolutionarily lead to the extinction of predators.
-
Key words:
- adaptive dynamics /
- group defense /
- handling time /
- evolutionary coexistence
-
-
[1] 李自珍, 韩晓卓, 李文龙. 具有生态位构建作用的种群进化动力学模型及其应用研究[J]. 应用数学和力学, 2006, 27(3): 293-299 doi: 10.3321/j.issn:1000-0887.2006.03.007LI Zizhen, HAN Xiaozhuo, LI Wenlong. Evolutionary dynamic model of population with niche construction and its application research[J]. Applied Mathematics and Mechanics, 2006, 27(3): 293-299.(in Chinese) doi: 10.3321/j.issn:1000-0887.2006.03.007 [2] DERCOLE F, RINALDI S. Analysis of Evolutionary Processes: the Adaptive Dynamics Approach and Its Applications[M]. Princeton University Press, 2008. [3] RUEFFLER C, VAN DOOREN T J M, METZ J A J. Adaptive walks on changing landscapes: Levins’ approach extended[J]. Theoretical Population Biology, 2004, 65(2): 165-178. doi: 10.1016/j.tpb.2003.10.001 [4] METZ J A J, NISBET R M, GERITZ S A H. How should we define ‘fitness’ for general ecological scenarios?[J]. Trends in Ecology & Evolution, 1992, 7(6): 198-202. [5] DIECKMANN U, LAW R. The dynamical theory of coevolution: a derivation from stochastic ecological processes[J]. Journal of Mathematical Biology, 1996, 34(5): 579-612. [6] GERITZ S A H, KISDI E, METZ J A J, et al. Evolutionarily singular strategies and the adaptive growth and branching of the evolutionary tree[J]. Evolutionary Ecology, 1998, 12(1): 35-57. doi: 10.1023/A:1006554906681 [7] ZU J, WANG J, DU J. Adaptive evolution of defense ability leads to diversification of prey species[J]. Acta Biotheoretica, 2014, 62(2): 207-234. doi: 10.1007/s10441-014-9218-8 [8] HARDIN G. The competitive exclusion principle[J]. Science, 1960, 131(3409): 1292-1297. doi: 10.1126/science.131.3409.1292 [9] VOLTERRA V. Variazioni e Flluttuazioni del Numero D’individui in Specie Animali Conviventi[M]. Società Anonima Tipografica “Leonardo da Vinci”, 1926. [10] MACARTHUR R H, LEVINS R. Competition, habitat selection, and character displacement in a patchy environment[J]. Proceedings of the National Academy of Sciences, 1964, 51(6): 1207-1210. doi: 10.1073/pnas.51.6.1207 [11] TSOULARIS A. A learning strategy for predator preying on edible and inedible prey[J]. Acta Biotheoretica, 2007, 55(3): 283-295. doi: 10.1007/s10441-007-9020-y [12] ITO H C, SHIMADA M, IKEGAMI T. Coevolutionary dynamics of adaptive radiation for food-web development[J]. Population Ecology, 2009, 51(1): 65-81. doi: 10.1007/s10144-008-0113-5 [13] YAMAGUCHI W, KONDOH M, KAWATA M. Effects of evolutionary changes in prey use on the relationship between food web complexity and stability[J]. Population Ecology, 2011, 53(1): 59-72. doi: 10.1007/s10144-010-0212-y [14] ROSENZWEIG M L, MACARTHUR R H. Graphical representation and stability conditions of predator-prey interactions[J]. The American Naturalist, 1963, 97(895): 209-223. doi: 10.1086/282272 [15] ARMSTRONG R A, MCGEHEE R. Competitive exclusion[J]. The American Naturalist, 1980, 115(2): 151-170. doi: 10.1086/283553 [16] ABRAMS P A, HOLT R D. The impact of consumer-resource cycles on the coexistence of competing consumers[J]. Theoretical Population Biology, 2002, 62(3): 281-295. doi: 10.1006/tpbi.2002.1614 [17] 柳文清, 陈清婉. 捕食者食饵均染病的入侵反应扩散捕食系统中扩散的作用[J]. 应用数学和力学, 2019, 40(3): 321-331 doi: 10.1007/s10483-019-2443-9LIU Wenqing, CHEN Qingwan. Influence of diffusion on an invasion diffusion prey-predator model with disease infection in both populations[J]. Applied Mathematics and Mechanics, 2019, 40(3): 321-331.(in Chinese) doi: 10.1007/s10483-019-2443-9 [18] KISDI E, LIU S. Evolution of handling time can destroy the coexistence of cycling predators[J]. Journal of Evolutionary Biology, 2006, 19(1): 49-58. doi: 10.1111/j.1420-9101.2005.00993.x [19] GERITZ S A H, KISDI E, YAN P. Evolutionary branching and long-term coexistence of cycling predators: critical function analysis[J]. Theoretical Population Biology, 2007, 71(4): 424-435. doi: 10.1016/j.tpb.2007.03.006 [20] SOKOL W, HOWELL J A. Kinetics of phenol oxidation by washed cells[J]. Biotechnology and Bioengineering, 1981, 23(9): 2039-2049. doi: 10.1002/bit.260230909 [21] METZ J A J, GERITZ S A H, MESZENA G, et al. Adaptive dynamics: a geometrical study of the consequences of nearly faithful reproduction: Working Papers wp95099[R]. International Institute for Applied Systems Analysis, 1995. [22] XIAO D, RUAN S. Global analysis in a predator-prey system with nonmonotonic functional response[J]. SIAM Journal on Applied Mathematics, 2001, 61(4): 1445-1472. doi: 10.1137/S0036139999361896 [23] CHRISTIANSEN F B. On conditions for evolutionary stability for a continuously varying character[J]. The American Naturalist, 1991, 138(1): 37-50. doi: 10.1086/285203 [24] SMITH J M. Evolution and the Theory of Games[M]. Cambridge: Cambridge University Press, 1982. [25] DE MAZANCOURT C, DIECKMANN U. Trade-off geometries and frequency-dependent selection[J]. The American Naturalist, 2004, 164(6): 765-778. doi: 10.1086/424762 [26] ESHEL I. Evolutionary and continuous stability[J]. Journal of Theoretical Biology, 1983, 103(1): 99-111. doi: 10.1016/0022-5193(83)90201-1 [27] CRESSMAN R. CSS, NIS and dynamic stability for two-species behavioral models with continuous trait spaces[J]. Journal of Theoretical Biology, 2010, 262(1): 80-89. doi: 10.1016/j.jtbi.2009.09.019 [28] GERITZ S A H. Resident-invader dynamics and the coexistence of similar strategies[J]. Journal of Mathematical Biology, 2005, 50(1): 67-82. doi: 10.1007/s00285-004-0280-8 [29] ZU J, WANG K, MIMURA M. Evolutionary branching and evolutionarily stable coexistence of predator species: critical function analysis[J]. Mathematical Biosciences, 2011, 231(2): 210-224. doi: 10.1016/j.mbs.2011.03.007 [30] MYLIUS S D, DIEKMANN O. On evolutionarily stable life histories, optimization and the need to be specific about density dependence[J]. Oikos, 1995, 74(2): 218-224. doi: 10.2307/3545651 [31] METZ J AJ, MYLIUS S D, DIEKMANN O. When does evolution optimize? On the relationship between evolutionary stability, optimization and density dependence: Working Papers wp96004[R]. International Institute for Applied Systems Analysis, 1996. [32] MESZENA G, KISDI E, DIECKMANN U, et al. Evolutionary optimisation models and matrix games in the unified perspective of adaptive dynamics[J]. Selection, 2002, 2(1/2): 193-220. doi: 10.1556/Select.2.2001.1-2.14 [33] FREEDMAN H I, WOLKOWICZ G S K. Predator-prey systems with group defence: the paradox of enrichment revisited[J]. Bulletin of Mathematical Biology, 1986, 48(5/6): 493-508. -




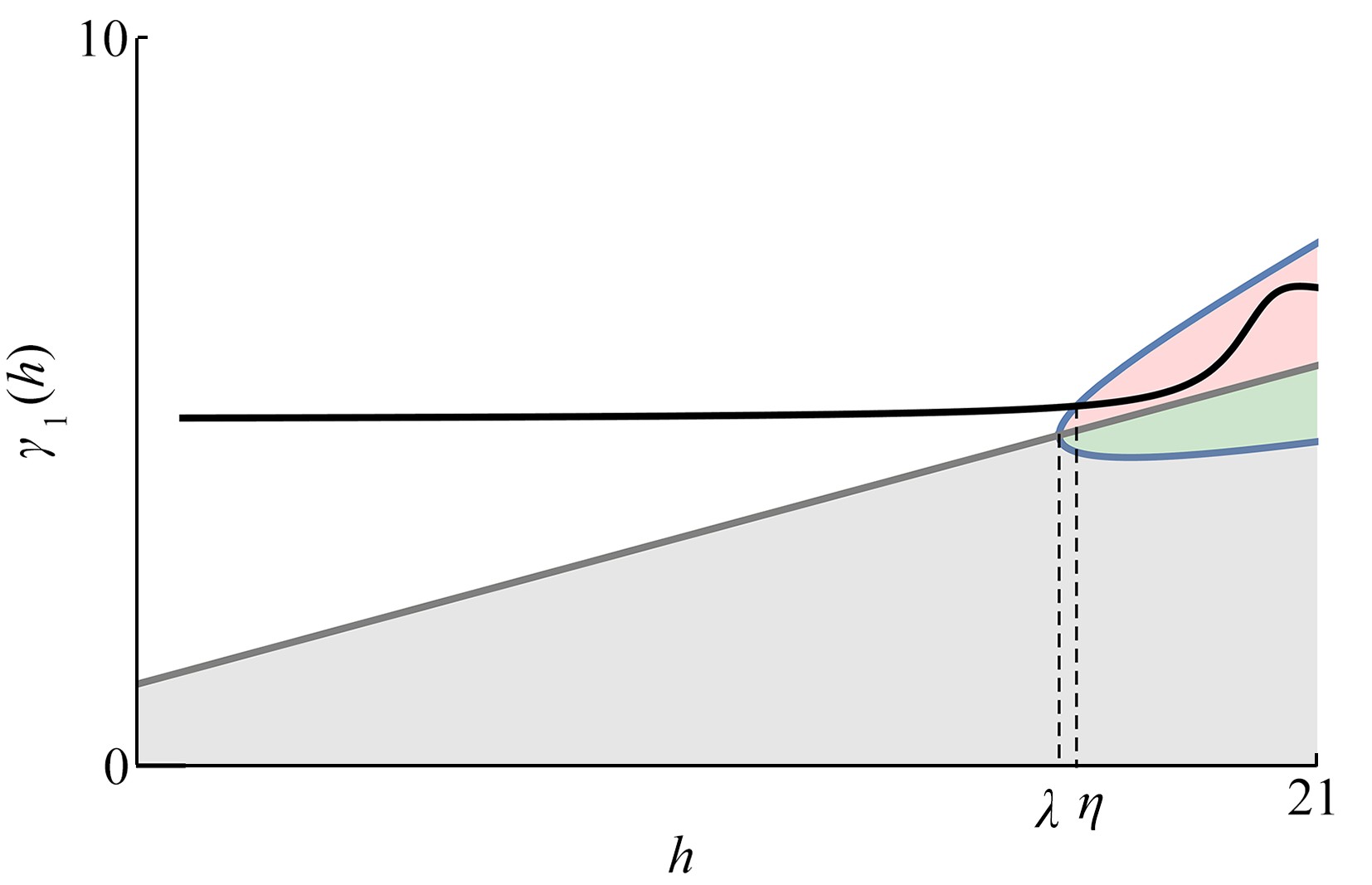


 下载:
下载:
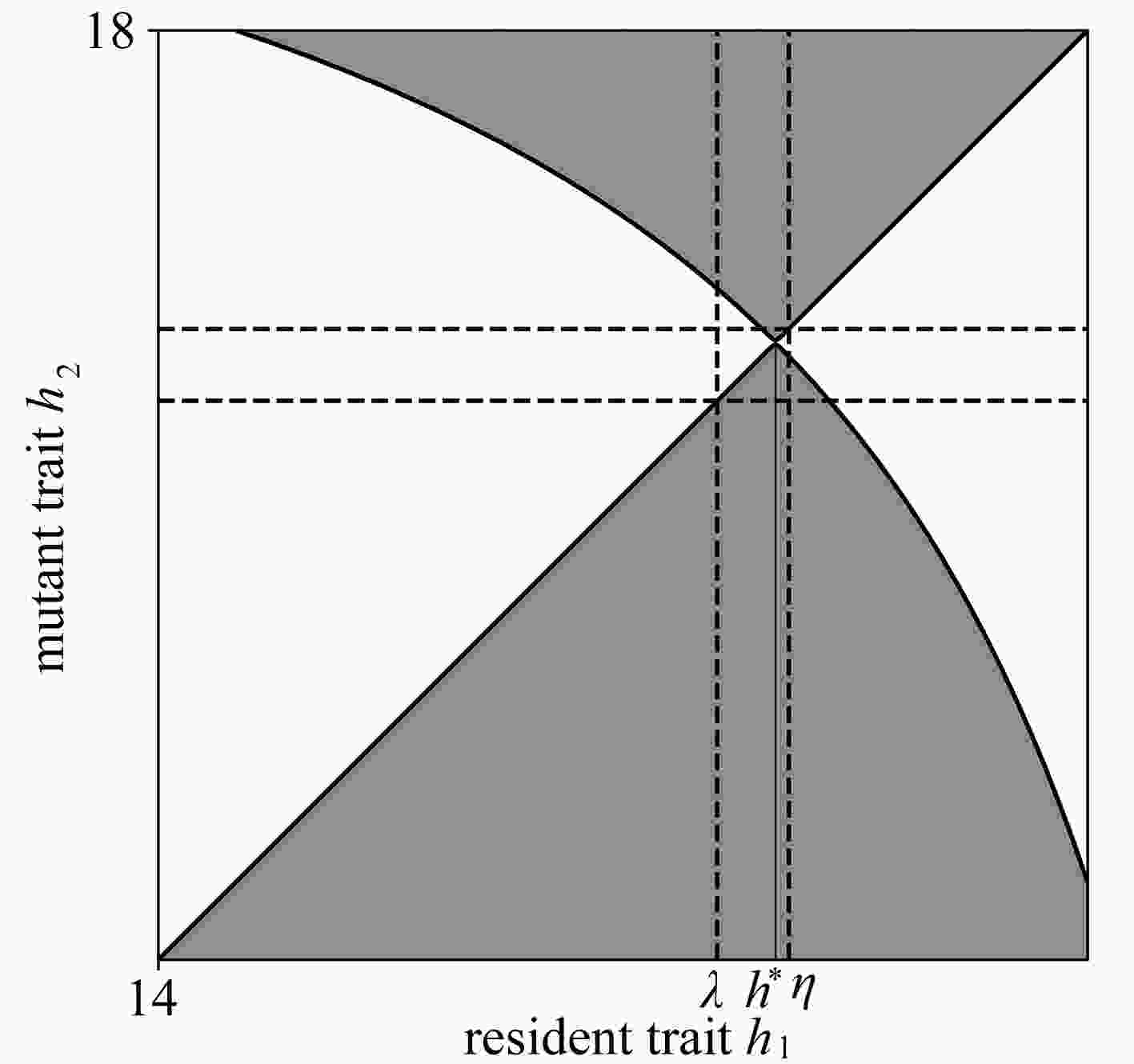


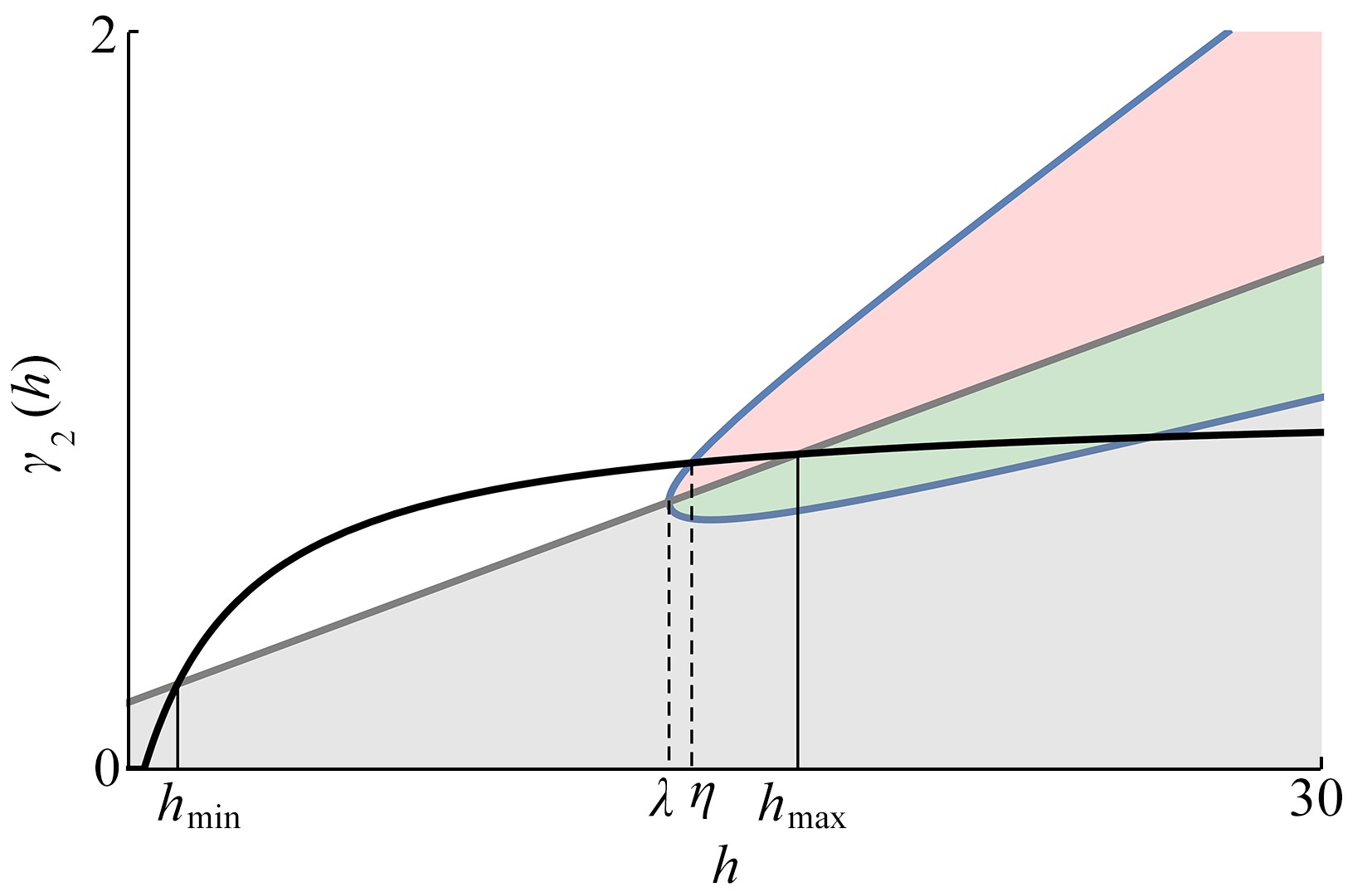


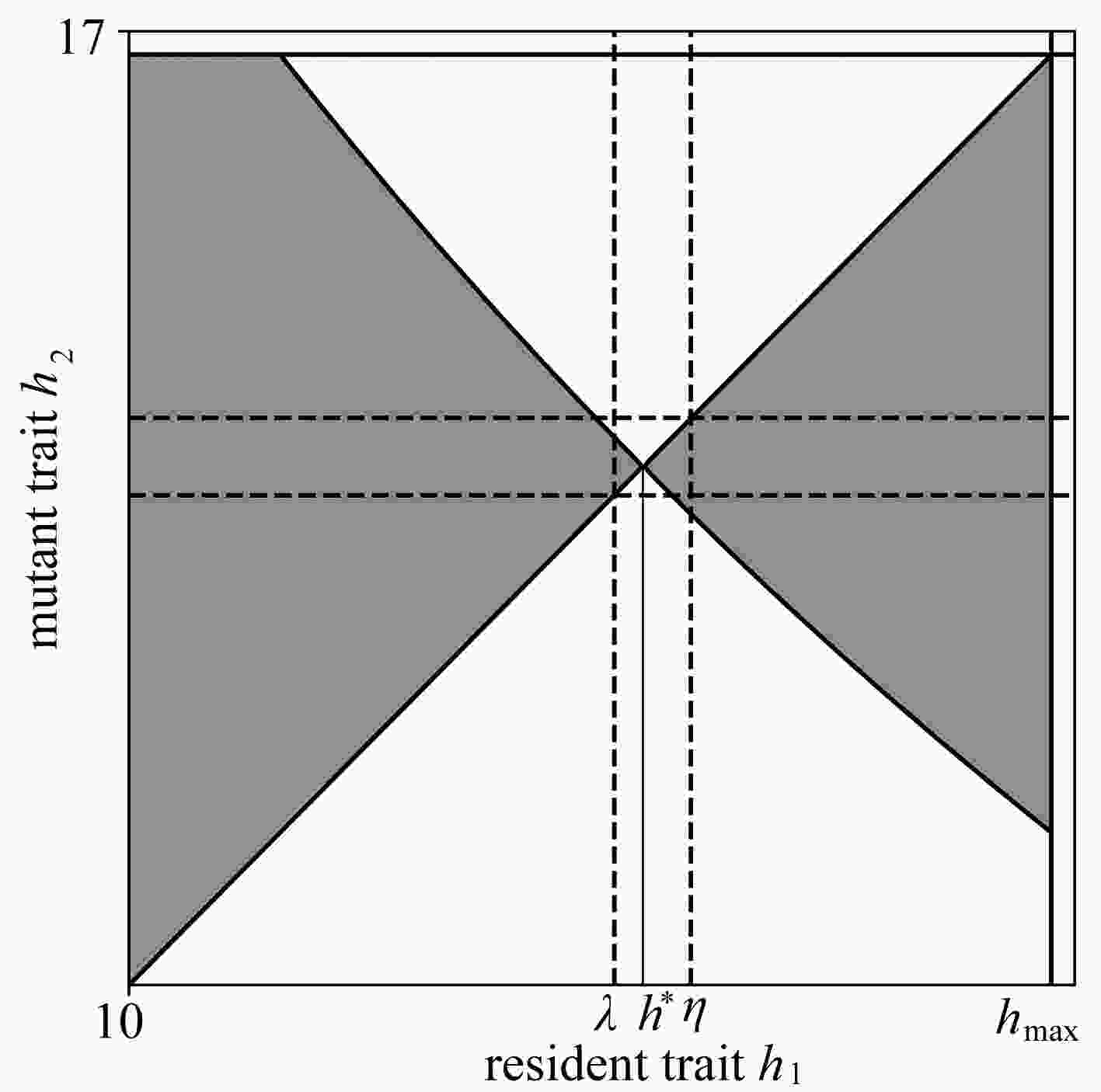


















 渝公网安备50010802005915号
渝公网安备50010802005915号