Numerical Simulation of Hydraulic Fractures Intersecting Natural Fractures in Shale With Plastic Deformation
-
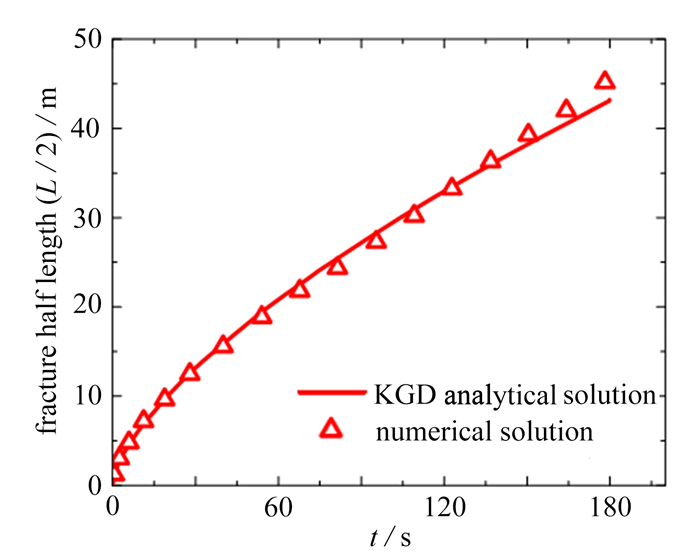
摘要: 水力压裂中，页岩的塑性变形和大量天然弱界面的存在，给水力裂缝扩展形态的预测带来了巨大的挑战.该文基于有限元法建立了一个完全耦合的弹塑性水力压裂数值模型，并考虑了天然裂缝和层理面.数值模型得到了KGD解析解和Blanton曲线的验证.模拟结果显示：与线弹性的水力压裂结果相比，岩石中的塑性变形使得水力裂缝更容易进入天然弱界面；裂缝扩展过程中，岩石塑性变形区域主要集中在储层内；当岩石发生韧性破坏时，水力裂缝更容易贯穿层理面；水力裂缝在高注入速率下，得益于较大驱动力，裂缝能够直接穿过天然裂缝和层理面.研究结果为页岩储藏中水力裂缝的扩展规律提供了新的认识.Abstract: The plastic deformation and numerous natural joints of shale pose a great challenge for the prediction of the hydraulic fracture geometry extension. Based on the finite element method, a fully coupled numerical model for elastoplastic hydraulic fractures was established with natural fractures and bedding planes considered. The numerical model was validated with the KGD analytical solution and Blanton's curve. The numerical results show that, compared with the numerical model solution of linear elasticity, the hydraulic fractures are prone to enter the natural weak interface due to the rock plastic deformation. The rock plastic deformation area mainly lies in the reservoir layer during the fracture propagation. In the case of rock ductile damage, the hydraulic fracture is more likely to penetrate the bedding plane. Hydraulic fractures can directly penetrate natural fractures and bedding planes at high injection rates due to large driving forces. The study provides new insights in terms of hydraulic fracture extension in elastoplastic formations.
-
Key words:
- elastoplastic formation /
- natural fracture /
- bedding plane /
- hydraulic fracture /
- fracture morphology
-
图 2 水力裂缝与天然裂缝交叉角度-地应力差曲线图
Figure 2. The approaching angle between the hydraulic fracture and the natural fracture-stress difference curve
图 3 分层页岩模型的几何形状和边界条件
Figure 3. Geometric and boundary conditions of the layered shale model
图 4 不同注入速率下弹性模型(左)和弹塑性模型(右)裂缝几何形态对比
注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.
Figure 4. Comparison of crack morphologies between the elastic model (left) and the elasto-plastic model (right)at different injection rates
图 5 分布在裂缝附近的等效塑性应变
Figure 5. Equivalent plastic strains distributed in the vicinity of the crack
图 6 储层和隔层中水力裂缝在不同强度下的断裂机制(方案A)
Figure 6. Fracture mechanisms of hydraulic fractures in the pay zone and the barrier at different strengths(plan A)
图 7 储层和隔层中水力裂缝在不同强度下的断裂机制(方案B)
Figure 7. Fracture mechanisms of hydraulic fractures in the pay zone and the barrier at different strengths (plan B)
图 8 裂缝形态随水力裂缝与层理面的交叉角度的变化
Figure 8. Variations of the fracture morphology with the approaching angle between the hydraulic fracture and the bedding plane
图 9 裂缝扩展随地应力差的变化
Figure 9. Variations of the crack extension with the stress difference
图 10 裂缝扩展随层理面抗拉强度的变化
Figure 10. Variations of the crack extension with the tensile strength of the bedding plane
图 11 裂缝扩展随注入速率的变化
Figure 11. Variations of the fracture extension with the injection rate
图 12 分层页岩中交叉角度-地应力差的散点图
Figure 12. The scatter plot of the stress difference vs.the approaching angle in the layered shale
表 1 分层页岩的输入参数
Table 1. The input parameters of the layered shale
parameter pay zone barrier bedding plane height H/m 10 10 - elastic modulus E/GPa 15 20 20 Poisson’s ratio ν 0.25 0.2 - permeability k/mD 10 1 - rock tensile strength T/MPa 1 2 1.5 vertical in-situ stress Vmax/MPa 9 9 - minimum horizontal in-situ stress hmin/MPa 5 7 - maximum horizontal in-situ stress hmax/MPa 8 8 - fluid leakoff coefficient (HF & NF) km/(m/(Pa·s)) 1×10-13 1×10-14 1×10-13 fluid viscosity μ/(Pa·s) 0.001 0.001 0.001 
下载: 导出CSV
表 2 页岩的塑性参数
Table 2. The input plastic parameters of the shale
parameter value hardening constant hr0 0.35 hardening parameter ξ 0.01 yield stress c/MPa 11.25 yield parameter α -0.365 yield parameter β 1.980 4 approaching angle θ/(°) 80
下载: 导出CSV
-
[1] WENG X. Modeling of complex hydraulic fractures in naturally fractured formation[J]. Journal of Unconventional Oil and Gas Resources, 2015, 9: 114-135. doi: 10.1016/j.juogr.2014.07.001 [2] SHANG X, LIU J, GAO X, et al. Analysis on nonlinear effect of unsteady percolation in the inhomogeneous shale gas reservoir[J]. Applied Mathematics and Mechanics, 2020, 41(1): 105-122. doi: 10.1007/s10483-020-2553-5 [3] LIU C, ZHAO A G, WU H A. Competition growth of biwing hydraulic fractures in naturally fractured reservoirs[J]. Gas Science and Engineering, 2023, 109: 204873. doi: 10.1016/j.jgsce.2023.204873 [4] CAO Y, HE Q, LIU C. Numerical investigation of fracture morphology characteristics in heterogeneous reservoirs[J]. Processes, 2022, 10(12): 2604. doi: 10.3390/pr10122604 [5] FLEWELLING S A, TYMCHAK M P, WARPINSKI N. Hydraulic fracture height limits and fault interactions in tight oil and gas formations[J]. Geophysical Research Letters, 2013, 40(14): 3602-3606. doi: 10.1002/grl.50707 [6] SUN C, ZHENG H, LIU W D, et al. Numerical simulation analysis of vertical propagation of hydraulic fracture in bedding plane[J]. Engineering Fracture Mechanics, 2020, 232: 107056. doi: 10.1016/j.engfracmech.2020.107056 [7] VAHAB M, HIRMAND M R, JAFARI A, et al. Numerical analysis of multiple hydro-fracture growth in layered media based on a non-differentiable energy minimization approach[J]. Engineering Fracture Mechanics, 2021, 241: 107361. doi: 10.1016/j.engfracmech.2020.107361 [8] DEHGHAN A N. An experimental investigation into the influence of pre-existing natural fracture on the behavior and length of propagating hydraulic fracture[J]. Engineering Fracture Mechanics, 2020, 240: 107330. doi: 10.1016/j.engfracmech.2020.107330 [9] ZOU J, JIAO Y Y, TAN F, et al. Complex hydraulic-fracture-network propagation in a naturally fractured reservoir[J]. Computers and Geotechnics, 2021, 135: 104165. doi: 10.1016/j.compgeo.2021.104165 [10] YANG Y, JIAO Z, LONGHUAN D, et al. Numerical simulation of shale reservoir fluid-driven fracture network morphology based on global CZM[J]. Frontiers in Earth Science, 2021, 9: 775446. doi: 10.3389/feart.2021.775446 [11] LAN H, MARTIN C D, HU B. Effect of heterogeneity of brittle rock on micromechanical extensile behavior during compression loading[J]. Journal of Geophysical Research: Solid Earth, 2010, 115: B01202. [12] ZHANG Y Z, DUSSEAULT M B, YASSIR N A. Effects of rock anisotropy and heterogeneity on stress distributions at selected sites in North America[J]. Engineering Geology, 1994, 37(3/4): 181-197. [13] WU M, WANG W, SONG Z, et al. Exploring the influence of heterogeneity on hydraulic fracturing based on the combined finite-discrete method[J]. Engineering Fracture Mechanics, 2021, 252: 107835. doi: 10.1016/j.engfracmech.2021.107835 [14] YI L P, WAISMAN H, YANG ZZ, et al. A consistent phase field model for hydraulic fracture propagation in poroelastic media[J]. Computer Methods in Applied Mechanics and Engineering, 2020, 372: 113396. doi: 10.1016/j.cma.2020.113396 [15] YOSHIOKA K, MOLLAALI M, KOLDITZ O. Variational phase-field fracture modeling with interfaces[J]. Computer Methods in Applied Mechanics and Engineering, 2021, 384: 113951. doi: 10.1016/j.cma.2021.113951 [16] JU Y, LIU P, CHEN J, et al. CDEM-based analysis of the 3D initiation and propagation of hydrofracturing cracks in heterogeneous glutenites[J]. Journal of Natural Gas Science and Engineering, 2016, 35: 614-623. doi: 10.1016/j.jngse.2016.09.011 [17] LI X, JAFFAL H, FENG Y, et al. Wellbore breakouts: Mohr-Coulomb plastic rock deformation, fluid seepage, and time-dependent mudcake buildup[J]. Journal of Natural Gas Science and Engineering, 2018, 52: 515-528. doi: 10.1016/j.jngse.2018.02.006 [18] 范白涛, 邓金根, 刘伟, 等. 地层塑性对水力压裂裂缝扩展影响的数值模拟[J]. 科学技术与工程, 2018, 18(28): 65-71. doi: 10.3969/j.issn.1671-1815.2018.28.008FAN Baitao, DENG Jingen, LIU Wei, et al. Numerical simulation of the effect of formation plasticity on the propagation of hydraulic fractures[J]. Science Technology and Engineering, 2018, 18(28): 65-71. (in Chinese) doi: 10.3969/j.issn.1671-1815.2018.28.008 [19] 唐红梅, 周福川, 陈松, 等. 高烈度下双裂缝主控结构面危岩的断裂破坏机制分析[J]. 应用数学和力学, 2021, 42(6): 645-655. doi: 10.21656/1000-0887.410187TANG Hongmei, ZHOU Fuchuan, CHEN Song, et al. Fracture mechanism of unstable rock with double-crack control discontinuity subjected to high earthquake intensities[J]. Applied Mathematics and Mechanics, 2021, 42(6): 645-655. (in Chinese) doi: 10.21656/1000-0887.410187 [20] 冯国益, 肖俊华, 苏梦雨. 考虑表面效应时孔边均布径向多裂纹Ⅲ型断裂力学分析[J]. 应用数学和力学, 2020, 41(4): 376-385. doi: 10.21656/1000-0887.400177FENG Guoyi, XIAO Junhua, SU Mengyu. Fracture mechanics analysis of mode-Ⅲ radial multi cracks on the edge of a hole with surface effects[J]. Applied Mathematics and Mechanics, 2020, 41(4): 376-385. (in Chinese) doi: 10.21656/1000-0887.400177 [21] 姚军, 黄朝琴, 刘文政, 等. 深层油气藏开发中的关键力学问题[J]. 中国科学: 物理学力学天文学, 2018, 48(4): 5-31. https://www.cnki.com.cn/Article/CJFDTOTAL-JGXK201804001.htmYAO Jun, HUANG Zhaoqin, LIU Wenzheng, et al. Key mechanical problems in the development of deep oil and gas reservoirs[J]. Scientia Sinica: Physica, Mechanica & Astronomica, 2018, 48(4): 5-31. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JGXK201804001.htm [22] GUO Y, YANG C, WANG L, et al. Study on the influence of bedding density on hydraulic fracturing in shale[J]. Arabian Journal for Science and Engineering, 2018, 43(11): 6493-6508. doi: 10.1007/s13369-018-3263-6 [23] HUANG B, LIU J. Fully three-dimensional propagation model of horizontal borehole hydraulic fractures in strata under the effect of bedding planes[J]. Energy Exploration & Exploitation, 2018, 36(5): 1189-1209. [24] ZENG Y, CHENG W, ZHANG X, et al. A criterion for identifying a mixed-mode Ⅰ/Ⅱ hydraulic fracture crossing a natural fracture in the subsurface[J]. Energy Exploration & Exploitation, 2020, 38(6): 2507-2520. [25] TAHERI-SHAKIB J, GHADERI A, HOSSEINI S, et al. Debonding and coalescence in the interaction between hydraulic and natural fracture: accounting for the effect of leak-off[J]. Journal of Natural Gas Science and Engineering, 2016, 36: 454-462. doi: 10.1016/j.jngse.2016.10.056 [26] 程正华, 艾池, 张军, 等. 胶结型天然裂缝对水力压裂裂缝延伸规律的影响[J]. 新疆石油地质, 2022, 43(4): 433-439. https://www.cnki.com.cn/Article/CJFDTOTAL-XJSD202204008.htmCHENG Zhenghua, AI Chi, ZHANG Jun, et al. Influences of cemented natural fractures on propagation of hydraulic fractures[J]. Xinjiang Petroleum Geology, 2022, 43(4): 433-439. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-XJSD202204008.htm [27] WAN L, CHEN M, HOU B, et al. Experimental investigation of the effect of natural fracture size on hydraulic fracture propagation in 3D[J]. Journal of Structural Geology, 2018, 116: 1-11. doi: 10.1016/j.jsg.2018.08.006 [28] ZHOU J, ZENG Y, GUO Y, et al. Effect of natural filling fracture on the cracking process of shale Brazilian disc containing a central straight notched flaw[J]. Journal of Petroleum Science and Engineering, 2021, 196: 107993. doi: 10.1016/j.petrol.2020.107993 [29] 陈治喜, 陈勉, 黄荣樽, 等. 层状介质中水力裂缝的垂向扩展[J]. 石油大学学报(自然科学版), 1997, 21(4): 23-26. https://www.cnki.com.cn/Article/CJFDTOTAL-SYDX704.006.htmCHEN Zhixi, CHEN Mian, HUANG Rongzun, et al. Vertical growth of hydraulic fracture in layered formations[J]. Journal of the University of Petroleum, 1997, 21(4): 23-26. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-SYDX704.006.htm [30] CHANG X, GUO Y, ZHOU J, et al. Numerical and experimental investigations of the interactions between hydraulic and natural fractures in shale formations[J]. Energies, 2018, 11(10): 2541. doi: 10.3390/en11102541 [31] ZHANG Y, LIU Z, HAN B, et al. Numerical study of hydraulic fracture propagation in inherently laminated rocks accounting for bedding plane properties[J]. Journal of Petroleum Science and Engineering, 2022, 210: 109798. doi: 10.1016/j.petrol.2021.109798 [32] WANG D, SHI F, QIN H, et al. Failure patterns and mechanisms of hydraulic fracture propagation behavior in the presence of naturally cemented fractures[J]. Computer Modeling in Engineering & Sciences, 2021, 126(3): 891-914. [33] WANG H Y. Poro-elasto-plastic modeling of complex hydraulic fracture propagation: simultaneous multi-fracturing and producing well interference[J]. Acta Mechanica, 2016, 227(2): 507-525. doi: 10.1007/s00707-015-1455-7 [34] LIU C, WANG Z. Numerical simulation of hydraulic fracture propagation in shale with plastic deformation[J]. International Journal of Fracture, 2022, 238: 115-132. doi: 10.1007/s10704-022-00659-7 [35] YANG Y, LI X, JU Y. Influence mechanisms of plasticity and horizontal stress difference on the fracture propagation in plastic reservoir rocks: a 3D XFEM-based plastic fracturing model[J]. Geomechanics and Geophysics for Geo-Energy and Geo-Resources, 2022, 8(5): 145. doi: 10.1007/s40948-022-00453-8 [36] ZENG Q D, YAO J, SHAO J. Numerical study of hydraulic fracture propagation accounting for rock anisotropy[J]. Journal of Petroleum Science and Engineering, 2018, 160: 422-432. doi: 10.1016/j.petrol.2017.10.037 [37] ZHAO H, CHEN M. Extending behavior of hydraulic fracture when reaching formation interface[J]. Journal of Petroleum Science and Engineering, 2010, 74(1/2): 26-30. [38] PAPANASTASIOU P. The influence of plasticity in hydraulic fracturing[J]. International Journal of Fracture, 1997, 84(1): 61-79. doi: 10.1023/A:1007336003057 [39] PAPANASTASIOU P. The effective fracture toughness in hydraulic fracturing[J]. International Journal of Fracture, 1999, 96(2): 127-147. doi: 10.1023/A:1018676212444 [40] GRÄSLE W, PLISCHKE I. LT-A experiment: mechanical behavior of opalinus clay, data report from phase 15[R]. 2010. [41] BOONE T J, INGRAFFEA A R. A numerical procedure for simulation of hydraulically-driven fracture propagation in poroelastic media[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1990, 14(1): 27-47. doi: 10.1002/nag.1610140103 [42] BIOT M A. General theory of three-dimensional consolidation[J]. Journal of Applied Physics, 1941, 12(2): 155-164. doi: 10.1063/1.1712886 [43] TOMAR V, ZHAI J, ZHOU M. Bounds for element size in a variable stiffness cohesive finite element model[J]. International Journal for Numerical Methods in Engineering, 2004, 61(11): 1894-1920. doi: 10.1002/nme.1138 [44] CHEN Z, BUNGER A P, ZHANG X, et al. Cohesive zone finite element-based modeling of hydraulic fractures[J]. Acta Mechanica Solida Sinica, 2009, 22(5): 443-452. doi: 10.1016/S0894-9166(09)60295-0 [45] WANG H Y. Hydraulic fracture propagation in naturally fractured reservoirs: complex fracture or fracture networks[J]. Journal of Natural Gas Science and Engineering, 2019, 68: 102911. doi: 10.1016/j.jngse.2019.102911 [46] GEERTSMA J, DE KLERK F. A rapid method of predicting width and extent of hydraulically induced fractures[J]. Journal of Petroleum Technology, 1969, 21(12): 1571-1581. doi: 10.2118/2458-PA [47] XU W, ZHAO J, RAHMAN SS, et al. A comprehensive model of a hydraulic fracture interacting with a natural fracture: analytical and numerical solution[J]. Rock Mechanics and Rock Engineering, 2019, 52(4): 1095-1113. doi: 10.1007/s00603-018-1608-9 [48] SANTILLÁN D, JUANES R, CUETO-FELGUEROSO L. Phase field model of fluid-driven fracture in elastic media: immersed-fracture formulation and validation with analytical solutions[J]. Journal of Geophysical Research: Solid Earth, 2017, 122(4): 2565-2589. doi: 10.1002/2016JB013572 [49] BLANTON T L. An experimental study of interaction between hydraulically induced and pre-existing fractures[C]//SPE Unconventional Gas Recovery Symposium. Pittsburgh, Pennsylvania, 1982. [50] SUN T, ZENG Q, XING H. A quantitative model to predict hydraulic fracture propagating across cemented natural fracture[J]. Journal of Petroleum Science and Engineering, 2022, 208: 109595. doi: 10.1016/j.petrol.2021.109595 [51] LIU C, ZHANG J N, YU H, et al. New insights of natural fractures growth and stimulation optimization based on a three-dimensional cohesive zone model[J]. Journal of Natural Gas Science and Engineering, 2020, 76: 103165. doi: 10.1016/j.jngse.2020.103165 [52] PARISIO F, SAMAT S, LALOUI L. Constitutive analysis of shale: a coupled damage plasticity approach[J]. International Journal of Solids and Structures, 2015, 75: 88-98. [53] SONG Y B, LU W Y, HE C C, et al. Numerical simulation of the influence of natural fractures on hydraulic fracture propagation[J]. Geofluids, 2020, 2020: 8878548. [54] LI P, DONG Y, WANG S, et al. Numerical modelling of interaction between hydraulic fractures and natural fractures by using the extended finite element method[J]. Advances in Civil Engineering, 2020, 2020: 8848900. -
图(12) / 表(2)
计量
- 文章访问数: 435
- HTML全文浏览量: 161
- PDF下载量: 69
- 被引次数: 0
渝公网安备50010802005915号