Research on Partial Slip Contact Behaviors Under Temperature Effects
-
摘要:
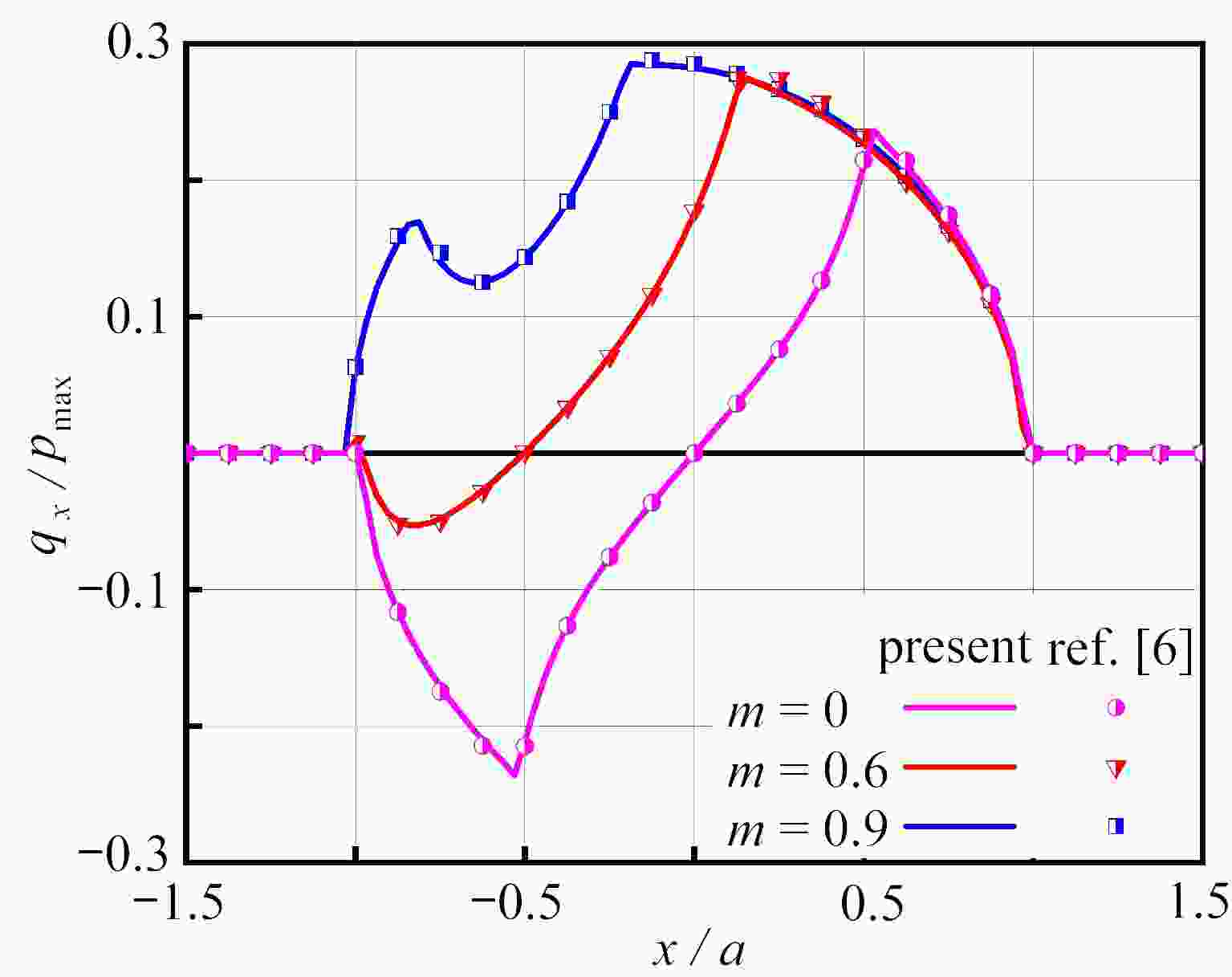
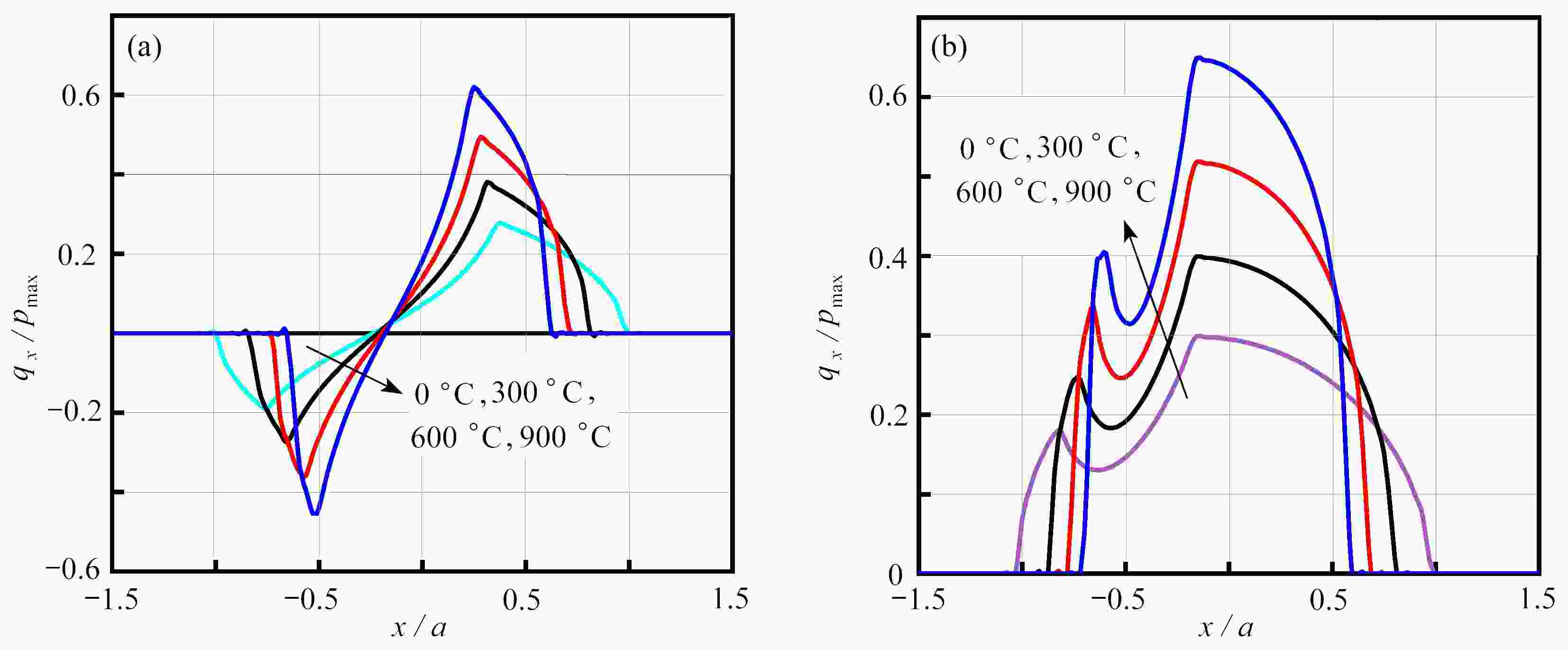
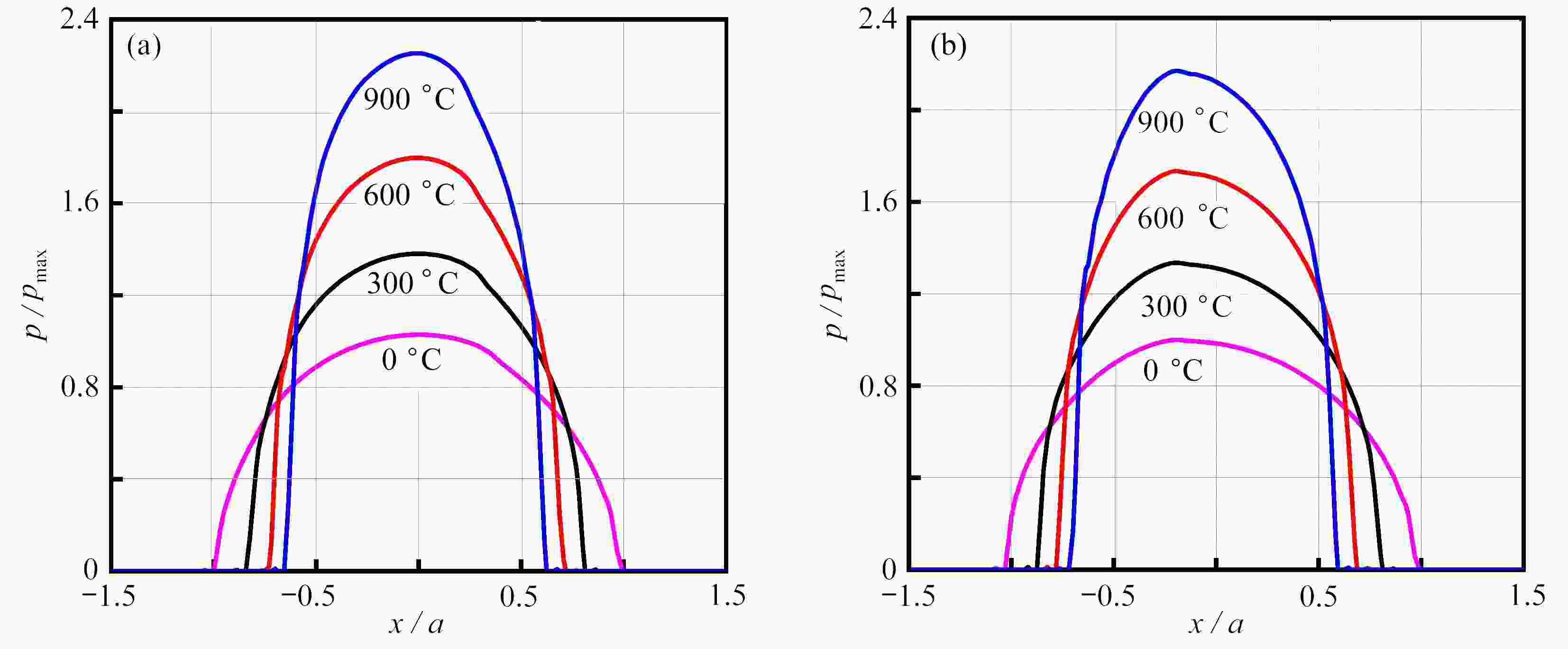
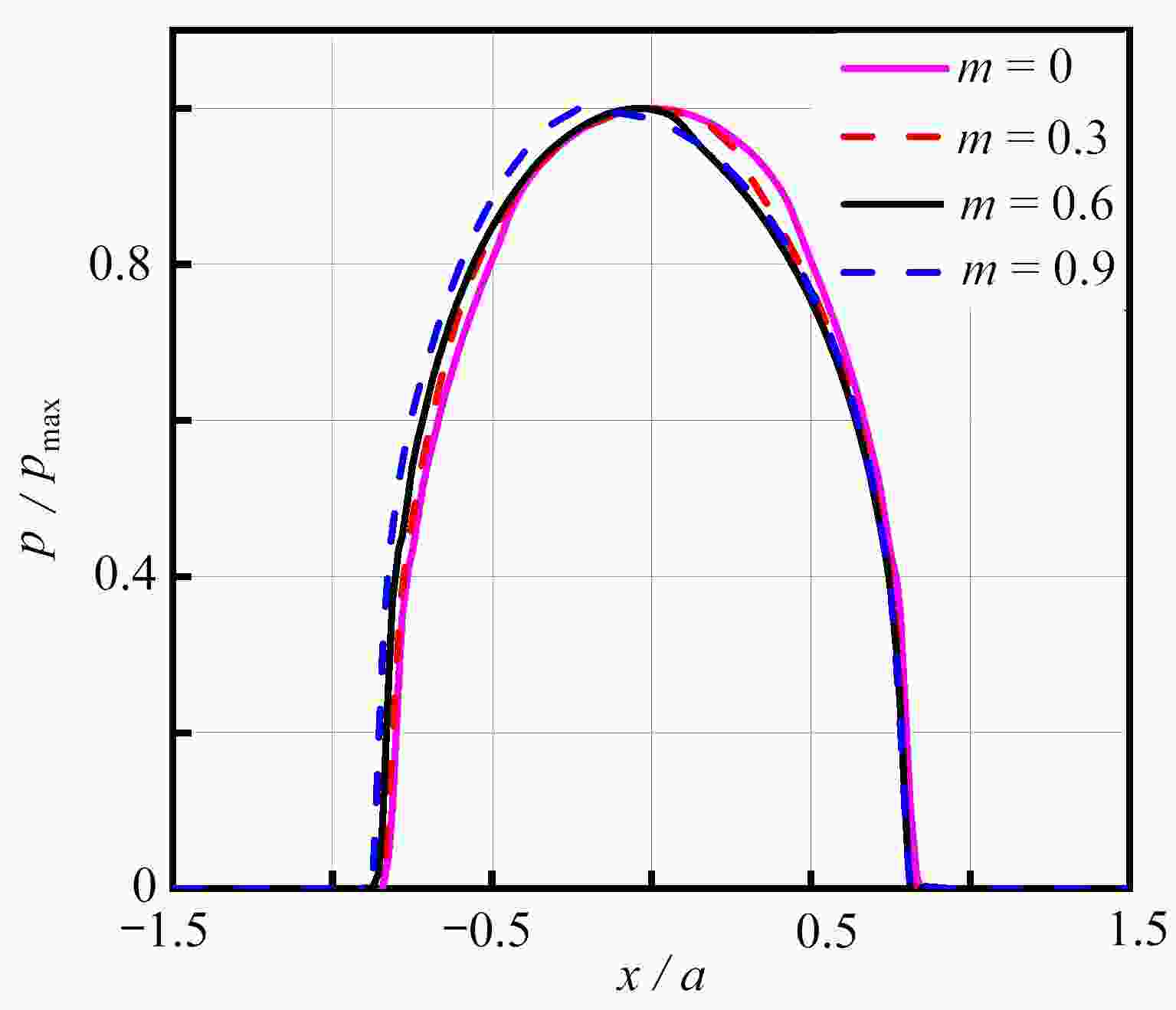
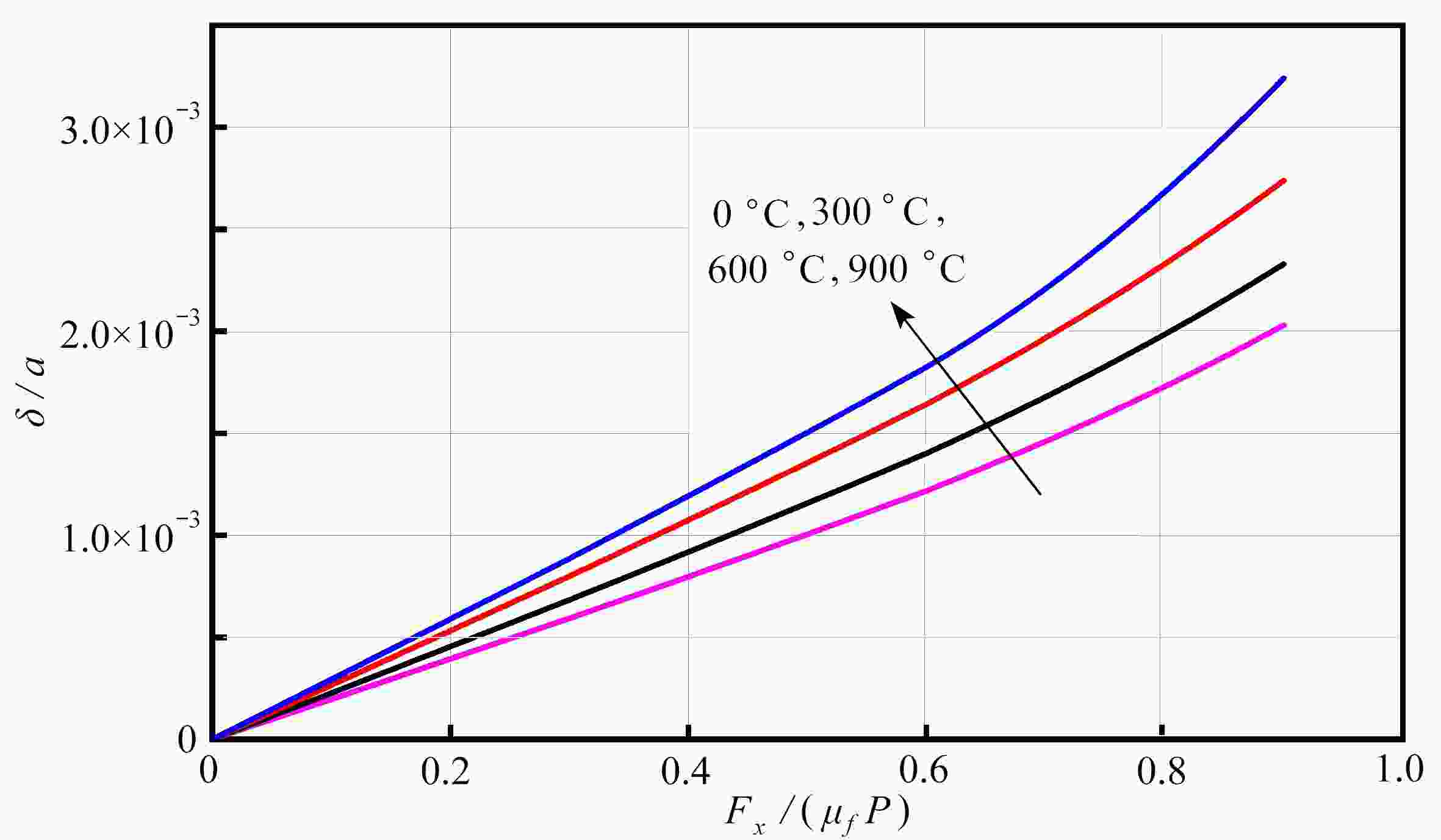
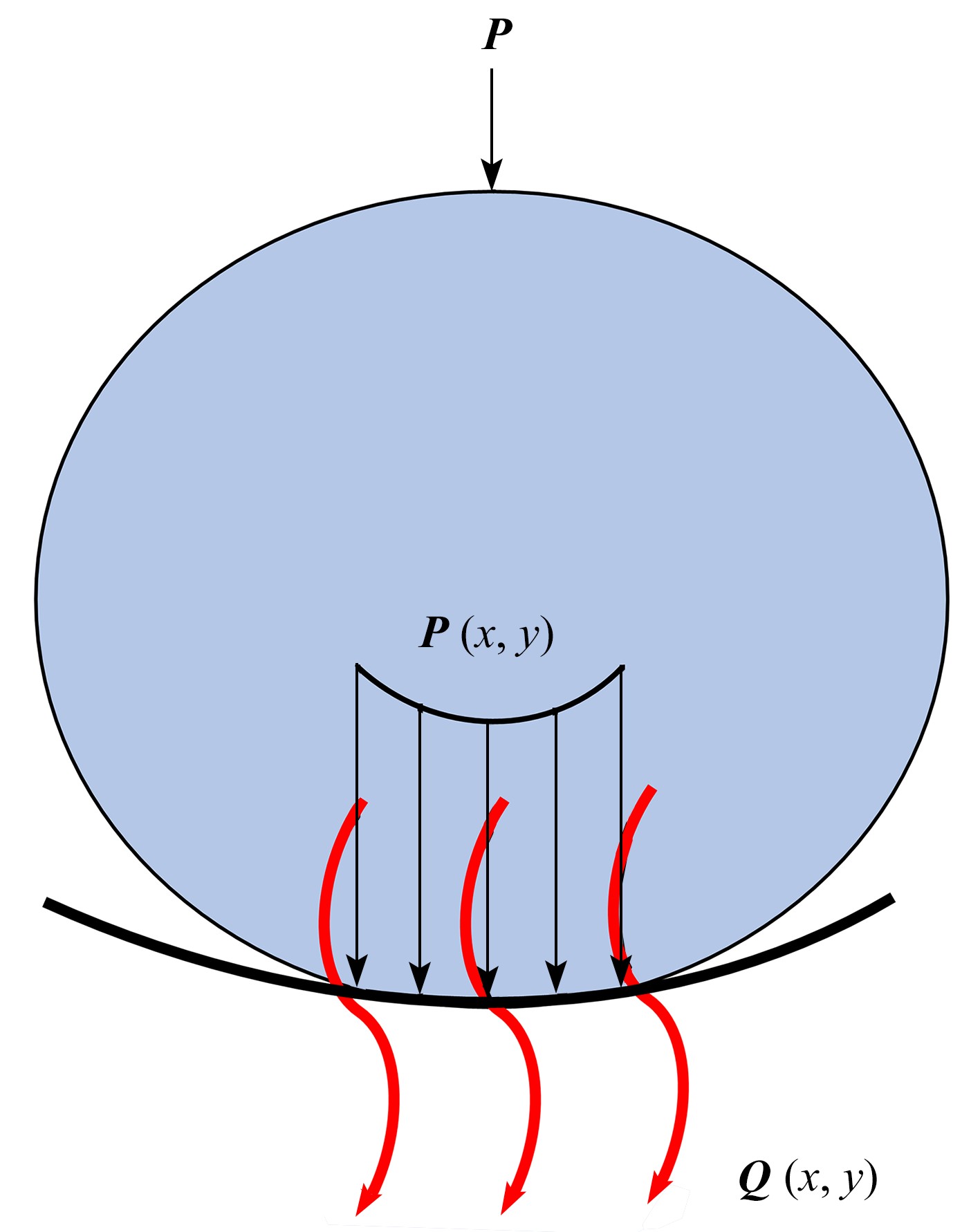
针对不同温度装配件间接触界面的局部滑移问题,建立了三维稳态热弹性局部滑移接触的半解析求解模型。基于热弹性理论与热传导方程,构建了半空间受热流载荷和力载荷作用下的频响函数并建立了相应的影响系数矩阵。借助离散卷积-快速Fourier变换等数学工具,实现了针对高温压头与热弹性半空间局部滑移接触问题的高效求解。接触界面间的热量传递满足Fourier热传导定律,并且黏/滑状态由Coulomb定律确定。基于该半解析模型分析了不同荷载及温差对表面法向压力分布、摩擦力分布、刚体位移及接触区黏/滑演化行为的影响。研究结果表明,当法向荷载和切向荷载一定时,温差的上升会导致接触区域的减小,引起接触面法向压力及摩擦力的峰值增大,并且会显著影响黏着区与滑移区的分布情况。
-
关键词:
- 热弹性接触 /
- 局部滑移 /
- 半解析法 /
- 离散卷积-快速Fourier变换 /
- 共轭梯度法
Abstract:Aimed at the partial slip problem of contact interface between assemblies at different temperatures, a semi-analytical model for 3D steady-state thermoelastic partial slip contact was established. Based on the thermoelastic theory and the heat conduction equation, the frequency response functions of the half space under heat flux and force load were given, and the corresponding influence coefficients were established. With the discrete convolution and fast Fourier transform (DC-FFT), the partial contact between the rigid high-temperature indenter and the thermoelastic half space was efficiently solved. The heat conduction behavior was assumed to follow Fourier’s law, and the stick/slip state on the contact interface was determined under Coulomb’s law. Based on this semi-analytical model, the effects of external loads and temperature differences on the surface pressure distribution, the friction distribution, the rigid body displacement, and the stick/slip evolution behaviors, were analyzed in detail. The numerical results show that, the increase of the temperature difference will lead to a decrease of the contact area, result in an increase of the peak values of the normal pressure and the friction, and significantly influence the regions of the stick zone and the slip zone.
-
表 1 模型参数
Table 1. Model parameters
model parameter value normal load $P/{\rm{N}}$ 20 tangential load ${F_x}/{\rm{N}}$ $\left( {0 \sim 0.9} \right) \times {\mu _f}P$ tangential load ${F_y}/{\rm{N}}$ 0 friction coefficient ${\mu _f}$ 0.3 ball radius $R/{\rm{mm}}$ 18 maximum Hertzian pressure ${p_{\max } }/{\rm{MPa}}$ 907 elastic half space modulus $E/{\rm{GPa}}$ 210 Poisson’s ratio of elastic half space $\nu $ 0.3 thermal expansion in coefficient elastic half space K $2.0 \times {10^{ - 5}}$ elastic half space heat transfer coefficien $Q/({{\text{W}}/ {({\text{m}} \cdot {\text{K}})}}$) 60 -
[1] JOHNSON K L. Contact Mechanics[M]. Cambridge, UK: Cambridge University Press, 1985. [2] LAKKARAJU R K, BOBARU F, ROHDE S L. Optimization of multilayer wear-resistant thin filmsusing finite element analysis on stiff and compliantsubstrates[J]. Journal of Vacuum Science & Technology A, 2006, 24(1): 146-155. [3] 潘先云, 余江鸿, 周枫林. 非齐次弹性力学问题双互易边界元方法研究[J]. 应用数学和力学, 2022, 43(9): 1004-1015PAN Xianyun, YU Jianghong, ZHOU Fenglin. Research on the dual reciprocity boundary element method for non-homogeneous elasticity problems[J]. Applied Mathematics and Mechanics, 2022, 43(9): 1004-1015.(in Chinese) [4] 王晋宝, 童焯煜, 何博, 等. 考虑应力沿深度变化的饱和软黏土一维固结半解析解[J]. 应用数学和力学, 2019, 40(10): 1099-1108WANG Jinbao, TONG Zhuoyu, HE Bo, et al. A semi-analytical 1D consolidation solution of saturated soft clay with changing stresses along the depth[J]. Applied Mathematics and Mechanics, 2019, 40(10): 1099-1108.(in Chinese) [5] KUPCHYTSR, BAJKOWSKIA. Elastic coating within homogeneous inter layer under the action of normal and tangential forces[J]. Materials Science, 2014, 49(5): 650-659. doi: 10.1007/s11003-014-9659-x [6] WANG Z J, WANG W Z, WANG H, et al. Partial slip contact analysis on three-dimensional elastic layered half space[J]. Journal of Tribology, 2010, 132(2): 021403. doi: 10.1115/1.4001011 [7] CHEN W, WANG Q J. A numerical static friction model for spherical contacts of rough surfaces, influence of load, material, and roughness[J]. Journal of Tribology, 2009, 131(2): 021402. doi: 10.1115/1.3063814 [8] WANG Z J, JIN X, LIU S B, et al. A new fast method for solving contact plasticity and its application in analyzing elasto-plastic partial slip[J]. Mechanics of Materials, 2013, 60: 18-35. doi: 10.1016/j.mechmat.2013.01.001 [9] WANG Z J, MENG F M, XIAO K, et al. Numerical analysis of partial slip contact under a tangential force anda twisting moment[J]. Proceedings of the Institution of Mechanical Engineers (Part J) : Journal of Engineering Tribology, 2011, 225(2): 72-83. doi: 10.1177/2041305X10392125 [10] 向金兰, 刘娟, 沈火明. 热源影响下的涂层板滑动接触问题研究[J]. 机械科学与技术, 2021, 40(2): 165-167 doi: 10.13433/j.cnki.1003-8728.20200040XIANG Jinlan, LIU Juan, SHEN Huoming. Study on sliding contact problem of coated plate considering heat source[J]. Mechanical Science and Technology, 2021, 40(2): 165-167.(in Chinese) doi: 10.13433/j.cnki.1003-8728.20200040 [11] 李玲, 田海飞, 云强强, 等. 微动接触中分形粗糙表面的温升分布研究[J]. 表面技术, 2019, 48(6): 238-244 doi: 10.16490/j.cnki.issn.1001-3660.2019.06.028LI Ling, TIAN Haifei, YUN Qiangqiang, et al. Temperature rise distribution of fractal rough surface in fretting contact[J]. Surface Technology, 2019, 48(6): 238-244.(in Chinese) doi: 10.16490/j.cnki.issn.1001-3660.2019.06.028 [12] ZHANG X, SHEN H M, LIU J, et al. An efficient numerical model for predicting the torsional fretting wear considering real rough surface[J]. Wear, 2015, 344/345: 32-45. doi: 10.1016/j.wear.2015.10.019 [13] ZHANG X, WANG Z J, SHEN H M, et al. Dynamic contact in multiferroic energy conversion[J]. International Journal of Solids and Structures, 2018, 143: 84-102. doi: 10.1016/j.ijsolstr.2018.02.034 [14] LIU S, WANG Q. Studying contact stress fields caused by surface tractions with a discrete convolution and fast Fourier transform algorithm[J]. Journal of Tribology, 2002, 124(1): 36-45. doi: 10.1115/1.1401017 [15] POLONSKY I A, KEER L M. A numerical method for solving rough contact problems based on the multi-level multi-summation and conjugate gradient techniques[J]. Wear, 1999, 231(2): 206-219. doi: 10.1016/S0043-1648(99)00113-1 [16] 王宇星. 三维偶应力弹性体接触力学问题的半解析法建模研究[D]. 博士学位论文. 成都: 西南交通大学, 2021.WANG Yuxing. Semi-analytical modeling of three-dimensional contact problem within the framework[D]. PhD Thesis. Chengdu: Southwest Jiaotong University, 2021. (in Chinese) -




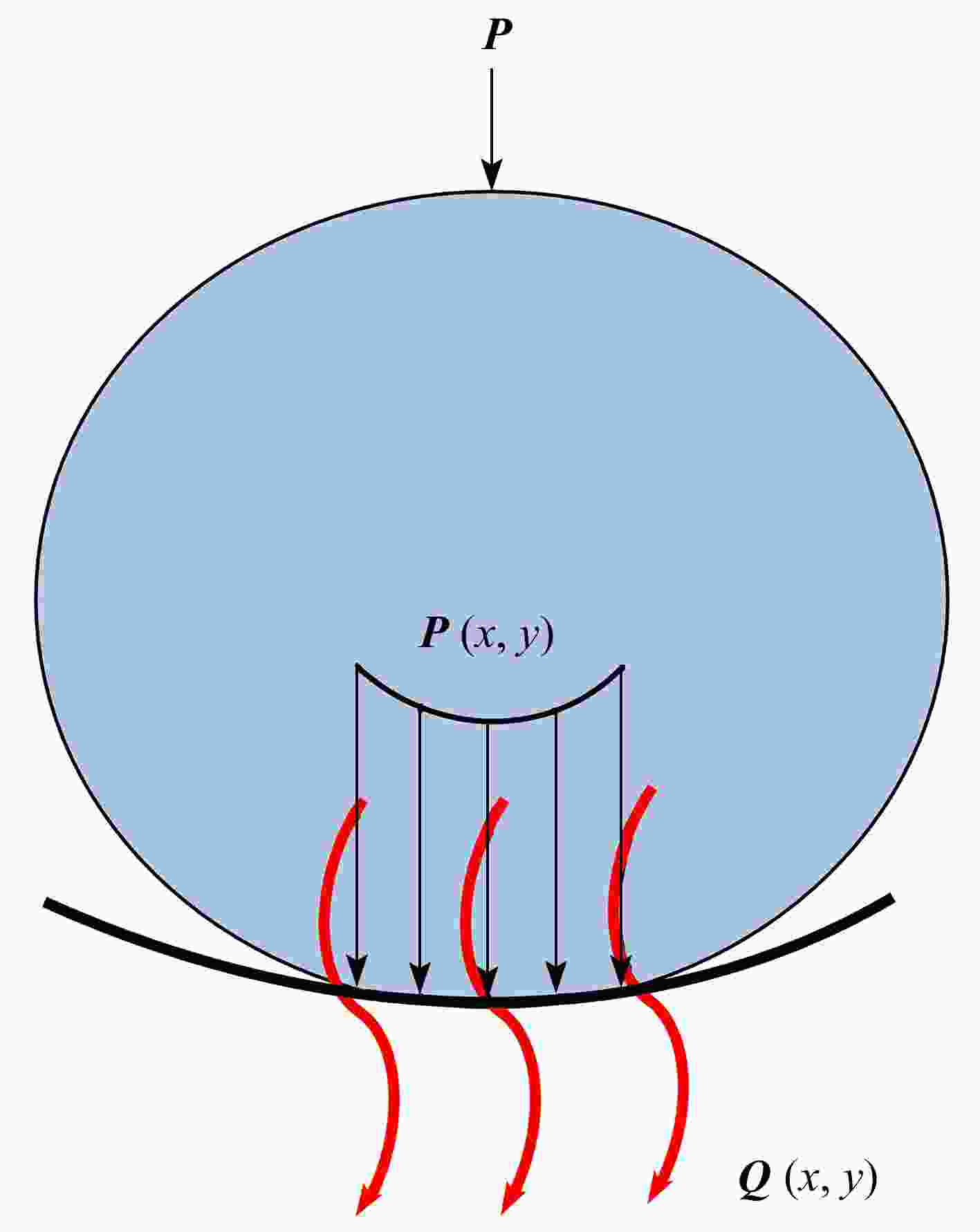
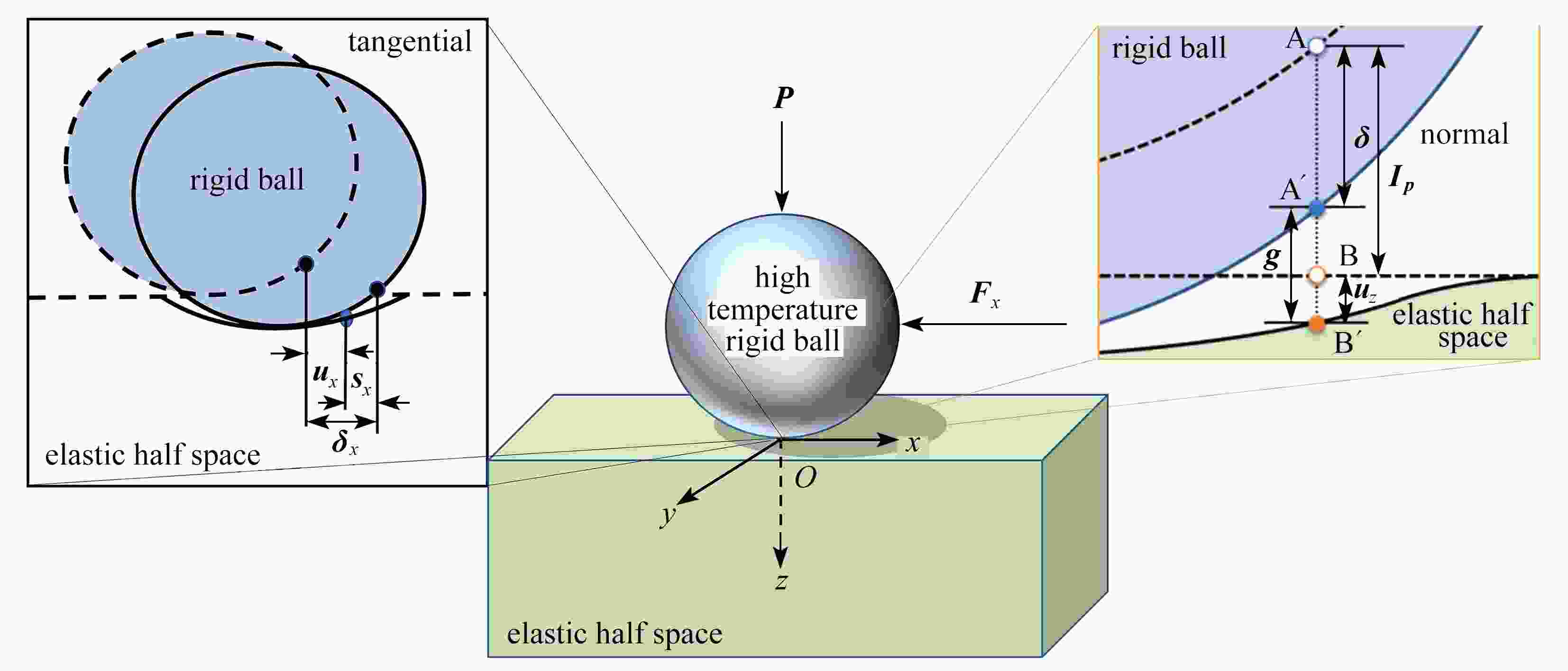
 下载:
下载:
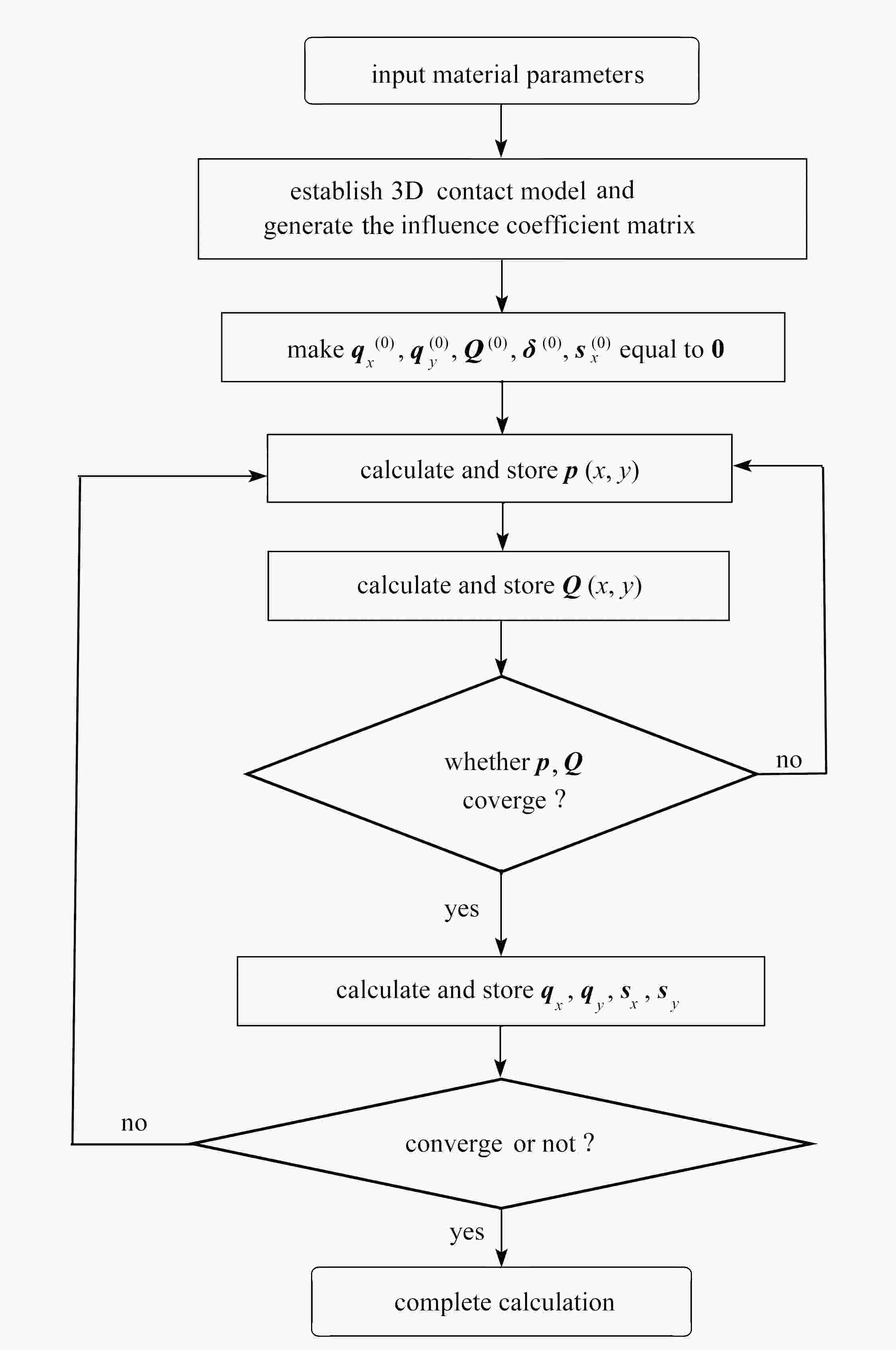
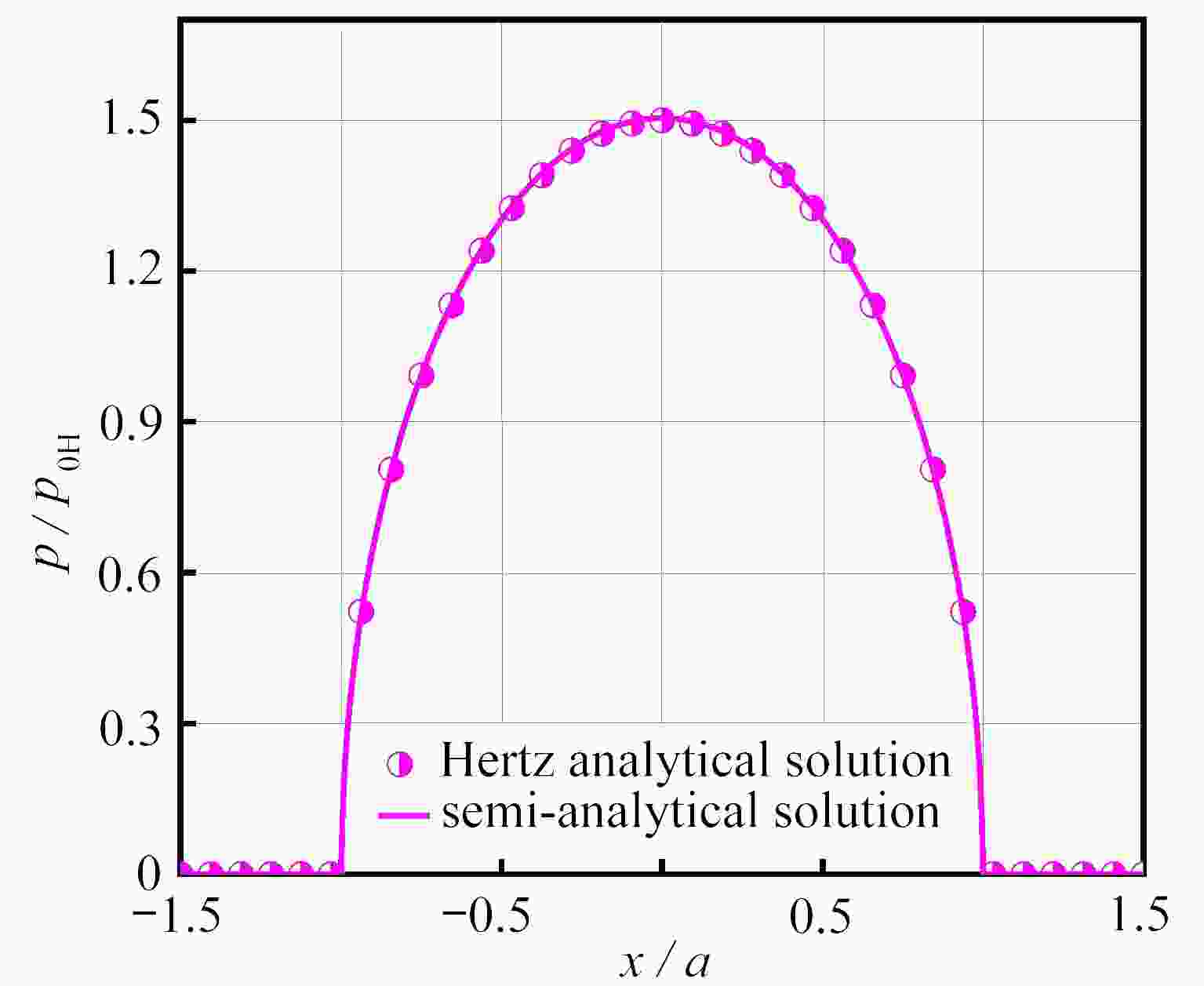












 渝公网安备50010802005915号
渝公网安备50010802005915号