微分求积法求解悬臂L梁固有振动特性研究

李智超; 郝育新

doi:10.21656/1000-0887.430382

微分求积法求解悬臂L梁固有振动特性研究

doi: 10.21656/1000-0887.430382

李智超,
郝育新^,

北京信息科技大学机电工程学院，北京 100192

基金项目:

国家自然科学基金项目 11872127

详细信息

作者简介:
李智超(1998—)，男，硕士生(E-mail: lzc19980510@163.com)

通讯作者:
郝育新(1972—)，男，教授(通讯作者. E-mail: bimhao@163.com)

中图分类号: O31; O32
计量
- 文章访问数: 559
- HTML全文浏览量: 186
- PDF下载量: 87
- 被引次数: 0
出版历程
- 收稿日期: 2022-11-29
- 修回日期: 2023-02-17
- 刊出日期: 2023-05-01

Study on Natural Vibration Characteristics of L-Shaped Cantilever Beams With the Differential Quadrature Method

LI Zhichao,
HAO Yuxin^,

Mechanical Electrical Engineering School, Beijing Information Science and Technology University, Beijing 100192, P.R.China

摘要

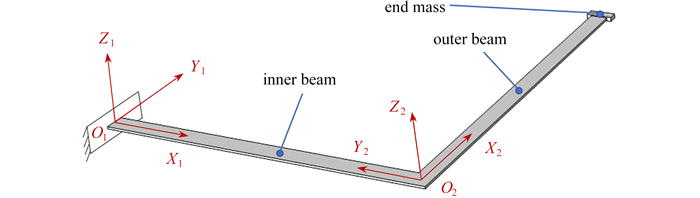
摘要: 悬臂L梁结构由于具有柔性大、可设计性强、空间利用充分，振动过程中变形方式多样等独特优势而受到了广泛的关注与研究. 该文提出了一种基于微分求积法求解末端附加质量块的矩形等截面均质悬臂细长L梁的各阶固有频率和模态的方法. 在双坐标系下，基于Euler-Bernoulli梁理论建立了悬臂L梁的动力学方程，然后通过选取Chebyshev多项式的根作为节点坐标、选取Lagrange插值基函数、求解各阶权系数、处理边界条件等步骤，最终利用求解矩阵广义特征值问题的方法求得结构各阶固有频率及模态. 在边界条件的处理上，直接将边界条件施加于边界点上，通过对比研究验证了该文固有频率理论解的正确性. 最后分析了末端质量、内外梁的长度比、宽度、厚度对各阶固有振动特性的影响. 该方法可以进一步应用推广到相关结构振动的研究中.
- 微分求积法 /
- 悬臂L梁 /
- 固有振动特性
Abstract: The L-shaped cantilever beam structure has many unique advantages such as large flexibility, strong designability, full utilization of space and various deformation modes during vibration, and is widely regarded and studied. A differential quadrature method was proposed to solve the natural frequencies and modes of rectangular-section homogeneous slender L-shaped cantilever beams with additional end masses. In the double coordinate systems, the dynamic equations for the L-shaped cantilever beam based on the Euler-Bernoulli beam theory were established. With selected roots of the Chebyshev polynomial as the node coordinates, the Lagrange interpolation basis function was employed, the weight coefficients of each order were solved, and the boundary conditions were considered, to obtain the natural frequencies and modes of all orders of the structure through resolution of the generalized matrix eigenvalue problem. The theoretical solution of the natural frequencies was verified in comparison with the previous theoretical results and the finite element results. Finally, the effects of the end mass, the length ratio, the width and the thickness of the inner and outer beams on the natural vibration characteristics of all orders were discussed. This method can be further applied to the study of related structural vibrations.
- differential quadrature method /
- L-shaped cantilever beam /
- natural vibration characteristic

HTML全文

图 1 悬臂L梁示意图

Figure 1. Schematic diagram of the L-shaped cantilever beam

下载: 全尺寸图片幻灯片

图 2 结构前五阶模态图

Figure 2. Diagram of the first five modes of the structure

下载: 全尺寸图片幻灯片

图 3 内、外梁长度比对结构各阶固有频率的影响

Figure 3. The effect of the length ratio of the outer beam to the inner beam on natural frequencies of the structure

下载: 全尺寸图片幻灯片

图 4 梁宽度对结构各阶固有频率的影响

Figure 4. The effect of the beam width on natural frequencies of the structure

下载: 全尺寸图片幻灯片

图 5 梁厚度对结构各阶固有频率的影响

Figure 5. The effect of the beam thickness on natural frequencies of the structure

下载: 全尺寸图片幻灯片

图 6 末端质量对结构各阶固有频率的影响

Figure 6. The effect of the end mass on natural frequencies of the structure

下载: 全尺寸图片幻灯片

表 1 悬臂L梁的几何与材料参数表

Table 1. Geometric and material parameters of the L-shaped cantilever beam

parameter	value^[20]	parameter	value^[20]
length l₁/m, l₂/m	0.75, 0.75	elasticity modulus E/Pa	7×10¹⁰
width b/m	0.04	polar moment of inertia I_p/m⁴	1.535×10^-9
thickness h/m	0.005	end mass m/kg	0.5
density ρ/(kg/m³)	2 700	Poisson’s ratio υ	0.3

下载: 导出CSV

表 2 不同节点数下的结构前五阶固有频率表(单位：Hz)

Table 2. First five-order natural frequencies of the structure with different number of nodes (unit: Hz)

node	mode
node	1	2	3	4	5
N_1，2=9	1.377 7	5.514 0	27.671 8	42.532 3	94.087 5
N_1，2=10	1.377 7	5.513 6	27.661 4	42.342 1	93.287 7
N_1，2=11	1.377 7	5.513 6	27.659 9	42.259 0	93.070 6
N_1，2=12	1.377 7	5.513 6	27.660 2	42.261 4	93.072 7
N_1，2=13	1.377 7	5.513 6	27.660 2	42.263 6	93.088 2
N_1，2=14	1.377 7	5.513 6	27.660 2	42.263 5	93.089 0
N_1，2=15	1.377 7	5.513 6	27.660 2	42.263 4	93.088 4

下载: 导出CSV

表 3 结构前五阶固有频率对比表(单位：Hz)

Table 3. Comparison of the structure's first five-order natural frequencies (unit: Hz)

mode	1	2	3	4	5
present N_1，2=13	1.377 7	5.513 6	27.660 2	42.263 6	93.088 2
ref. [20] (error δ/%)	1.377 2(0.04)	5.531 6(-0.33)	27.761 9(-0.37)	42.456 2(-0.45)	93.500 7(-0.44)
PATRAN (error δ/%)	1.370 1(0.55)	5.486 1(0.50)	27.582 0(0.28)	41.738 0(1.26)	92.665 0(0.46)
COMSOL (error δ/%)	1.405 3(-1.96)	5.554 0(-0.73)	27.779 0(-0.43)	41.556 0(1.70)	92.551 0(0.58)

下载: 导出CSV

参考文献(20)

[1]	CHEN L Q, JIANG W A, PANYAM M, et al. A broadband internally resonant vibratory energy harvester[J]. Journal of Vibration and Acoustics, 2016, 138(6): 061107. http://www.istic.ac.cn/suoguan/detailed.htm?dbname=xw_qk&wid=0220161200680273
[2]	ERTURK A, RENNO J M, INMAN D J. Modeling of piezoelectric energy harvesting from an L-shaped beam-mass structure with an application to UAVs[J]. Journal of Intelligent Material Systems and Structures, 2009, 20(5): 529-544. doi: 10.1177/1045389X08098096
[3]	HARNE R L, SUN A, WANG K W. Leveraging nonlinear saturation-based phenomena in an L-shaped vibration energy harvesting system[J]. Journal of Sound and Vibration, 2016, 363: 517-531. doi: 10.1016/j.jsv.2015.11.017
[4]	LI H, SUN H, SONG B, et al. Nonlinear dynamic response of an L-shaped beam-mass piezoelectric energy harvester[J]. Journal of Sound and Vibration, 2021, 499: 116004. doi: 10.1016/j.jsv.2021.116004
[5]	KIM I H, JANG S J, JUNG H J. Design and experimental study of an L shape piezoelectric energy harvester[J]. Shock and Vibration, 2017, 2017: 8523218.
[6]	BERT C W, JANG S K, STRIZ A G. Two new approximate methods for analyzing free vibration of structural components[J]. AIAA Journal, 1988, 26(5): 612-618. doi: 10.2514/3.9941
[7]	王冬梅, 张伟, 刘寅立. 微分求积法在工程结构动力学中的应用研究[J]. 天津科技大学学报, 2018, 33(1): 71-78. https://www.cnki.com.cn/Article/CJFDTOTAL-TQYX201801015.htm WANG Dongmei, ZHANG Wei, LIU Yinli. Application of differential quadrature method in engineering structural dynamics[J]. Journal of Tianjin University of Science & Technology, 2018, 33(1): 71-78. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-TQYX201801015.htm
[8]	吴鹦泽, 王冬梅. 微分求积法在悬臂梁结构非线性动力学中的应用研究[J]. 力学研究, 2018, 7: 1-13. WU Yingze, WAND Dongmei. Application of differential quadrature method to nonlinear dynamics of cantilever beam structures[J]. International Journal of Mechanics Research, 2018, 7: 1-13. (in Chinese)
[9]	WANG X. Differential Quadrature and Differential Quadrature Based Element Methods: Theory and Applications[M]. Oxford: Butterworth-Heinemann, 2015.
[10]	TORNABENE F, FANTUZZI N, UBERTINI F. Strong formulation finite element method based on differential quadrature: a survey[J]. Applied Mechanics Reviews, 2015, 67(2): 020801. doi: 10.1115/1.4028859
[11]	夏雨, 葛仁余, 王静平, 等. 变截面Euler-Bernoulli梁稳态谐振动的微分求积法研究[J]. 安徽工程大学学报, 2021, 36(4): 56-63. https://www.cnki.com.cn/Article/CJFDTOTAL-AHJD202104011.htm XIA Yu, GE Renyu, WANG Jingping, et al. Differential quadrature method for steady-state harmonic vibration of beams with variable cross-section[J]. Journal of Anhui Polytechnic University, 2021, 36(4): 56-63. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-AHJD202104011.htm
[12]	葛仁余, 张佳宸, 刘凡, 等. 微分求积法在计算功能梯度Timoshenko梁临界荷载中的应用研究[J]. 应用力学学报, 2020, 37(6): 2634-2641. https://www.cnki.com.cn/Article/CJFDTOTAL-YYLX202006043.htm GE Renyu, ZHANG Jiachen, LIU Fan, et al. Calculation of critical load for functionally graded Timoshenko beam using differential quadrature method[J]. Chinese Journal of Applied Mechanics, 2020, 37(6): 2634-2641. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YYLX202006043.htm
[13]	KHAKPOUR M, BAZARGAN-LARI Y, ZAHEDINEJAD P, et al. Vibrations evaluation of functionally graded porous beams in thermal surroundings by generalized differential quadrature method[J]. Shock and Vibration, 2022, 2022(4): 8516971.
[14]	PENG L, WANG Y. Differential quadrature method for vibration analysis of prestressed beams[J]. E3S Web of Conferences, 2021, 237: 03029. doi: 10.1051/e3sconf/202123703029
[15]	SZEKRÉNYES A. Differential quadrature solution for composite flat plates with delamination using higher-order layerwise models[J]. International Journal of Solids and Structures, 2022, 248: 111621.
[16]	LIU H, ZHAO Y, PISHBIN M, et al. A comprehensive mathematical simulation of the composite size-dependent rotary 3D microsystem via two-dimensional generalized differential quadrature method[J]. Engineering With Computers, 2022, 38(5): 4181-4196. doi: 10.1007/s00366-021-01419-2
[17]	AL-FURJAN M S H, HABIBI M, SHAN L, et al. On the vibrations of the imperfect sandwich higher-order disk with a lactic core using generalize differential quadrature method[J]. Composite Structures, 2021, 257: 113150. http://www.sciencedirect.com/science/article/pii/S0263822320330762
[18]	刘旭, 姚林泉. 热环境中旋转功能梯度纳米环板的振动分析[J]. 应用数学和力学, 2020, 41(11): 1224-1236. doi: 10.21656/1000-0887.410090 LIU Xu, YAO Linquan. Vibration analysis of rotating functionally gradient nano annular plates in thermal environment[J]. Applied Mathematics and Mechanics, 2020, 41(11): 1224-1236. (in Chinese) doi: 10.21656/1000-0887.410090
[19]	葛仁余, 张佳宸, 马国强, 等. 微分求积法分析平面接头应力奇异性[J]. 应用数学和力学, 2022, 43(4): 382-391. doi: 10.21656/1000-0887.420218 GE Renyu, ZHANG Jiachen, MA Guoqiang, et al. Analysis on stress singularity of plane joints with the differential quadrature method[J]. Applied Mathematics and Mechanics, 2022, 43(4): 382-391. (in Chinese) doi: 10.21656/1000-0887.420218
[20]	CAO Y, CAO D, HE G, et al. Vibration analysis and distributed piezoelectric energy harvester design for the L-shaped beam[J]. European Journal of Mechanics A: Solids, 2021, 87: 104214. http://www.sciencedirect.com/science/article/pii/S0997753821000103