弹性地基上多孔二维功能梯度材料微梁自由振动研究

赵英治; 唐怀平; 赖泽东; 章家杰

doi:10.21656/1000-0887.440050

弹性地基上多孔二维功能梯度材料微梁自由振动研究

doi: 10.21656/1000-0887.440050

西南交通大学力学与航空航天学院, 成都 610031

基金项目:

国家自然科学基金项目 51778548

详细信息

作者简介:
赵英治(1999—)，男，硕士生(E-mail: 964003289@qq.com)

通讯作者:
唐怀平(1967—)，男，副教授，博士(通讯作者. E-mail: thp-vib@163.com)

中图分类号: O342
计量
- 文章访问数: 382
- HTML全文浏览量: 117
- PDF下载量: 52
- 被引次数: 0
出版历程
- 收稿日期: 2023-02-27
- 修回日期: 2023-04-03
- 刊出日期: 2023-11-01

Free Vibration Analysis of Porous 2D Functionally Graded Material Microbeams on Winkler's Foundation

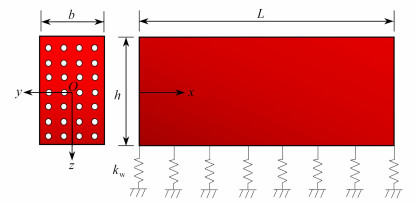
School of Mechanics and Aerospace Engineering, Southwest Jiaotong University, Chengdu 610031, P.R.China

摘要

摘要: 基于修正偶应力和Timoshenko梁理论，利用Hamilton变分原理推导了Winkler弹性地基上多孔二维功能梯度材料(2D-FGM)微梁的振动控制方程, 采用微分求积法获得固支-固支(C-C)、简支-简支(S-S)边界条件下微梁的振动频率和基本振型, 对刚度矩阵进行数学处理后极大地提高了计算效率, 将该文模型退化为宏观和微观二维功能梯度模型且与已有文献对比验证其正确性.算例结果表明：该文数学模型适用于不同类型的二维材料分布；微梁的无量纲振动频率随着Winkler弹性地基模量的增大而增大; 在一定Winkler弹性地基模量下, 微梁的无量纲振动频率随着功能梯度指数、轴向功能梯度指数、孔隙率的增大而减小.材料变化对振动模态的影响随着振动模态阶数的增加而增加.同样参数下, 孔隙均匀分布时梁频率略小于孔隙线性分布的情况.
- 修正偶应力理论 /
- 孔隙率 /
- Winkler弹性地基 /
- 二维功能梯度材料 /
- 微分求积法
Abstract: Based on the modified couple stress theory and the Timoshenko beam theory, the governing equations for free vibration of porous 2D functional graded material (FGM) on Winkler's foundation were derived under Hamilton's principle. The differential quadrature method was used to obtain the numerical solutions of the vibration frequencies and fundamental mode shapes of microbeams with both ends clamped (C-C) and simply supported (S-S). The improved stiffness matrix was used to greatly improve the calculation efficiency. The proposed model was degenerated to the macro and micro 2D-FGM models, which were compared with those in previous literatures for validation. The results show that, the present mathematical model is suitable for different types of 2D material distributions. The dimensionless frequencies increase with the dimensionless elastic modulus of Winkler's foundation. Under a certain dimensionless elastic foundation modulus, the dimensionless frequencies decrease with the functionally graded index, the axial functionally graded index and the porosity. The effect of the material variation on the mode shape increases with the mode number. For the same parameter, the dimensionless frequencies of the beam with uniform porosity distribution are slightly lower than those with linear porosity distribution.
- modified couple stress theory /
- porosity /
- Winkler's foundation /
- 2D functionally graded material /
- differential quadrature method

HTML全文

图 1 Winkler弹性地基上多孔2D-FGM微梁几何尺寸及坐标系

Figure 1. The geometry and coordinates of a porous 2D functionally graded microbeam on Winkler's foundation

下载: 全尺寸图片幻灯片

图 2 孔隙分布模式

Figure 2. Porosity distribution patterns

下载: 全尺寸图片幻灯片

图 3 孔隙率对前三阶无量纲频率的影响(P_z=2, L/h=5, λ=3×10^-6)

Figure 3. Effects of the porosity on the 1st 3 dimensionless frequencies (P_z=2, L/h=5, λ=3×10^-6)

下载: 全尺寸图片幻灯片

图 4 不同理论下功能梯度指数对前二阶无量纲频率的影响(b=2h, L=5h, θ=0.2, λ=3×10^-6)

Figure 4. Effects of the functionally graded index on the 1st 2 dimensionless frequencies under different theories (b=2h, L=5h, θ=0.2, λ=3×10^-6)

下载: 全尺寸图片幻灯片

图 5 功能梯度指数P_z和P_x共同对弹性地基多孔2D-FGM微梁一阶无量纲频率的影响(θ=0.2, λ=3×10^-6)

Figure 5. The effects of P_z and P_x on the dimensionless fundamental frequency of porous 2D-FGM microbeams(θ=0.2, λ=3×10^-6)

下载: 全尺寸图片幻灯片

图 6 跨厚比和功能梯度指数共同对弹性地基多孔2D-FGM梁一阶无量纲频率的影响(孔隙均匀分布, θ=0.2, λ=3×10^-6)

Figure 6. The effects of the span-to-depth ratio and the functionally graded index on the dimensionless fundamental frequencies of porous 2D-FGM microbeams(uniform porosity distribution, θ=0.2, λ=3×10^-6)

下载: 全尺寸图片幻灯片

图 7 弹性地基上多孔2D-FGM微梁的前三阶振动模态(C-C边界)

Figure 7. The 1st 3 mode shape of the porous 2D-FGM microbeam on Winkler's foundation (C-C boundary condition)

下载: 全尺寸图片幻灯片

图 8 弹性地基上多孔2D-FGM微梁的前三阶振动模态(S-S边界)

Figure 8. The 1st 3 mode shapes of the porous 2D-FGM microbeam on Winkler's foundation (S-S boundary condition)

下载: 全尺寸图片幻灯片

表 1 弹性地基多孔2D-FGM微梁无量纲频率收敛性分析(S-S边界, P_x=1, P_z=1, θ=0.1, L/h=5, h=2l, λ=3×10^-6)

Table 1. Convergence verification of dimensionless frequencies of the porous 2D-FGM microbeam on Winkler's foundation (S-S boundary condition, P_x=1, P_z=1, θ=0.1, L/h=5, h=2l, λ=3×10^-6)

	N
	7	9	11	13	15	17	19	21
Ω₁	5.429 7	5.428 5	5.428 3	5.428 3	5.428 3	5.428 3	5.428 3	5.428 3
Ω₂	19.390 2	18.200 1	18.193 4	18.193 2	18.193 2	18.193 2	18.193 2	18.193 2
Ω₃	38.905 4	35.116 2	34.682 9	34.672 5	34.672 4	34.672 4	34.672 4	34.672 4

下载: 导出CSV

表 2 C-C边界条件下2D-FGM微梁一阶振动频率

Table 2. Dimensionless frequencies of the 2D functionally graded microbeam under the C-C boundary condition

model	P_z=0, P_x=0	P_z=1, P_x=0	P_z=0, P_x=1	P_z=1, P_x=1
this paper	28.585 4	23.883 1	22.223 6	19.534 0
ref. [22]	28.577 9	23.677 7	22.427 6	19.546 4

下载: 导出CSV

表 3 基于本文模型的宏观2D-FGM梁无量纲频率与文献中结果的对比(C-C边界)

Table 3. Comparison of the dimensionless fundamental frequencies of the C-C bi-directional functionally graded beam

	model	P_z=0	P_z=2	P_z=4	P_z=6	P_z=8
P_x=0	ref. [31]	6.454 1	5.872 9	4.664 3	3.557 0	2.766 1
P_x=0	this papaer	6.455 2	5.873 7	4.664 7	3.557 1	2.766 1
P_x=2	ref. [31]	6.616 8	6.021 0	4.782 0	3.646 7	2.835 9
P_x=2	this papaer	6.617 9	6.021 8	4.782 3	3.646 9	2.835 9
P_x=4	ref. [31]	7.150 6	6.506 8	5.167 9	3.941 1	3.064 8
P_x=4	this papaer	7.152 3	6.508 1	5.168 6	3.941 4	3.065 0
P_x=6	ref. [31]	8.162 0	7.427 3	5.899 0	4.498 7	3.498 5
P_x=6	this papaer	8.168 3	7.432 7	5.903 0	4.501 6	3.500 6
P_x=8	ref. [31]	9.753 2	8.875 3	7.049 3	5.376 1	4.180 8
P_x=8	this papaer	9.802 4	8.919 9	7.084 5	5.402 8	4.201 5

下载: 导出CSV

表 4 无量纲弹性地基模量对前三阶无量纲频率的影响(P_x=1, P_z=1, θ=0.1, L/h=5, h=2l, 孔隙均匀分布)

Table 4. Effects of the dimensionless elastic foundation modulus on the 1st 3 orders of dimensionless frequencies (P_x=1, P_z=1, θ=0.1, L/h=5, h=2l, uniform porosity distribution)

λ	C-C			S-S
λ	Ω₁	Ω₂	Ω₃	Ω₁	Ω₂	Ω₃
0	10.2652	23.536 3	40.299 4	5.581 7	18.666 0	35.487 9
1×10^-5	10.272 0	23.539 2	40.301 1	5.593 5	18.669 5	35.489 8
2×10^-5	10.278 7	23.542 2	40.302 8	5.605 3	18.673 0	35.491 6
3×10^-5	10.285 5	23.545 1	40.304 4	5.617 2	18.676 5	35.493 5
4×10^-5	10.292 2	23.548 0	40.306 1	5.628 9	18.680 0	35.495 3
5×10^-5	10.299 0	23.550 9	40.307 8	5.640 7	18.683 5	35.497 2
6×10^-5	10.305 7	23.553 8	40.309 5	5.652 4	18.687 0	35.499 1
7×10^-5	10.312 4	23.556 7	40.311 2	5.664 1	18.690 5	35.500 9
8×10^-5	10.319 2	23.559 6	40.312 9	5.675 8	18.694 0	35.502 8
9×10^-5	10.325 9	23.562 5	40.314 6	5.687 5	18.697 5	35.504 6
1×10^-4	10.332 6	23.565 5	40.316 3	5.699 1	18.701 0	35.506 5

下载: 导出CSV

参考文献(31)

[1]	UDUPA G, RAO S S, GANGADHARAN K V. Functionally graded composite materials: an overview[J]. Procedia Materials Science, 2014, 5: 1291-1299. doi: 10.1016/j.mspro.2014.07.442
[2]	SANKAR B V. An elasticity solution for functionally graded beams[J]. Composites Science and Technology, 2001, 61(5): 689-696. doi: 10.1016/S0266-3538(01)00007-0
[3]	MALEKZADEH P, KARAMI G, FARID M. DQEM for free vibration analysis of Timoshenko beams on elastic foundations[J]. Computational Mechanics, 2003, 31(3/4): 219-228.
[4]	SHARIAT B A S, ESLAMI M R. Buckling of thick functionally graded plates under mechanical and thermal loads[J]. Composite Structures, 2007, 78(3): 433-439. doi: 10.1016/j.compstruct.2005.11.001
[5]	BENATTA M A, MECHAB I, TOUNSI A, et al. Static analysis of functionally graded short beams including warping and shear deformation effects[J]. Computational Materials Science, 2008, 44(2): 765-773. doi: 10.1016/j.commatsci.2008.05.020
[6]	ALSHORBAGY A E, ELTAHER M A, MAHMOUD F F. Free vibration characteristics of a functionally graded beam by finite element method[J]. Applied Mathematical Modelling, 2011, 35(1): 412-425. doi: 10.1016/j.apm.2010.07.006
[7]	ŞIMŞEK M. Bi-directional functionally graded materials (BDFGMs) for free and forced vibration of Timoshenko beams with various boundary conditions[J]. Composite Structures, 2015, 133: 968-978. doi: 10.1016/j.compstruct.2015.08.021
[8]	ŞIMŞEK M. Buckling of Timoshenko beams composed of two-dimensional functionally graded material (2D-FGM) having different boundary conditions[J]. Composite Structures, 2016, 149: 304-314. doi: 10.1016/j.compstruct.2016.04.034
[9]	滕兆春, 衡亚洲, 张会凯, 等. 弹性地基上转动FGM梁自由振动的DTM分析[J]. 计算力学学报, 2017, 34(6): 712-717. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201706006.htm TENG Zhaochun, HENG Yazhou, ZHANG Huikai, et al. DTM analysis for free vibration of rotating FGM beams resting on elastic foundations[J]. Chinese Journal of Computational Mechanics, 2017, 34(6): 712-717. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG201706006.htm
[10]	蒲育, 周凤玺. FGM梁临界屈曲载荷的改进型GDQ法分析[J]. 应用基础与工程科学学报, 2019, 27(6): 1308-1320. doi: 10.16058/j.issn.1005-0930.2019.06.011 PU Yu, ZHOU Fengxi. Critical buckling loads analysis of FGM beams by a modified generalized differential quadrature method[J]. Journal of Basic Science and Engineering, 2019, 27(6): 1308-1320. (in Chinese) doi: 10.16058/j.issn.1005-0930.2019.06.011
[11]	WITVROUW A, MEHTA A. The use of functionally graded poly-sige layers for MEMS applications[J]. Materials Science Forum, 2005, 520(492/493): 255-260.
[12]	FLECK N A, MULLER G M, ASHBY M F, et al. Strain gradient plasticity: theory and experiment[J]. Acta Metallurgica et Materialia, 1994, 42(2): 475-487. doi: 10.1016/0956-7151(94)90502-9
[13]	BAŽANT Z P. Size effect in blunt fracture: concrete, rock, metal[J]. Journal of Engineering Mechanics, 1984, 110(4): 518-535. doi: 10.1061/(ASCE)0733-9399(1984)110:4(518)
[14]	YANG F, CHONG A C M, LAM D C C, et al. Couple stress based strain gradient theory for elasticity[J]. International Journal of Solids and Structures, 2002, 39(10): 2731-2743. doi: 10.1016/S0020-7683(02)00152-X
[15]	周强. 考虑表面效应的压电纳米梁的振动研究[J]. 应用数学和力学, 2020, 41(8): 853-865. doi: 10.21656/1000-0887.400330 ZHOU Qiang. Vibration of piezoelectric nanobeams with surface effects[J]. Applied Mathematics and Mechanics, 2020, 41(8): 853-865. (in Chinese) doi: 10.21656/1000-0887.400330
[16]	ŞIMŞEK M, KOCATÜRK T, AKBAŞ Ş D. Static bending of a functionally graded microscale Timoshenko beam based on the modified couple stress theory[J]. Composite Structures, 2013, 95: 740-747. doi: 10.1016/j.compstruct.2012.08.036
[17]	KE L L, WANG Y S. Size effect on dynamic stability of functionally graded microbeams based on a modified couple stress theory[J]. Composite Structures, 2011, 93(2): 342-350. doi: 10.1016/j.compstruct.2010.09.008
[18]	ŞIMŞEK M, REDDY J N. Bending and vibration of functionally graded microbeams using a new higher order beam theory and the modified couple stress theory[J]. International Journal of Engineering Science, 2013, 64: 37-53. doi: 10.1016/j.ijengsci.2012.12.002
[19]	AL-BASYOUNI K S, TOUNSI A, MAHMOUD S R. Size dependent bending and vibration analysis of functionally graded micro beams based on modified couple stress theory and neutral surface position[J]. Composite Structures, 2015, 125: 621-630. doi: 10.1016/j.compstruct.2014.12.070
[20]	AKGÖZ B, CIVALEK Ö. Free vibration analysis of axially functionally graded tapered Bernoulli-Euler microbeams based on the modified couple stress theory[J]. Composite Structures, 2013, 98: 314-322. doi: 10.1016/j.compstruct.2012.11.020
[21]	刘松正, 张波, 沈火明, 等. 准三维功能梯度微梁的尺度效应模型及微分求积有限元[J]. 应用数学和力学, 2021, 42(6): 623-636. doi: 10.21656/1000-0887.410260 LIU Songzheng, ZHANG Bo, SHEN Huoming, et al. A size-dependent quasi-3D functionally graded microbeam model andrelated differential quadrature finite elements[J]. Applied Mathematics and Mechanics, 2021, 42(6): 623-636. (in Chinese) doi: 10.21656/1000-0887.410260
[22]	雷剑, 谢宇阳, 姚明格, 等. 变截面二维功能梯度微梁的振动和屈曲特性[J]. 应用数学和力学, 2022, 43(10): 1133-1145. doi: 10.21656/1000-0887.420323 LEI Jian, XIE Yuyang, YAO Mingge, et al. Vibration and buckling characteristics of two-dimensional functionally graded microbeams with variable cross sections[J]. Applied Mathematics and Mechanics, 2022, 43(10): 1133-1145. (in Chinese) doi: 10.21656/1000-0887.420323
[23]	CHEN X, LU Y, LI Y. Free vibration, buckling and dynamic stability of bi-directional FG microbeam with a variable length scale parameter embedded in elastic medium[J]. Applied Mathematical Modelling, 2019, 67: 430-448. doi: 10.1016/j.apm.2018.11.004
[24]	CHEN D, YANG J, KITIPORNCHAI S. Elastic buckling and static bending of shear deformable functionally graded porous beam[J]. Composite Structures, 2015, 133: 54-61. doi: 10.1016/j.compstruct.2015.07.052
[25]	CHEN D, YANG J, KITIPORNCHAI S. Free and forced vibrations of shear deformable functionally graded porous beams[J]. International Journal of Mechanical Sciences, 2016, 108/109: 14-22.
[26]	LEI Y L, GAO K, WANG X, et al. Dynamic behaviors of single- and multi-span functionally graded porous beams with flexible boundary constraints[J]. Applied Mathematical Modelling, 2020, 83: 754-776.
[27]	王伟斌, 杨文秀, 滕兆春. 多孔功能梯度材料Timoshenko梁的自由振动分析[J]. 计算力学学报, 2021, 38(5): 586-594. https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG202205007.htm WANG Weibin, YANG Wenxiu, TENG Zhaochun. Free vibration analysis of porous functionally graded materials Timoshenko beam[J]. Chinese Journal of Computational Mechanics, 2021, 38(5): 586-594. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-JSJG202205007.htm
[28]	WANG Xinwei. Differential Quadrature and Differential Quadrature Based Element Methods[M]. 2015.
[29]	TORNABENE F, FANTUZZI N, UBERTINI F, et al. Strong formulation finite element method based on differential quadrature: a survey[J]. Applied Mechanics Reviews, 2015, 67(2): 020801.
[30]	吴明明. 弹性地基上的功能梯度梁力学问题研究[D]. 硕士学位论文. 邯郸: 河北工程大学, 2019. WU Mingming. Research on mechanical problems of functionally graded beams resting on the elastic foundation[D]. Master Thesis. Handan: Hebei University of Engineering, 2019. (in Chinese)
[31]	DENG H, CHENG W. Dynamic characteristics analysis of bi-directional functionally graded Timoshenko beams[J]. Composite Structures, 2016, 141: 253-263.