圆锥形负刚度超材料吸能性能研究

王竞哲; 陈保才; 朱绍伟; 陈立明

doi:10.21656/1000-0887.440055

圆锥形负刚度超材料吸能性能研究

doi: 10.21656/1000-0887.440055

王竞哲^{1, 2,},
陈保才^{1, 2},
朱绍伟^{1, 2},
陈立明^{1, 2, ,}

1.
重庆大学航空航天学院，重庆 400030
2.
重庆大学非均质材料力学重庆市重点实验室，重庆 400030

(我刊编委陈立明来稿)

基金项目:

国家自然科学基金项目 11972096

详细信息

作者简介:
王竞哲(1998—)，男，硕士生(E-mail: 202031021007@cqu.edu.cn)

通讯作者:
陈立明(1986—)，男，教授，博士，博士生导师(通讯作者. E-mail: clm07@cqu.edu.cn)

中图分类号: O34
计量
- 文章访问数: 694
- HTML全文浏览量: 257
- PDF下载量: 107
- 被引次数: 0
出版历程
- 收稿日期: 2023-03-03
- 修回日期: 2023-03-27
- 刊出日期: 2023-10-31

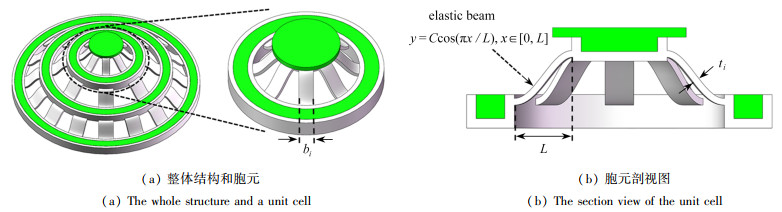
Study on Energy Absorption Performances of Conical Negative Stiffness Metamaterials

WANG Jingzhe^{1, 2
,},
CHEN Baocai^{1, 2},
ZHU Shaowei^{1, 2},
CHEN Liming^{1, 2
, ,}

1.
College of Aerospace Engineering, Chongqing University, Chongqing 400030, P.R.China
2.
Chongqing Key Laboratory of Heterogeneous Material Mechanics, Chongqing University, Chongqing 400030, P.R.China

(Contributed by CHEN Liming, M. AMM Editorial Board)

摘要

摘要: 由于负刚度超材料作为吸能材料具有可重复使用的特性，因此有必要对负刚度超材料的吸能性能和可重复使用性能进行深入研究. 采用3D打印技术制备了所设计的负刚度超材料，通过反复加载实验研究了超材料在多稳态模式和单稳态模式下的吸能性能，并采用自然时效的方法研究了残余应力对超材料吸能性能的影响. 结果表明，所设计超材料在反复加载时，随加载次数的增加，超材料的比吸能先下降后趋于稳定. 在多稳态模式和单稳态模式下，采用自然时效方法都可以有效释放超材料中的残余应力，从而提高其反复吸能性能.
- 负刚度 /
- 超材料 /
- 能量吸收
Abstract: Since negative stiffness metamaterials are reusable as energy-absorbing materials, it is necessary to investigate the energy-absorbing performances and reusability of negative stiffness metamaterials. The designed negative stiffness metamaterial was prepared with the 3D printing technology, and the energy absorption performance of the metamaterial in the multi-stable mode and the mono-stable mode was investigated by repeated loading experiments. The effect of the residual stress on the energy absorption performance of the metamaterial was studied with the natural aging method. The results show that, the specific energy absorption of the designed metamaterial first decreases and then stabilizes with the increase of the number of loading times in the case of repeated loading. In both the multi-stable mode and the mono-stable mode, the natural aging method can effectively release the residual stresses in the metamaterial, thus improving its repeated energy absorption performance.
- negative stiffness /
- metamaterial /
- energy absorption
edited-by

edited-by
注释:

1) (我刊编委陈立明来稿)

HTML全文

图 1 超材料结构设计

注为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.

Figure 1. The structural design of the metamaterial

下载: 全尺寸图片幻灯片

图 2 超材料实验变形过程

Figure 2. The experimental deformation process of the metamaterial

下载: 全尺寸图片幻灯片

图 3 超材料二十次反复加载的力-应变曲线

Figure 3. Force-strain curves for 20 times of repeated loading on the metamaterial

下载: 全尺寸图片幻灯片

图 4 多稳态模式下二十次反复加载比吸能变化

Figure 4. The change in E_SEA during 20 times of repeated loading in the multi-stable mode

下载: 全尺寸图片幻灯片

图 5 单稳态模式下二十次反复加载比吸能变化

Figure 5. The change in E_SEA during 20 times of repeated loading in the mono-stable mode

下载: 全尺寸图片幻灯片

图 6 多稳态模式和单稳态模式下二十次反复加载吸能和比吸能对比

Figure 6. The comparison of E_EA and E_SEA during 20 times of repeated loading in multi-stable and mono-stable modes

下载: 全尺寸图片幻灯片

图 7 多稳态模式下超材料第二轮反复加载的力-应变曲线

Figure 7. Force-strain curves for the 2nd round of repeated loading on the metamaterial in the multi-stable mode

下载: 全尺寸图片幻灯片

图 8 多稳态模式下超材料第一轮反复加载与第二轮反复加载的力-应变曲线对比

Figure 8. The comparison of force-strain curves for the 1st round of repeated loading and the 2nd round of repeated loading on the metamaterial in the multi-stable mode

下载: 全尺寸图片幻灯片

图 9 多稳态模式下第一轮反复加载与第二轮反复加载的比吸能对比

Figure 9. The comparison of the E_SEA between the 1st round of repeated loading and the 2nd round of repeated loading in the multi-stable mode

下载: 全尺寸图片幻灯片

图 10 单稳态模式下超材料第二轮反复加载的力-应变曲线

Figure 10. Force-strain curves for the 2nd round of repeated loading on the metamaterial in the mono-stable mode

下载: 全尺寸图片幻灯片

图 11 单稳态模式下超材料第一轮反复加载与第二轮反复加载的力-应变曲线对比

Figure 11. The comparison of force-strain curves for the 1st round of repeated loading and the 2nd round of repeated loading on the metamaterial in the mono-stable mode

下载: 全尺寸图片幻灯片

图 12 单稳态模式下第一轮反复加载与第二轮反复加载的比吸能对比

Figure 12. The comparison of the E_SEA between the 1st round of repeated loading and the 2nd round of repeated loading in the mono-stable mode

下载: 全尺寸图片幻灯片

表 1 试件材料参数

Table 1. Material parameters of the specimen

material parameter	TPU	PLA
density ρ/(kg/m³)	1 250	1 000
Poisson’s ratio ν	0.47	0.24
Young’s modulus E/MPa	75	1 024

下载: 导出CSV

参考文献(19)

[1]	CHRONOPOULOS D, ANTONIADIS I, AMPATZIDIS T. Enhanced acoustic insulation properties of composite metamaterials having embedded negative stiffness inclusions[J]. Extreme Mechanics Letters, 2017, 12: 48-54. doi: 10.1016/j.eml.2016.10.012
[2]	ESIN M, PASTERNAK E, DYSKIN A V. Stability of chains of oscillators with negative stiffness normal, shear and rotational springs[J]. International Journal of Engineering Science, 2016, 108: 16-33. doi: 10.1016/j.ijengsci.2016.08.002
[3]	YANG H, MA L. Multi-stable mechanical metamaterials by elastic buckling instability[J]. Journal of Materials Science, 2019, 54: 3509-3526. doi: 10.1007/s10853-018-3065-y
[4]	CHEN B, CHEN L, DU B, et al. Novel multifunctional negative stiffness mechanical metamaterial structure: tailored functions of multi-stable and compressive mono-stable[J]. Composites(Part B): Engineering, 2021, 204: 108501. doi: 10.1016/j.compositesb.2020.108501
[5]	SHAN S, KANG S H, RANEY J R, et al. Multistable architected materials for trapping elastic strain energy[J]. Advanced Materials, 2015, 27(29): 4296-4301. doi: 10.1002/adma.201501708
[6]	TAN X, WANG B, YAO K, et al. Novel multi-stable mechanical metamaterials for trapping energy through shear deformation[J]. International Journal of Mechanical Sciences, 2019, 164: 105168. doi: 10.1016/j.ijmecsci.2019.105168
[7]	WANG B, TAN X, ZHU S, et al. Cushion performance of cylindrical negative stiffness structures: analysis and optimization[J]. Composite Structures, 2019, 227: 111276. doi: 10.1016/j.compstruct.2019.111276
[8]	TAN X, WANG B, CHEN S, et al. A novel cylindrical negative stiffness structure for shock isolation[J]. Composite Structures, 2019, 214: 397-405. doi: 10.1016/j.compstruct.2019.02.030
[9]	TAN X, CHEN S, ZHU S, et al. Reusable metamaterial via inelastic instability for energy absorption[J]. International Journal of Mechanical Sciences, 2019, 155: 509-517. doi: 10.1016/j.ijmecsci.2019.02.011
[10]	HAGHPANAH B, SALARI-SHARIF L, POURRAJAB P, et al. Multistable shape-reconfigurable architected materials[J]. Advanced Materials, 2016, 28(36): 7915-7920. doi: 10.1002/adma.201601650
[11]	RAFSANJANI A, PASINI D. Bistable auxetic mechanical metamaterials inspired by ancient geometric motifs[J]. Extreme Mechanics Letters, 2016, 9(2): 291-296.
[12]	杨航, 马力. 多材料点阵结构的热可编程力学行为[J]. 应用数学和力学, 2022, 43(5): 534-552. doi: 10.21656/1000-0887.430104 YANG Hang, MA Li. Multimaterial lattice structures with thermally programmable mechanical behaviors[J]. Applied Mathematics and Mechanics, 2022, 43(5): 534-552. (in Chinese) doi: 10.21656/1000-0887.430104
[13]	AN X, LAI C, FAN H, et al. 3D acoustic metamaterial-based mechanical metalattice structures for low-frequency and broadband vibration attenuation[J]. International Journal of Solids and Structures, 2020, 191/192: 293-306. doi: 10.1016/j.ijsolstr.2020.01.020
[14]	LE T D, AHN K K. Experimental investigation of a vibration isolation system using negative stiffness structure[J]. International Journal of Mechanical Sciences, 2013, 70(5): 99-112.
[15]	FRENZEL T, FINDEISEN C, KADIC M, et al. Tailored buckling microlattices as reusable light-weight shock absorbers[J]. Advanced Materials, 2016, 28(28): 5865-5870. doi: 10.1002/adma.201600610
[16]	JIANG H, LE BARBENCHON L, BEDNARCYK B A, et al. Bioinspired multilayered cellular composites with enhanced energy absorption and shape recovery[J]. Additive Manufacturing, 2020, 36: 101430. doi: 10.1016/j.addma.2020.101430
[17]	ZHU S, WANG B, TAN X, et al. A novel bi-material negative stiffness metamaterial in sleeve-type via combining rigidity with softness[J]. Composite Structures, 2021, 262: 113381. doi: 10.1016/j.compstruct.2020.113381
[18]	周世奇, 侯秀慧, 邓子辰. 一般宏观应力状态下凹角蜂窝结构的屈曲性能分析[J]. 应用数学和力学, 2023, 44(1): 12-24. doi: 10.21656/1000-0887.430202 ZHOU Shiqi, HOU Xiuhui, DENG Zichen. Buckling analysis of re-entrant honeycomb structures under general macroscopic stress states[J]. Applied Mathematics and Mechanics, 2023, 44(1): 12-24. (in Chinese) doi: 10.21656/1000-0887.430202
[19]	陈保才. 多稳态/单稳态可调控结构设计与吸能机制研究[D]. 硕士学位论文. 重庆: 重庆大学, 2021. CHEN Baocai. Study on the design and energy absorption mechanism in tailored multi-stable/mono-stable structure[D]. Master Thesis. Chongqing: Chongqing University, 2021. (in Chinese)