基于扭转-滑移耦合约束的梁桥抗倾覆滑移整体稳定分析

童上航; 肖汝诚; 庄冬利; 孙斌

doi:10.21656/1000-0887.440138

基于扭转-滑移耦合约束的梁桥抗倾覆滑移整体稳定分析

doi: 10.21656/1000-0887.440138

同济大学桥梁工程系，上海 200092

(我刊编委肖汝诚来稿)

基金项目:

国家自然科学基金项目 52378185

详细信息

作者简介:
童上航(2000—)，男，硕士生(E-mail: tongttmail@163.com)

通讯作者:
孙斌(1977—)，男，副教授，博士生导师(通讯作者. E-mail: sunbin@tongji.edu.cn)

中图分类号: U441⁺.4;O343.5
计量
- 文章访问数: 525
- HTML全文浏览量: 153
- PDF下载量: 67
- 被引次数: 0
出版历程
- 收稿日期: 2023-05-09
- 修回日期: 2023-07-30
- 刊出日期: 2023-12-01

Overall Overturning and Sliding Stability Analysis of Girder Bridges Under Torsion-Slippage Coupling Constraints

Department of Bridge Engineering, Tongji University, Shanghai 200092, P.R.China

(Contributed by XIAO Rucheng, M. AMM Editorial Board)

摘要

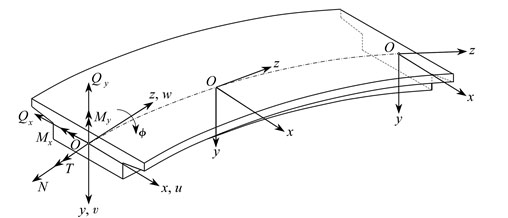
摘要: 为了分析考虑支座失效的梁桥整体稳定问题，推导了七自由度曲梁单元刚度矩阵，并建立了部分支座脱空和滑移等情况下的边界约束方程，利用Newton-Raphson迭代法求解了含支座约束的有限元总方程，并提出了依据支座受力状态判断梁桥失稳模式的方法，编制了相应程序. 以简支超静定曲梁为例，验证了所提出的七自由度曲梁单元的精度；进一步利用所提出方法分析了某匝道桥倒塌事故，通过对比传统杆系有限元方法，验证了所提出的方法能更精确地模拟各种支座失效情况下的梁桥平衡状态.
- 曲梁单元 /
- 约束方程 /
- 扭转-滑移耦合效应 /
- 倾覆 /
- 滑移
Abstract: To analyze the overall overturning and sliding stability of girder bridges with bearing failures, the stiffness matrix of the 7-DOF curved beam element was derived, and the constraint equations for the bearing detachment and slippage failures were presented. The finite element equation with the constraint equations was solved with the Newton-Raphson method. A process of judging the instability modes of girder bridges according to the bearing failures was established, and the corresponding program was compiled. The accuracy of the 7-DOF curved beam element was verified through calculation of a simply supported statically indeterminate curved beam. A curved ramp bridge collapse accident was analyzed with the proposed method. The results show that, the proposed method could more accurately simulate the equilibrium state of the girder bridge under various bearing failure conditions, in comparison with the traditional bar-system finite element model.
- curved beam element /
- constraint equation /
- torsion-slippage coupling effect /
- overturn /
- slip
edited-by

edited-by
注释:

1) (我刊编委肖汝诚来稿)

HTML全文

图 1 曲梁截面内力分量与流动坐标系

Figure 1. The internal force components and the co-rotational coordinate system of the curved beam

下载: 全尺寸图片幻灯片

图 2 七自由度曲梁单元

Figure 2. The curved beam element with 7 degrees of freedom

下载: 全尺寸图片幻灯片

图 3 单墩支座滑移计算简图

Figure 3. Schematic of the bearing slippage on a single column

下载: 全尺寸图片幻灯片

图 4 挠度的理论结果与有限元结果比较

注为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.

Figure 4. Comparison of deflections given by theoretical

下载: 全尺寸图片幻灯片

图 5 扭转角的理论结果与有限元结果比较

注为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.

Figure 5. Comparison of torsion angles given by theoretical and finite element methods and finite element methods

下载: 全尺寸图片幻灯片

图 6 平面布置图(单位：m)

Figure 6. The bridge layout in plan (unit: m)

下载: 全尺寸图片幻灯片

图 7 事故桥梁横断面(单位：cm)

Figure 7. The cross section of the bridge main beam (unit: cm)

下载: 全尺寸图片幻灯片

图 8 不同荷载放大率下各支座的竖向支反力

Figure 8. The vertical support reaction of each bearing under different load amplification ratios

下载: 全尺寸图片幻灯片

图 9 所提出计算模型与传统模型的竖向支反力对比

Figure 9. Comparison of the vertical support reactions given by the proposed model with the traditional model

下载: 全尺寸图片幻灯片

表 1 近年梁桥倾覆事故

Table 1. Bridge overturning accidents in recent years

time of accident	bridge site	vehicle load
2007-10	the viaduct of Minzu East Road in Baotou City	3 vehicles weighing approximately 100 t each
2009-07	the ramp bridge of Tianjin-Shanxi expressway in Tianjin	3 vehicles weighing approximately 140 t each
2011-02	the Chunhui E-ramp bridge in Shangyu City, Zhejiang	3 vehicles weighing approximately 120 t each
2012-08	the viaduct of the third ring road in Harbin City	4 vehicles weighing approximately 120 t each
2015-06	the ramp bridge of Guangdong-Jiangxi expressway in Heyuan City	3 vehicles weighing 80~115 t each
2019-10	the bridge of Xigang Road in Wuxi City	2 vehicles weighing approximately 160 t each
2021-12	the Huahu D-ramp bridge of Shanghai-Chongqing expressway	a 67.67 m long car unit with a total weight of 522 t

下载: 导出CSV

表 2 支座约束方程

Table 2. The constraint equations of bearings

failure condition	ϕ>0	ϕ < 0
the beam slipping at the bearing on a single column support	$\left\{ \begin{array}{l}g_{1}= u \tan \phi-v+h_{\mathrm{b}}(1-\sec \phi)=0, \\ g_{2}= -m_{z}+P_{x}\left(v-h_{\mathrm{b}}\right)-P_{y} u=0, \\ g_{3}= P_{y}(\sin \phi-\mu \cos \phi)+ \\ \; \; \; \; \; \; \; P_{x}(\cos \phi+\mu \sin \phi)=0\end{array} \right.$	$\left\{ {\begin{array}{*{20}{l}} {{g_1} = u\tan \phi - v + {h_{\rm{b}}}(1 - \sec \phi) = 0, }\\ {{g_2} = - {m_z} + {P_x}\left({v - {h_{\rm{b}}}} \right) - {P_y}u = 0, }\\ {{g_3} = {P_y}(\sin \phi + \mu \cos \phi) + }\\ {\; \; \; \; \; \; \; {P_x}(\cos \phi - \mu \sin \phi) = 0} \end{array}} \right.$
the beam detaching from the bearing at the end of the bridge, without slipping (l is half of the bearing spacing)	$\left\{\begin{array}{l}g_1=-u+l(1-\cos \phi)+h_{\mathrm{b}} \sin \phi=0, \\ g_2=-v+h_{\mathrm{b}}(1-\cos \phi)-l \sin \phi=0, \\ g_3=-m_z+P_y(l-u)-P_x\left(h_{\mathrm{b}}-v\right)=0\end{array}\right.$	$\left\{\begin{array}{l}g_1=-u-l(1-\cos \phi)+h_{\mathrm{b}} \sin \phi=0, \\ g_2=-v+h_{\mathrm{b}}(1-\cos \phi)+l \sin \phi=0, \\ g_3=-m_z-P_y(l+u)-P_x\left(h_{\mathrm{b}}-v\right)=0\end{array}\right.$
the beam detaching and slipping from the bearing at the end of the bridge (l is half of the bearing spacing)	$\left\{\begin{aligned} g_1= & u \tan \phi-l \tan \phi-v+h_{\mathrm{b}}(1-\sec \phi)=0, \\ g_2= & P_x(\cos \phi+\mu \sin \phi)+ \\ & P_y(\sin \phi-\mu \cos \phi)=0, \\ g_3= & -m_z+P_y(l-u)-P_x\left(h_{\mathrm{b}}-v\right)=0\end{aligned}\right.$	$\left\{\begin{aligned} g_1= & u \tan \phi+l \tan \phi-v+h_{\mathrm{b}}(1-\sec \phi)=0, \\ g_2= & P_x(\cos \phi-\mu \sin \phi)+ \\ & P_y(\sin \phi+\mu \cos \phi)=0, \\ g_3= & -m_z-P_y(l+u)-P_x\left(h_{\mathrm{b}}-v\right)=0\end{aligned}\right.$

下载: 导出CSV

表 3 各参数取值

Table 3. The value of each parameter

parameter	value
elastic modulus E/MPa	3.5×10⁴
shear modulus G/MPa	1.46×10⁴
Poisson’s ratio ν	0.2
moment of inertia around x axis I_x/m⁴	0.5
moment of inertia around y axis I_y/m⁴	10
torsional moment of inertia I_d/m⁴	1.5
sectorial moment of inertia I_ω/m⁶	4.5
section area A/m²	5
curved beam radius R/m	50
curved beam length L/m	30
uniformly distributed torque m_z/(kN·m/m)	-20
uniformly distributed load q_y/(kN/m)	10

下载: 导出CSV

参考文献(18)

[1]	姜爱国, 杨志. 独柱墩曲线梁桥倾覆轴线研究[J]. 世界桥梁, 2013, 41(4): 58-61. JIANG Aiguo, YANG Zhi. Study of overturning axis of curved beam bridge with single-column piers[J]. World Bridges, 2013, 41(4): 58-61. (in Chinese)
[2]	庄冬利. 偏载作用下箱梁桥抗倾覆稳定问题的探讨[J]. 桥梁建设, 2014, 44(2): 27-31. ZHUANG Dongli. Study of overturning stability issues of box girder bridges under action of eccentric load[J]. Bridge Construction, 2014, 44(2): 27-31. (in Chinese)
[3]	American Association of State Highway and Transportation Officials. AASHTO LRFD bridge design specifications[S]. 4th ed. 2007.
[4]	公路钢筋混凝土及预应力混凝土桥涵设计规范: JTG 3362—2018[S]. 北京: 人民交通出版社, 2018. Specifications for design of highway reinforced concrete and prestressed concrete bridges and culverts: JTG 3362—2018[S]. Beijing: China Communication Press, 2018. (in Chinese)
[5]	彭卫兵, 朱志翔, 陈光军, 等. 梁桥倾覆机理、破坏模式与计算方法研究[J]. 土木工程学报, 2019, 52(12): 104-113. PENG Weibing, ZHU Zhixiang, CHEN Guangjun, et al. Research on overturning failure mode of beam bridges and applicability of calculation method[J]. China Civil Engineering Journal, 2019, 52(12): 104-113. (in Chinese)
[6]	SHI X F, ZHOU Z J, RUAN X. Failure analysis of a girder bridge collapse under eccentric heavy vehicles[J]. Journal of Bridge Engineering, 2016, 21(12): 05016009. doi: 10.1061/(ASCE)BE.1943-5592.0000964
[7]	XIONG W, CAI C S, KONG B, et al. Overturning-collapse modeling and safety assessment for bridges supported by single-column piers[J]. Journal of Bridge Engineering, 2017, 22(11): 04017084. doi: 10.1061/(ASCE)BE.1943-5592.0001133
[8]	SHI X F, CAO Z, MA H Y, et al. Failure analysis on a curved girder bridge collapse under eccentric heavy vehicles using explicit finite element method: case study[J]. Journal of Bridge Engineering, 2018, 23(3): 05018001. doi: 10.1061/(ASCE)BE.1943-5592.0001201
[9]	PENG W B, ZHAO H, DAI F, et al. Analytical method for overturning limit analysis of single-column pier bridges[J]. Journal of Performance of Constructed Facilities, 2017, 31(4): 04017007. doi: 10.1061/(ASCE)CF.1943-5509.0000999
[10]	SHI X F, ZHOU Z J, MA H Y, et al. Failure mechanism and design method for box girder bridge with interior hinged supports under eccentrically vertical loads[J]. Structures, 2023, 48: 438-449. doi: 10.1016/j.istruc.2022.12.101
[11]	彭卫兵, 潘若丹, 马俊, 等. 独柱墩梁桥倾覆破坏模式与计算方法研究[J]. 桥梁建设, 2016, 46(2): 25-30. PENG Weibing, PAN Ruodan, MA Jun, et al. Study of overturning failure modes and anti-overturning calculation methods for single-column pier beam bridges[J]. Bridge Construction, 2016, 46(2): 25-30. (in Chinese)
[12]	ZHUANG D L, XIAO R C, JIA L J, et al. Failure analysis for overall stability against sliding and overturning of a girder bridge[J]. Engineering Failure Analysis, 2020, 109: 104271. doi: 10.1016/j.engfailanal.2019.104271
[13]	庄冬利. 梁桥抗滑移和倾覆整体稳定的分析方法与性能评估[D]. 博士学位论文. 上海: 同济大学, 2023. ZHUANG Dongli. Analysis methods and performance evaluation for overall stability against sliding and overturning of girder bridge[D]. PhD Thesis. Shanghai: Tongji University, 2023. (in Chinese)
[14]	邵荣光, 夏淦. 混凝土弯梁桥[M]. 北京: 人民交通出版社, 1994. SHAO Rongguang, XIA Gan. Concrete Curved-Girders Bridge[M]. Beijing: Chinese Communications Press, 1994. (in Chinese)
[15]	魏娜, 张元海, 姚晓东. 波形钢腹板箱梁的约束扭转剪应力分析[J]. 应用数学和力学, 2020, 41(4): 386-395. doi: 10.21656/1000-0887.390329 WEI Na, ZHANG Yuanhai, YAO Xiaodong. Analysis on restrained torsional shear stresses of box girders with corrugated steel webs[J]. Applied Mathematics and Mechanics, 2020, 41(4): 386-395. (in Chinese) doi: 10.21656/1000-0887.390329
[16]	朱莉莉, 赵颖华. 翘曲空间曲线梁自然坐标精确解[J]. 应用数学和力学, 2008, 29(7): 846-854. http://www.applmathmech.cn/article/id/1105 ZHU Lili, ZHAO Yinghua. Exact solution of spatial warping curved beams in natural coordinates[J]. Applied Mathematics and Mechanics, 2008, 29(7): 846-854. (in Chinese) http://www.applmathmech.cn/article/id/1105
[17]	王勖成. 有限单元法[M]. 北京: 清华大学出版社, 2003. WANG Xucheng. Finite Element Method[M]. Beijing: Tsinghua University Press, 2003. (in Chinese)
[18]	公路桥梁板式橡胶支座: JT/T 4—2019[S]. 北京: 人民交通出版社, 2019. Laminated bearing for highway bridge: JT/T 4—2019[S]. Beijing: China Communication Press, 2019. (in Chinese)