表面效应对热电材料中纳米孔周围热应力的影响

赵婕燕; 杨海兵

doi:10.21656/1000-0887.440151

表面效应对热电材料中纳米孔周围热应力的影响

doi: 10.21656/1000-0887.440151

赵婕燕^1,,
杨海兵^2, ,

1.
华南理工大学土木与交通学院，广州 510641
2.
河海大学力学与材料学院，南京 211100

基金项目:

国家自然科学基金项目 11902116

广东省自然科学基金项目 2022A1515011773

详细信息

作者简介:
赵婕燕(1998—)，女，硕士生(E-mail: 18768174161@163.com)

通讯作者:
杨海兵(1988—)，男，讲师，博士(通讯作者. E-mail: yanghb@scut.edu.cn)

中图分类号: TB332;O39
计量
- 文章访问数: 459
- HTML全文浏览量: 181
- PDF下载量: 54
- 被引次数: 0
出版历程
- 收稿日期: 2023-05-15
- 修回日期: 2023-06-15
- 刊出日期: 2023-11-01

Surface Effects on Thermal Stresses Around the Nanohole in Thermoelectric Material

ZHAO Jieyan^1
,,
YANG Haibing^{2
, ,}

1.
School of Civil Engineering and Transportation, South China University of Technology, Guangzhou 510641, P.R.China
2.
College of Mechanics and Materials, Hohai University, Nanjing 211100, P.R.China

摘要

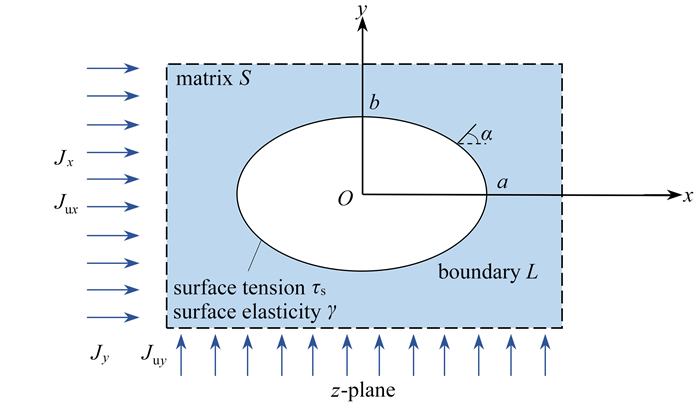
摘要: 基于完整Gurtin-Murdoch(G-M)低阶表面能模型，进一步探讨了纳米尺度下表面效应的影响. 建立了合理考虑构型变化的应力边界条件，实现了研究尺度从宏观到微观的转变. 利用复变函数理论和保角映射技术，构建了用于纳米尺度下的热-电-力理论框架模型，得到了热电基体中纳米孔周围热场、温度场以及应力场的半解析解. 数值结果表明，相对于完整G-M模型，简化G-M模型(忽略孔洞构型变化的影响)往往会高估表面效应和远场热电载荷对热应力分布的影响. 此外，表面效应的存在将在一定程度上缓解纳米孔周围的热应力集中.
- 热电材料 /
- 完整Gurtin-Murdoch模型 /
- 表面效应 /
- 复变函数理论 /
- 纳米孔
Abstract: Based on the complete Gurtin-Murdoch (G-M) low-order surface energy model, the surface effects at nanoscale were further explored. The transition from macroscale to microscale was achieved through construction of reasonable stress boundary conditions in view of the change of hole geometry configuration. With the series expansion techniques and complex variable methods, the semi-analytic solutions for the electric field, the temperature field, and the full stress field in the vicinity of the nanohole within the thermoelectric matrix were derived eventually with a built thermal-electrical-force theoretical framework model at nanoscale. Numerical results show that, compared with the complete G-M model, the simplified G-M model (neglecting the effects of nanohole geometry changes) would overestimate the surface effects and far-field thermoelectric loading effects on the thermal stress distributions. In addition, the surface effects can relieve the thermal stress concentration around the nanohole to some extent.
- thermoelectric material /
- complete Gurtin-Murdoch model /
- surface effect /
- complex function theory /
- nanohole

HTML全文

图 1 包含纳米孔的无限大热电基体

Figure 1. The infinite thermoelectric matrix with a nanohole

下载: 全尺寸图片幻灯片

图 2 微观尺度下，本文工作与前人工作的比较

Figure 2. Comparison of this work with previous work at microscale

下载: 全尺寸图片幻灯片

图 3 表面张力对圆孔周围环向热应力的影响

Figure 3. Effects of surface tensions on hoop thermal stresses around the circular hole

下载: 全尺寸图片幻灯片

图 4 环向热应力随表面张力的线性变化

Figure 4. Linear variations of hoop thermal stresses with the surface tension

下载: 全尺寸图片幻灯片

图 5 纳米孔周围的环向热应力

Figure 5. Hoop thermal stresses around the nanohole

下载: 全尺寸图片幻灯片

图 6 孔周残余应力分布

Figure 6. The residual hoop stress distributions around the nanohole

下载: 全尺寸图片幻灯片

图 7 表面张力的引入对环向热应力和由热电外载引起的环向热应力的影响

Figure 7. The influences of the surface tension on the hoop thermal stress and the hoop thermal stress induced by thermoelectric external loading

下载: 全尺寸图片幻灯片

图 8 表面效应对由热电外载引起的环向热应力的影响

Figure 8. The surface effects on the hoop thermal stress induced by thermoelectric external loading

下载: 全尺寸图片幻灯片

表 1 Bi₂Te₃和PbTe的材料性能

Table 1. Properties of the Bi₂Te₃ material and the PbTe material

	δ/(S/m)	E/GPa	ε/(V/K)	κ/(W/(m·K))	λ/K^-1	ν
Bi₂Te₃	1.1×10⁵	47	2×10^－4	1.6	2.7×10^－5	0.4
PbTe	1×10⁴	58	3×10^－4	1.5	2×10^－5	0.29

下载: 导出CSV

表 2 不同表面弹性与表面张力共同作用下的环向应力值(单位：MPa)

Table 2. Hoop stresses under the combined actions of different surface elasticities and surface tensions (unit: MPa)

surface elasticity γ/(N/m)	δ_tt/MPa
surface elasticity γ/(N/m)	τ_s=0.2 N/m	τ_s=0.6 N/m	τ_s=1.0 N/m
0	138.587 0	136.587 0	134.587 0
0.2	138.573 3	136.573 3	134.573 4
0.6	138.545 8	136.546 0	134.546 1
1.0	138.518 3	136.518 6	134.518 9

下载: 导出CSV

参考文献(30)

[1]	BISWAS K, HE J Q, BLUM I D, et al. High-performance bulk thermoelectrics with all-scale hierarchical architectures[J]. Nature, 2012, 489: 414-418. doi: 10.1038/nature11439
[2]	ZHAO L D, LO S H, ZHANG Y S, et al. Ultralow thermal conductivity and high thermoelectric figure of merit in SnSe crystals[J]. Nature, 2014, 508: 373-377. doi: 10.1038/nature13184
[3]	MARTIN-GONZALEZ M, CABALLERO-CALERO O, DIAZ-CHAO P. Nanoengineering thermoelectrics for 21st century: energy harvesting and other trends in the field[J]. Renewable & Sustainable Energy Reviews, 2013, 24: 288-305.
[4]	LIU W S, YAN X, CHEN G, et al. Recent advances in thermoelectric nanocomposites[J]. Nano Energy, 2012, 1(1): 42-56. doi: 10.1016/j.nanoen.2011.10.001
[5]	DRESSELHAUS M S, CHEN G, TANG M Y, et al. New directions for low-dimensional thermoelectric materials[J]. Advanced Materials, 2007, 19(8): 1043-1053. doi: 10.1002/adma.200600527
[6]	WU Y H, ZHAI R S, ZHU T J, et al. Enhancing room temperature thermoelectric performance of n-type polycrystalline bismuth-telluride-based alloys via Ag doping and hot deformation[J]. Materials Today Physics, 2017, 2: 62-68. doi: 10.1016/j.mtphys.2017.09.001
[7]	WANG Y, LIU W D, GAO H, et al. High porosity in nanostructured n-type Bi₂Te₃ obtaining ultralow lattice thermal conductivity[J]. ACS Applied Materials & Interfaces, 2019, 11(34): 31237-31244.
[8]	XU B A, FENG T L, AGNE M T, et al. Highly porous thermoelectric nanocomposites with low thermal conductivity and high figure of merit from large-scale solution-synthesized Bi₂Te_2.5Se_0.5 hollow nanostructures[J]. Angewandte Chemie-International Edition, 2017, 56(13): 3546-3551. doi: 10.1002/anie.201612041
[9]	TARKHANYAN R H, NIARCHOS D G. Reduction of thermal conductivity in porous "gray" materials[J]. APL Materials, 2014, 2(7): 076107. doi: 10.1063/1.4886220
[10]	DUAN H L, WANG J, KARIHALOO B L, et al. Nanoporous materials can be made stiffer than non-porous counterparts by surface modification[J]. ACTA Materialia, 2006, 54(11): 2983-2990. doi: 10.1016/j.actamat.2006.02.035
[11]	YU C B, YANG H B, SONG K, et al. Stress concentration around an arbitrarily-shaped hole in nonlinear fully coupled thermoelectric materials[J]. Journal of Mechanics of Materials and Structures, 2019, 14(2): 259-276. doi: 10.2140/jomms.2019.14.259
[12]	崔春丽, 徐耀玲. 预测纳米纤维复合材料有效弹性性能的界面模型和界面相模型[J]. 应用数学和力学, 2022, 43(8): 877-887. doi: 10.21656/1000-0887.420231 CUI Chunli, XU Yaoling. The interface model and the interphase model for predicting the effective elastic properties of nano-fiber composites[J]. Applied Mathematics and Mechanics, 2022, 43(8): 877-887. (in Chinese) doi: 10.21656/1000-0887.420231
[13]	GURTIN M E, MURDOCH A I. A continuum theory of elastic material surfaces[J]. Archive for Rational Mechanics Analysis, 1975, 57(4): 291-323. doi: 10.1007/BF00261375
[14]	DAI M, GAO C F, RU C Q. Surface tension-induced stress concentration around a nanosized hole of arbitrary shape in an elastic half-plane[J]. Meccanica, 2014, 49(12): 2847-2859. doi: 10.1007/s11012-014-0030-y
[15]	WANG S, YANG H B, GAO C F, et al. In-plane stress analysis of two nanoscale holes under surface tension[J]. Archive of Applied Mechanics, 2020, 90(6): 1363-1372. doi: 10.1007/s00419-020-01672-9
[16]	冯国益, 肖俊华, 苏梦雨. 考虑表面效应时孔边均布径向多裂纹Ⅲ型断裂力学分析[J]. 应用数学和力学, 2020, 41(4): 376-385. doi: 10.21656/1000-0887.400177 FENG Guoyi, XIAO Junhua, SU Mengyu. Fracture mechanics analysis of mode-Ⅲ fadial multi cracks on the edge of a hole with surface effects[J]. Applied Mathematics and Mechanics, 2020, 41(4): 376-385. (in Chinese) doi: 10.21656/1000-0887.400177
[17]	WANG S, DAI M, RU C Q, et al. Surface tension-induced interfacial stresses around a nanoscale inclusion of arbitrary shape[J]. Zeitschrift fur Angewandte Mathematik und Physik, 2017, 68(6): 127. doi: 10.1007/s00033-017-0876-7
[18]	WANG S, LI X Y, YI X, et al. Morphological changes of nanofiber cross-sections due to surface tension[J]. Extreme Mechanics Letters, 2021, 44: 101211. doi: 10.1016/j.eml.2021.101211
[19]	黄汝超, 陈永强. 残余界面应力对粒子填充热弹性纳米复合材料有效热膨胀系数的影响[J]. 应用数学和力学, 2011, 32(11): 1283-1293. doi: 10.3879/j.issn.1000-0887.2011.11.003 HUANG Ruchao, CHEN Yongqiang. Effects of residual interface stress on effective thermal expansion coefficient of particle-filled composite[J]. Applied Mathematics and Mechanics, 2011, 32(11): 1283-1293. (in Chinese) doi: 10.3879/j.issn.1000-0887.2011.11.003
[20]	SONG K, SONG H P, SCHIAVONE P, et al. The effects of surface elasticity on the thermal stress around a circular nano-hole in a thermoelectric material[J]. Mathematics and Mechanics of Solids, 2019, 24(10): 3156-3166. doi: 10.1177/1081286519837315
[21]	DAI M, GHARAHI A, SCHIAVONE P. Note on the deformation-induced change in the curvature of a material surface in plane deformations[J]. Mechanics Research Communications, 2018, 94: 88-90. doi: 10.1016/j.mechrescom.2018.10.001
[22]	DAI M, SCHIAVONE P. Deformation-induced change in the geometry of a general material surface and its relation to the Gurtin-Murdoch model[J]. Journal of Applied Mechanics-Transactions of the ASME, 2020, 87(6): 061005. doi: 10.1115/1.4046635
[23]	ZHANG A B, WANG B L. Explicit solutions of an elliptic hole or a crack problem in thermoelectric materials[J]. Engineering Fracture Mechanics, 2016, 151: 11-21. doi: 10.1016/j.engfracmech.2015.11.013
[24]	WANG P, WANG B L, WANG K F, et al. Analysis of inclusion in thermoelectric materials: the thermal stress field and the effect of inclusion on thermoelectric properties[J]. Composites (Part B): Engineering, 2019, 166: 130-138. doi: 10.1016/j.compositesb.2018.11.120
[25]	MOGILEVSKAYA S G, CROUCH S L, STOLARSKI H K. Multiple interacting circular nano-inhomogeneities with surface/interface effects[J]. Journal of the Mechanics and Physics of Solids, 2008, 56(6): 2298-2327. doi: 10.1016/j.jmps.2008.01.001
[26]	DAI M, WANG Y J, SCHIAVONE P. Integral-type stress boundary condition in the complete Gurtin-Murdoch surface model with accompanying complex variable representation[J]. Journal of Elasticity, 2019, 134(2): 235-241. doi: 10.1007/s10659-018-9695-0
[27]	PEI P Y, YANG H B, DAI M. Consistency of the boundary value problem of an elastic body involving surface tension in small deformations[J]. Mathematics and Mechanics of Solids, 2023, 28(6): 1488-1499. doi: 10.1177/10812865221122151
[28]	MENG Q L, KONG S, HUANG Z W, et al. Simultaneous enhancement in the power factor and thermoelectric performance of copper sulfide by In₂S₃ doping[J]. Journal of Materials Chemistry A, 2016, 4(32): 12624-12629. doi: 10.1039/C6TA03780A
[29]	DENNLER G, CHMIELOWSKI R, JACOB S, et al. Are binary copper sulfides/selenides really new and promising thermoelectric materials?[J]. Advanced Energy Materials, 2014, 4(9): 1301581. doi: 10.1002/aenm.201301581
[30]	DAI M, LI M, SCHIAVONE P. Plane deformations of an inhomogeneity-matrix system incorporating a compressible liquid inhomogeneity and complete Gurtin-Murdoch interface model[J]. Journal of Applied Mechanics-Transactions of the ASME, 2018, 85(12): 121010. doi: 10.1115/1.4041469