Stretching Flow and Magnetic Diffusion Analysis of Maxwell Magnetic Nanofluids in Non-Uniform Magnetic Fields
-
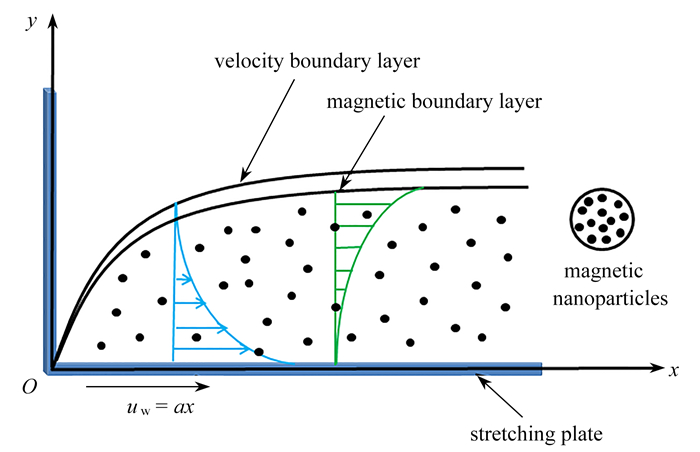
摘要: 磁性纳米颗粒可以提升聚合物的导电性和导热性等性能，被广泛应用于机械、生物医学、能源存储等领域.当外界施加非均匀磁场时，感应磁场在高Reynolds数的情况下不可忽略.为探究磁性纳米颗粒对层流边界层内黏弹性流体非稳态拉伸流动与磁扩散的影响，将时间分布阶Maxwell本构方程与动量方程耦合，建立了二维不可压缩Maxwell磁纳米流体的速度与磁扩散偏微分方程组.采用有限差分法进行数值分析，通过控制磁性纳米颗粒种类、体积分数和磁参数大小，分析了流体的速度和感应磁场在边界层内的分布.研究发现：在熔融聚合物中添加Fe2O3纳米颗粒后，流体的速度、感应磁场最大，速度和磁边界层的厚度最厚；Maxwell纳米流体的松弛时间参数增大，速度与磁扩散均减小；另外，随着磁参数增大，流体的速度边界层厚度减小，磁边界层厚度增大；Fe3O4纳米颗粒的体积分数越大，流体流动越快，感应磁场越小.因此，非均匀磁场下在聚合物中添加磁性纳米颗粒的研究，为改善材料的性能给予了可参考的数据.Abstract: Magnetic nanoparticles can enhance the electrical and thermal conductivity of polymers, which are widely used in fields such as machinery, biomedicine, and energy storage. When a non-uniform magnetic field is imposed externally, the induced magnetic field cannot be ignored in the case of high Reynolds numbers. To explore the effects of magnetic nanoparticles on the unsteady flow and magnetic diffusion of viscoelastic fluid over the stretching sheet within the laminar boundary layer, the time distributed-order Maxwell constitutive equation was coupled with the momentum equation to establish partial differential equations for the velocity and magnetic diffusion of a 2D incompressible Maxwell magnetic nanofluid. Numerical analysis was performed with the finite difference method, and the velocity and the induced magnetic field distribution of the fluid within the boundary layer were analyzed by control of the magnetic nanoparticle type, the volume fraction and the magnetic parameter magnitude. The results show that, the velocity and induced magnetic field of the fluid are the largest in the case of Fe2O3 nanoparticles added to molten polymers, besides, the velocity and magnetic boundary layer thickness is the largest. With the increase of the Maxwell nanofluid relaxation time parameter, both the velocity and the magnetic diffusion will decrease. In addition, the velocity boundary layer thickness and the magnetic boundary layer thickness of the fluid decrease with the magnetic parameter. The larger the volume fraction of Fe3O4 nanoparticles is, the faster the fluid flow and the smaller the induced magnetic field will be. Therefore, the study of the addition of magnetic nanoparticles to polymers in non-uniform magnetic fields gives referential data for improving material properties.
-
图 3 不同磁性纳米颗粒对速度的影响
Figure 3. Effects of different magnetic nanoparticles on the velocity
图 4 不同磁性纳米颗粒对感应磁场的影响
Figure 4. Effects of different magnetic nanoparticles on the induced magnetic field
图 10 不同λ1对感应磁场的影响
Figure 10. Effects of different λ1 values on the induced magnetic field
表 1 磁性纳米颗粒的物理性质
Table 1. Physical properties of magnetic nanoparticles
ρ/(kg/m3) σ/(Ω·m)-1 Fe3O4 5 200 25 000 Fe2O3 5 180 10-5.99 Fe 7 870 9.93×106 Co 8 900 6.24×106 
下载: 导出CSV
-
[1] 庄昕, 刘付军, 孙艳萍, 等. 非等温黏弹性聚合物流体圆柱绕流的高精度数值模拟[J]. 应用数学和力学, 2022, 43(12): 1380-1391. doi: 10.21656/1000-0887.430127ZHUANG Xin, LIU Fujun, SUN Yanping, et al. High accuracy numerical simulation of non-isothermal viscoelastic polymer fluid past a cylinder[J]. Applied Mathematics and Mechanics, 2022, 43(12): 1380-1391. (in Chinese)) doi: 10.21656/1000-0887.430127 [2] HUMINIC G, HUMINIC A. Application of nanofluids in heat exchangers: a review[J]. Renewable and Sustainable Energy Reviews, 2012, 16(8): 5625-5638. doi: 10.1016/j.rser.2012.05.023 [3] RAMEZANIZADEH M, NAZARI M A, AHMADI M H, et al. Application of nanofluids in thermosyphons: a review[J]. Journal of Molecular Liquids, 2018, 272: 395-402. doi: 10.1016/j.molliq.2018.09.101 [4] ZAINAL N A, NAZAR R, NAGANTHRAN K, et al. Unsteady EMHD stagnation point flow over a stretching/shrinking sheet in a hybrid Al2O3-Cu/H2O nanofluid[J]. International Communications in Heat and Mass Transfer, 2021, 123: 105205. doi: 10.1016/j.icheatmasstransfer.2021.105205 [5] SHEIKHOLESLAMI M, RASHIDI M M, GANJI D D. Effect of non-uniform magnetic field on forced convection heat transfer of Fe3O4-water nanofluid[J]. Computer Methods in Applied Mechanics and Engineering, 2015, 294: 299-312. doi: 10.1016/j.cma.2015.06.010 [6] 赵芳彪. 非均匀磁场下磁液液滴生成与输运的实验研究[D]. 昆明: 昆明理工大学, 2019.ZHAO Fangbiao. Experimental study on droplet formation and transport of magnetic liquid under inhomogeneous magnetic field[D]. Kunming: Kunming University of Science and Technology, 2019. (in Chinese) [7] SHEIKHOLESLAMI M, SEYEDNEZHAD M. Nanofluid heat transfer in a permeable enclosure in presence of variable magnetic field by means of CVFEM[J]. International Journal of Heat and Mass Transfer, 2017, 114: 1169-1180. doi: 10.1016/j.ijheatmasstransfer.2017.07.018 [8] SHAKER H, ABBASALIZADEH M, KHALILARYA S, et al. Two-phase modeling of the effect of non-uniform magnetic field on mixed convection of magnetic nanofluid inside an open cavity[J]. International Journal of Mechanical Sciences, 2021, 207: 106666. doi: 10.1016/j.ijmecsci.2021.106666 [9] 翟梦情, 李琦, 郑素佩. 求解一维理想磁流体方程的移动网格熵稳定格式[J]. 计算力学学报, 2023, 40(2): 229-236.ZHAI Mengqing, LI Qi, ZHENG Supei. A moving-grid entropy stable scheme for the 1D ideal MHD equations[J]. Chinese Journal of Computational Mechanics, 2023, 40(2): 229-236. (in Chinese)) [10] BÉG O A, BAKIER A Y, PRASAD V R, et al. Nonsimilar, laminar, steady, electrically-conducting forced convection liquid metal boundary layer flow with induced magnetic field effects[J]. International Journal of Thermal Sciences, 2009, 48(8): 1596-1606. doi: 10.1016/j.ijthermalsci.2008.12.007 [11] HAYAT T, AJAZ U, KHAN S A, et al. Entropy optimized radiative flow of viscous nanomaterial subject to induced magnetic field[J]. International Communications in Heat and Mass Transfer, 2022, 136: 106159. doi: 10.1016/j.icheatmasstransfer.2022.106159 [12] DU M, WANG Z, HU H. Measuring memory with the order of fractional derivative[J]. Scientific Reports, 2013, 3(1): 1-3. [13] 杨旭, 梁英杰, 孙洪广, 等. 空间分数阶非Newton流体本构及圆管流动规律研究[J]. 应用数学和力学, 2018, 39(11): 1213-1226. doi: 10.21656/1000-0887.390153YANG Xu, LIANG Yingjie, SUN Hongguang, et al. A study on the constitutive relation and the flow of spatial fractional non-Newtonian fluid in circular pipes[J]. Applied Mathematics and Mechanics, 2018, 39(11): 1213-1226. (in Chinese)) doi: 10.21656/1000-0887.390153 [14] ZHAO J, ZHENG L, CHEN X, et al. Unsteady Marangoni convection heat transfer of fractional Maxwell fluid with Cattaneo heat flux[J]. Applied Mathematical Modelling, 2017, 44: 497-507. doi: 10.1016/j.apm.2017.02.021 [15] CHECHKIN A V, GORENFLO R, SOKOLOV I M. Retarding subdiffusion and accelerating super diffusion governed by distributed-order fractional diffusion equations[J]. Physical Review E, 2002, 66(4): 046129. doi: 10.1103/PhysRevE.66.046129 [16] YANG S, LIU L, LONG Z, et al. Unsteady natural convection boundary layer flow and heat transfer past a vertical flat plate with novel constitution models[J]. Applied Mathematics Letters, 2021, 120: 107335. doi: 10.1016/j.aml.2021.107335 [17] YANG W, CHEN X, ZHANG X, et al. Flow and heat transfer of viscoelastic fluid with a novel space distributed-order constitution relationship[J]. Computers & Mathematics With Applications, 2021, 94: 94-103. [18] LONG Z, LIU L, YANG S, et al. Analysis of Marangoni boundary layer flow and heat transfer with novel constitution relationships[J]. International Communications in Heat and Mass Transfer, 2021, 127: 105523. doi: 10.1016/j.icheatmasstransfer.2021.105523 [19] LIU L, FENG L, XU Q, et al. Flow and heat transfer of generalized Maxwell fluid over a moving plate with distributed order time fractional constitutive models[J]. International Communications in Heat and Mass Transfer, 2020, 116: 104679. doi: 10.1016/j.icheatmasstransfer.2020.104679 [20] MING C Y, LIU F W, ZHENG L C, et al. Analytical solutions of multi-term time fractional differential equations and application to unsteady flows of generalized viscoelastic fluid[J]. Computers & Mathematics With Applications, 2016, 72: 2084-2097. [21] ALHARBI K A M, SHAHMIR N, RAMZAN M, et al. Combined impacts of low oscillating magnetic field and Shliomis theory on mono and hybrid nanofluid flows with nonlinear thermal radiation[J]. Nanotechnology, 2023, 34(32): 325402. doi: 10.1088/1361-6528/acd38b [22] LANJWANI H B, CHANDIO M S, MALIK K, et al. Stability analysis of boundary layer flow and heat transfer of Fe2O3 and Fe-water base nanofluid over a stretching/shrinking sheet with radiation effect[J]. Engineering, Technology & Applied Science Research, 2022, 12(1): 8114-8122. [23] LIU F W, ZHUANG P, ANH V, et al. Stability and convergence next term of the difference methods for the space-time fractional advection-diffusion equation[J]. Applied Mathematical and Computation, 2007, 191: 12-20. doi: 10.1016/j.amc.2006.08.162 [24] 郭柏灵, 蒲学科, 黄凤辉. 分数阶偏微分方程及其数值解[M]. 北京: 科学出版社, 2011.GUO Bailing, PU Xueke, HUANG Fenghui. Fractional Partial Differential Equations and Their Numerical Solutions[M]. Beijing: Science Press, 2011. (in Chinese) -
图(10) / 表(1)
计量
- 文章访问数: 289
- HTML全文浏览量: 162
- PDF下载量: 60
- 被引次数: 0
渝公网安备50010802005915号