多孔介质中单相气体局部流动的均质化建模

李树光; 曲凯

doi:10.21656/1000-0887.440246

多孔介质中单相气体局部流动的均质化建模

doi: 10.21656/1000-0887.440246

李树光^,,
曲凯

大连海事大学理学院，辽宁大连 116026

基金项目:

辽宁省博士科研启动基金计划 2022-BS-093

详细信息

通讯作者:
李树光(1990—)，男，副教授，博士，硕士生导师(通讯作者. E-mail: shuguangli2008@126.com)

中图分类号: O3
计量
- 文章访问数: 377
- HTML全文浏览量: 118
- PDF下载量: 44
- 被引次数: 0
出版历程
- 收稿日期: 2023-08-17
- 修回日期: 2023-11-12
- 刊出日期: 2024-02-01

Homogenization Modeling of Single-Phase Gas Local Flow in Porous Media

LI Shuguang^,,
QU Kai

School of Science, Dalian Maritime University, Dalian, Liaoning 116026, P.R.China

摘要

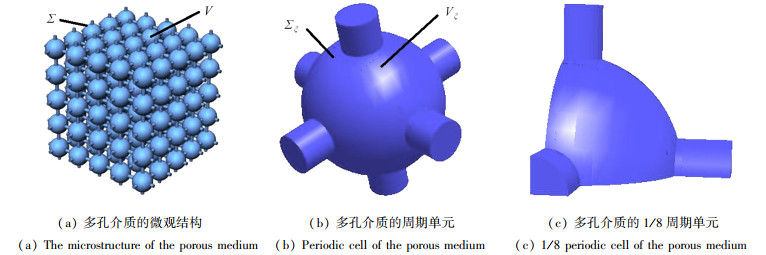
摘要: 该文研究了渐近均质法在单相气体渗流理论中的应用，开发了气体在孔隙尺度下流动的数学模型和数值方法. 基于渐近均质法，建立了周期单元上描述周期性多孔结构孔隙尺度下单相气体流动的局部问题. 讨论了局部问题的特殊数学性质和物理意义. 利用一种基于对称性和反对称性扩展的简化方法，提出了求解局部问题的最小二乘有限元方法，克服了由于平均算子和周期性边界条件引起的数值困难. 局部问题的求解能够获得单孔内速度和压力的精确分布，并且在仅知道孔隙几何形状的情况下评估多孔介质的渗透性. 在局部问题的基础上，通过理论分析获得了微管中Poiseuille流动的解析解，验证了所提出的数学模型和数值算法. 最后，考虑了一种三维周期性多孔结构，获得了单孔中气体局部流动的数值结果和多孔介质的渗透系数.
- 渐近均质法 /
- 多孔介质 /
- 局部流动 /
- 渗透性 /
- 最小二乘有限元
Abstract: The application of asymptotic homogenization method was investigated based on the filtration theory for single-phase gas, and the mathematical model and numerical method for the gas flow at the pore scale were developed. With the asymptotic homogenization method, a local problem of periodic cells was established to describe the local flow process of a single-phase gas at the pore scale of the periodic porous medium. The special mathematical properties and physical significance of the local problem were discussed. With a simplified approach based on symmetric and antisymmetric extensions, a least squares finite element method for the local problem was proposed, to overcome the numerical difficulties due to averaging operators and periodic boundary conditions. The solution of the local problem was obtained with accurate local velocity and pressure distributions in a single pore, and with gas permeability evaluation of porous media only in knowledge of the pore geometry. Beyond the local problem, the analytical solution of the Poiseuille flow in microtubes was obtained through theoretical analysis, to verify the proposed mathematical model and the numerical algorithm. Finally, a 3D periodic porous structure was considered, and numerical results of local flow in a single pore and permeability coefficients in porous media were obtained.
- asymptotic homogenization method /
- porous medium /
- local flow /
- permeability /
- least squares finite element

HTML全文

图 1 多孔介质的几何模型(显示为气体所占的孔隙)

Figure 1. Geometric modeling of the porous medium (showing the porosity occupied by gas)

下载: 全尺寸图片幻灯片

图 2 对称或反对称扩展中局部问题L⁽¹⁾的解的符号变化

Figure 2. Symbolic changes of solutions to local problem L⁽¹⁾ in symmetric or antisymmetric extensions

下载: 全尺寸图片幻灯片

图 3 圆柱形单通道结构的1/8周期单元

Figure 3. The 1/8 periodic cell of the cylindrical single channel structure

下载: 全尺寸图片幻灯片

图 4 单通道结构中Poiseuille流动的数值解及其与精确解比较

(a) Poiseuille流动的数值解(b) 与精确解的比较

Figure 4. The numerical solution of the Poiseuille flow in single channel structure, in comparison with the exact solution

(a) The numerical solution of the Poiseuille flow (b) Comparison of numerical and exact solutions

下载: 全尺寸图片幻灯片

图 5 局部问题L⁽¹⁾的数值结果

注为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.

Figure 5. Numerical results of local problem L⁽¹⁾

下载: 全尺寸图片幻灯片

表 1 多孔介质孔隙率和渗透系数的计算结果

Table 1. Calculation results of the porosity and the permeability coefficient of porous media

	local problem L⁽¹⁾	local problem L⁽²⁾	local problem L⁽³⁾
permeability	0.000 232 222 744 711	0.000 231 967 234 967	0.000 232 933 436 047
porosity	0.204 745 216 893 771	0.204 745 216 893 771	0.204 745 216 893 771

下载: 导出CSV

参考文献(18)

[1]	BEAR J. Modeling Phenomena of Flow and Transport in Porous Media[M]. Springer, 2018.
[2]	姜海龙, 朱培旺, 徐东华. 考虑气体加速效应的高压气井产能方程推导及其应用[J]. 应用数学和力学, 2020, 41(2): 134-142. doi: 10.21656/1000-0887.400030 JIANG Hailong, ZHU Peiwang, XU Donghua. Derivation and application of productivity equations for high-pressure gas reservoirs with gas acceleration effects[J]. Applied Mathematics and Mechanics, 2020, 41(2): 134-142. (in Chinese) doi: 10.21656/1000-0887.400030
[3]	孔祥言. 高等渗流力学[M]. 3版. 合肥: 中国科学技术大学出版社, 2020. KONG Xiangyan. Advanced Mechanics of Fluids in Porous Media[M]. 3rd ed. Hefei: University of Science and Technology of China Press, 2020. (in Chinese)
[4]	AURIAULT J L, BOUTIN C, GEINDREAU C. Homogenization of Coupled Phenomena in Heterogenous Media[M]. John Wiley, 2009.
[5]	BLUNT M J, JACKSON M D, PIRI M. Detailed physics, predictive capabilities and macroscopic consequences for pore-network models of multiphase flow[J]. Advances in Water Resources, 2002, 25(8/12): 1069-1089.
[6]	朱帅润, 李绍红, 钟彩尹, 等. 时间分数阶的非饱和渗流数值分析及其应用[J]. 应用数学和力学, 2022, 43(9): 966-975. doi: 10.21656/1000-0887.420334 ZHU Shuairun, LI Shaohong, ZHONG Caiyin, et al. Numerical analysis of time fractional-order unsaturated flow and its application[J]. Applied Mathematics and Mechanics, 2022, 43(9): 966-975. (in Chinese) doi: 10.21656/1000-0887.420334
[7]	BAKHVALOV N S, PANASENKO G. Homogenisation: Averaging Processes in Periodic Media: Mathematical Problems in the Mechanics of Composite Materials[M]. Springer, 2012.
[8]	DIMITRIENKO Y I. Thermomechanics of Composites Under High Temperatures[M]. Springer, 2016.
[9]	CAI Y, XU L, CHENG G D. Novel numerical implementation of asymptotic homogenization method for periodic plate structures[J]. International Journal of Solids and Structures, 2014, 51(1): 284-292. doi: 10.1016/j.ijsolstr.2013.10.003
[10]	李鸿鹏, 凌松, 戚振彪, 等. 热力耦合问题数学均匀化方法的计算精度[J]. 应用数学和力学, 2020, 41(1): 54-69. https://www.cnki.com.cn/Article/CJFDTOTAL-YYSX202001005.htm LI Hongpeng, LING Song, QI Zhenbiao, et al. Accuracy of the mathematical homogenization method for thermomechanical problems[J]. Applied Mathematics and Mechanics, 2020, 41(1): 54-69. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-YYSX202001005.htm
[11]	LI S G, DIMITRIENKO Y I. Mathematical modeling for the local flow of a generalized Newtonian fluid in 3D porous media[J]. Applied Mathematical Modelling, 2022, 105: 551-565. doi: 10.1016/j.apm.2022.01.003
[12]	DIMITRIENKO Y I, DIMITRIENKO I D. Simulation of local transfer in periodic porous media[J]. European Journal of Mechanics B: Fluids, 2013, 37: 174-179. doi: 10.1016/j.euromechflu.2012.09.006
[13]	WANG J G, LEUNG C F, CHOU Y K. Numerical solutions for flow in porous media[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2003, 27(7): 565-583 doi: 10.1002/nag.286
[14]	XU W, FISH J. A multiscale modeling of permeability in a multi-porosity porous medium using smoothed particle hydrodynamics[J]. International Journal for Numerical Methods in Engineering, 2017, 111(8): 776-800. doi: 10.1002/nme.5494
[15]	CAI Z, LEE B, WANG P. Least-squares methods for incompressible Newtonian fluid flow: linear stationary problems[J]. SIAM Journal on Numerical Analysis, 2004, 42(2): 843-859. doi: 10.1137/S0036142903422673
[16]	LI S G, DIMITRIENKO Y I. Least squares finite element simulation of local transfer for a generalized Newtonian fluid in 2D periodic porous media[J]. Journal of Non-Newtonian Fluid Mechanics, 2023, 316: 105032. doi: 10.1016/j.jnnfm.2023.105032
[17]	PAYETTE G S, REDDY J N. On the roles of minimization and linearization in least squares finite element models of nonlinear boundary-value problems[J]. Journal of Computational Physics, 2011, 230(9): 3589-3613. doi: 10.1016/j.jcp.2011.02.002
[18]	吴望一. 流体力学[M]. 2版. 北京: 北京大学出版社, 2021. WU Wangyi. Fluid Mechanics[M]. 2nd ed. Beijing: Peking University Press, 2021. (in Chinese)