Research on Mechanical Properties of Negative Stiffness Torsion Metastructures
-
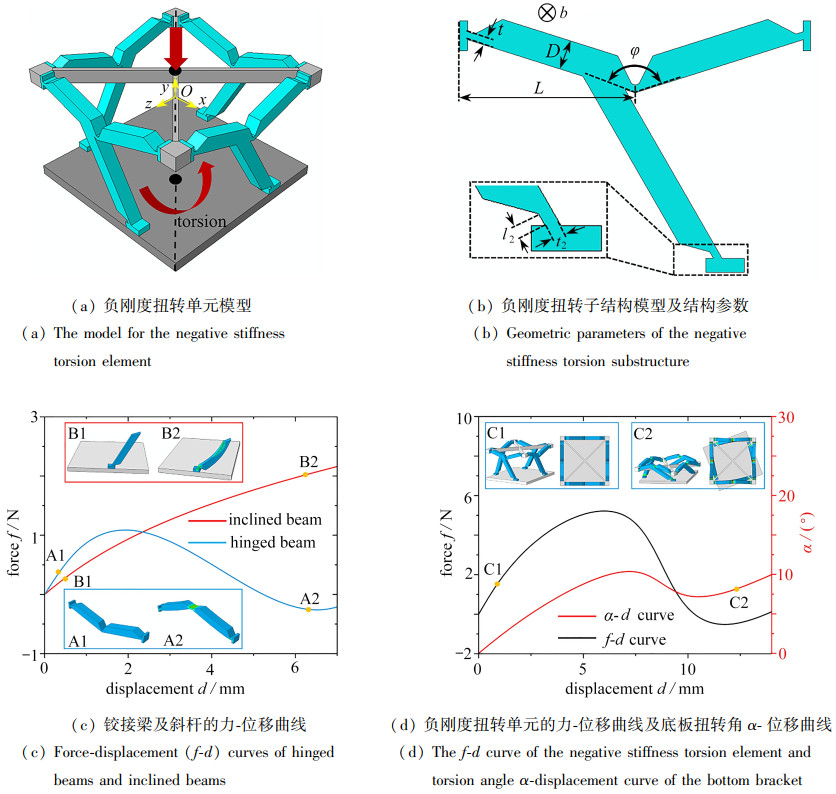
摘要: 通过屈曲变形实现非损伤耗散能量的负刚度超结构，为可重复使用的缓冲防护器件提供了新的设计思路，但其耗散能力较弱、难以过载保护的缺点限制了实际应用. 为增强耗能性能及最大允许变形量，将负刚度铰接梁与具有压扭效应的斜杆串联组合，设计了一种负刚度扭转超结构，通过引入扭转变形缓解了过载导致的应力集中. 建立了负刚度扭转单元模型，通过刚度匹配设计实现了对力学性能的调控，使负刚度扭转超结构表现出突跳行为，产生加卸载曲线不重合的迟滞现象，从而极大地提高了能量耗散能力. 通过结构参数及刚度关系的优化设计，负刚度扭转超结构的最大等效压缩应变可达71%，相同层数下，能量耗散能力可以达到传统屈曲梁超结构的两倍.Abstract: The negative stiffness metastructures provide a novel design strategy for reusable protective devices with the non-damage buckling energy dissipation mechanisms. However, the weak cushioning capacity and the measly overloading protection restrict the practical applications. To enhance the energy dissipation and maximum allowable deformation, a negative stiffness torsion metastructure was developed with substructures including buckling hinged beams and inclined beams. Through introduction of compression-torsion coupling effects, the stress concentration caused by overload can be alleviated. Based on a series model for the negative stiffness torsion element, a strategy to control the mechanical properties was proposed through design of the matching relations of stiffnesses. Snap-through behaviors and hysteresis phenomena can be obtained on the non-overlapping loading and unloading curves, to greatly improve the energy dissipation capacity. The optimization of geometric parameters and stiffness relations increases the maximum equivalent compressive strain of the negative stiffness torsion metastructure by 71%. Additionally, compared to the traditional buckling beam metastructures with the same number of layers, the negative stiffness torsion metastructure can double in the energy dissipation capacity.
-
Key words:
- negative stiffness /
- torsion metastructure /
- series design /
- energy dissipation
-
图 1 负刚度扭转单元力学响应
注 为了解释图中的颜色，读者可以参考本文的电子网页版本，后同.
Figure 1. Mechanical responses of negative stiffness torsion elements
图 2 刚度匹配关系对串联单元力学响应的影响
Figure 2. The influences of stiffness matching relations on the mechanical responses of series elements
图 4 刚度匹配关系对串联单元突跳行为的影响
Figure 4. The influences of stiffness matching relations on the snap-through behavior of series elements
图 8 不同刚度匹配关系对负刚度扭转单元力学响应的影响
Figure 8. Mechanical responses of negative stiffness torsion elements with different stiffness matching relations
图 9 负刚度扭转超结构制备及装配
Figure 9. Fabrication and assembling of negative stiffness torsion metastructures
图 10 不同铰接梁参数t/L下负刚度扭转超结构实验与仿真结果
Figure 10. Experiment and simulation results of negative stiffness torsion elements/metastructures with different t/L values
图 11 不同斜杆参数t2/l2下负刚度扭转超结构实验与仿真结果
Figure 11. Experiment and simulation results of negative stiffness torsion elements/metastructures with different t2/l2 values
图 13 负刚度扭转超结构压缩前后示意图
Figure 13. The diagram of the negative stiffness torsion metastructure before and after compression
表 1 串联单元Ⅰ至Ⅶ的几何参数及负刚度
Table 1. The geometric parameters and negative stiffnesses of (No. Ⅰ~Ⅶ) series elements
No. t t/L D/L φ/(°) K2/(N/mm) K4/(N/mm) Y Ⅰ 1 0.044 0.17 145 -0.428 0.2 0.467 Ⅱ 1 0.044 0.17 150 -0.318 0.2 0.628 Ⅲ 1 0.044 0.17 155 -0.219 0.2 0.913 Ⅳ 1 0.044 0.17 160 -0.125 0.2 1.600 Ⅴ 0.45 0.02 0.17 150 -0.273 0.2 0.732 Ⅵ 1.35 0.06 0.17 150 -0.325 0.2 0.615 Ⅶ 1.80 0.08 0.17 150 -0.375 0.2 0.532 
下载: 导出CSV
表 2 负刚度扭转子结构尺寸参数及刚度
Table 2. The geometric parameters and stiffnesses of negative stiffness torsion metastructures
No. t t2 t/L t2/l2 φ/(°) K2/(N/mm) K4/(N/mm) U1 0.54 1 0.024 1 145 -0.235 0.35 U2 1.125 1 0.050 1 145 -0.455 0.35 U3 1 0.6 0.044 1 145 -0.428 0.29 U4 1 2.6 0.044 4.3 145 -0.428 0.38
下载: 导出CSV
表 3 双层负刚度超结构耗散性能对比
Table 3. Comparisons of the dissipation performances of double-layer negative stiffness metastructures
下载: 导出CSV
表 4 负刚度扭转超结构的最大等效压缩应变
Table 4. Maximum allowable compressive displacements of negative stiffness torsion metastructures
No. H1/mm H2/mm $\tilde{\varepsilon}$/% U1 72 26 64 U2 72 27 62 U3 72 21 71 U4 72 28 61
下载: 导出CSV
-
[1] DARWISH Y, ELGAWADY M A. Numerical and experimental investigation of negative stiffness beams and honeycomb structures[J]. Engineering Structures, 2024, 301: 117163. doi: 10.1016/j.engstruct.2023.117163 [2] LI X Y, WANG J X, CHAI Y J, et al. A novel frog-like meta-structure with linkage mechanism for low-frequency vibration isolation[J]. Journal of Physics D: Applied Physics, 2024, 57: 135304. doi: 10.1088/1361-6463/ad1851 [3] 杨航, 马力. 多材料点阵结构的热可编程力学行为[J]. 应用数学和力学, 2022, 43(5): 534-552.YANG Hang, MA Li. Multimaterial lattice structures with thermally programmable mechanical behaviors[J]. Applied Mathematics and Mechanics, 2022, 43(5): 534-522. (in Chinese) [4] 王竞哲, 陈保才, 朱绍伟, 等. 圆锥形负刚度超材料吸能性能研究[J]. 应用数学和力学, 2023, 44(10): 1172-1179.WANG Jingzhe, CHEN Baocai, ZHU Shaowei, et al. Study on energy absorption performances of conical negative stiffness metamaterials[J]. Applied Mathematics and Mechanics, 2023, 44(10): 1172-1179. (in Chinese) [5] VALENCIA C, RESTREPO D, MANKAME N D, et al. Computational characterization of the wave propagation behavior of multi-stable periodic cellular materials[J]. Extreme Mechanics Letters, 2019, 33(C): 100565. [6] GOLDSBERRY B M, HABERMAN M R. Negative stiffness honeycombs as tunable elastic metamaterials[J]. Journal of Applied Physics, 2018, 123(9): 091711. doi: 10.1063/1.5011400 [7] FRAZIER M J. Multi-stable acoustic metamaterials with re-configurable mass distribution[J]. Journal of Applied Physics, 2022, 131(16): 165105. doi: 10.1063/5.0086214 [8] HU N, LI B, BAI R Y, et al. A torsion-bending antagonistic bistable actuator enables untethered crawling and swimming of miniature robots[J]. Research, 2023, 6: 0116. doi: 10.34133/research.0116 [9] MUNGEKAR M, MA L X, YAN W Z, et al. Design of bistable soft deployable structures via a kirigami-inspired planar fabrication approach[J]. Advanced Materials Technologies, 2023, 8(16): 00088. [10] CHI Y D, HONG Y Y, ZHAO Y, et al. Snapping for high-speed and high-efficient butterfly stroke-like soft swimmer[J]. Science Advances, 2022, 8(46): eadd3788. doi: 10.1126/sciadv.add3788 [11] WANG J, ZHAO T H, FAN Y Y, et al. Leveraging bioinspired structural constraints for tunable and programmable snapping dynamics in high-speed soft actuators[J]. Advanced Functional Materials, 2022, 33(2): 09798. [12] ZHOU S X, CAO J Y, ERTURK A, et al. Enhanced broadband piezoelectric energy harvesting using rotatable magnets[J]. Applied Physics Letters, 2013, 102(17): 173901. doi: 10.1063/1.4803445 [13] ZHOU S X, CAO J Y, INMAN D J, et al. Broadband tristable energy harvester: modeling and experiment verification[J]. Applied Energy, 2014, 133: 33-39. doi: 10.1016/j.apenergy.2014.07.077 [14] BARTON DAW, BURROW S G, CLARE L R. Energy harvesting from vibrations with a nonlinear oscillator[J]. Journal of Vibration and Acoustics, 2010, 132(2): 427-436. [15] SHAN S C, KANG S H, RANEY J R, et al. Multistable architected materials for trapping elastic strain energy[J]. Advanced Materials, 2015, 27(29): 4296-4301. doi: 10.1002/adma.201501708 [16] FRENZEL T, FINDISEN C, KADIC M, et al. Tailoredbuckling microlattices as reusable light-weight shock absorbers[J]. Advanced Materials, 2016, 28(28): 5865-5870. doi: 10.1002/adma.201600610 [17] WANG B, TAN X J, ZHU S W, et al. Cushion performance of cylindrical negative stiffness structures: analysis and optimization[J]. Composite Structures, 2019, 227: 111276. doi: 10.1016/j.compstruct.2019.111276 [18] ZHANG Y, TICHEM M, VAN KEULEN F. A novel design of multi-stable metastructures for energy dissipation[J]. Materials Design, 2021, 212: 110234. doi: 10.1016/j.matdes.2021.110234 [19] TAN X J, WANG L C, ZHU S W, et al. A general strategy for performance enhancement of negative stiffness mechanical metamaterials[J]. European Journal of Mechanics A: Solids, 2022, 96: 104702. doi: 10.1016/j.euromechsol.2022.104702 [20] MENG Z Q, OUYANG Z, CHEN C Q. Multi-step metamaterials with two phases of elastic and plastic deformation[J]. Composite Structures, 2021, 271: 114152. doi: 10.1016/j.compstruct.2021.114152 [21] SHI J H, MOFATTEH H, MIRABOLGHASEMI A, et al. Programmable multistable perforated shellular[J]. Advanced Materials, 2021, 33(42): 210243. [22] LIU S H, AZAD A, BURGUENO R. Architected materials for tailorable shear behavior with energy dissipation[J]. Extreme Mechanics Letters, 2019, 28: 1-7. doi: 10.1016/j.eml.2019.01.010 [23] CHEN S, WANG B, ZHU S W, et al. A novel composite negative stiffness structure for recoverable trapping energy[J]. Composites Part A, 2020, 129: 105697. doi: 10.1016/j.compositesa.2019.105697 -
计量
- 文章访问数: 239
- HTML全文浏览量: 102
- PDF下载量: 51
- 被引次数: 0
渝公网安备50010802005915号