变厚度连续纤维增强复合材料铺层设计优化方法

杜晨; 彭雄奇

doi:10.21656/1000-0887.420410

变厚度连续纤维增强复合材料铺层设计优化方法

doi: 10.21656/1000-0887.420410

杜晨,
彭雄奇^,

上海交通大学材料科学与工程学院，上海 200030

基金项目: 国家自然科学基金(U20A20288；11972225)

详细信息

作者简介:
杜晨（1999—），男，硕士生（E-mail：ChenDu@sjtu.edu.cn）

彭雄奇（1970—），男，教授，博士，博士生导师（通讯作者. E-mail：xqpeng@sjtu.edu.cn）

中图分类号: TB332; O39
计量
- 文章访问数: 782
- HTML全文浏览量: 223
- PDF下载量: 103
- 被引次数: 0
出版历程
- 收稿日期: 2021-12-28
- 修回日期: 2022-12-01
- 网络出版日期: 2022-12-08
- 刊出日期: 2022-12-01

Lamination Design Optimization for Continuous Fiber Reinforced Composites of Variable Thicknesses

DU Chen,
PENG Xiongqi^,

School of Materials Science and Engineering, Shanghai Jiao Tong University, Shanghai 200030, P.R.China

摘要

摘要:
由于具备高的比强度、比刚度，利用连续纤维增强复合材料代替传统金属材料以实现结构轻量化正受到设计者们的广泛关注。然而，结构的复杂性给复合材料的铺层设计与优化带来了很大的挑战。针对航空用复合材料铺层设计约束多的问题，通过逐步构建设计变量准确表达结构的铺层信息。基于经典遗传算法框架，结合各设计变量特点，定义了铺层优化算法中的遗传算子，通过引入“修复”策略保证了每一代解都能满足设计约束，分布在可行域区间内。最后利用精英保留策略提高了算法的局部寻优能力，可以降低复杂复合材料结构铺层设计的计算成本。通过解决经典benchmark问题并与已有优化结果的比较，验证了前述铺层优化算法的全局、局部寻优能力，为工程实际中的复合材料铺层设计优化提供了理论支撑。
- 连续纤维增强复合材料 /
- 铺层设计优化 /
- 遗传算法 /
- “修复”策略 /
- 寻优能力
Abstract:
Due to the high specific strength and stiffness, the use of continuous fiber reinforced composites instead of traditional metal materials to achieve structural lightweight has been widely considered by designers. However, the structural complexity brings great challenges to the design and optimization of composite lamination. Aimed at the problem of multiple constraints in the design of aviation composite laminates, the ply information of the structure was accurately expressed with gradually constructed design variables. Based on the classical genetic algorithm framework and the characteristics of all design variables, the genetic operators in the lamination optimization algorithm were defined, and the repair strategy was introduced to ensure that each generation of solutions could satisfy the design constraints and be distributed in the feasible region. Finally, the elite reservation strategy was used to improve the local optimization ability of the algorithm, which can reduce the computation cost of the lamination design of complex composite structures. Through the resolution of the classical benchmark problem and the comparison with the existing optimization results, the global and local optimization ability of the proposed lamination optimization algorithm was verified. The work provides theoretical supports for the optimization of composite lamination design in engineering practice.
- continuous fiber reinforced composites /
- layer design optimization /
- genetic algorithm /
- repair strategy /
- optimization ability

HTML全文

图 1 连续厚度变化层合板

注　为了解释图中的颜色，读者可以参考本文的电子网页版本，后同。

Figure 1. Continuous thickness varying laminates

下载: 全尺寸图片幻灯片

图 2 铺层顺序变量生成步骤

Figure 2. The generation steps of stacking sequence variables

下载: 全尺寸图片幻灯片

图 3 由S_ins和S_lam得到铺层角度信息

Figure 3. The ply angle information obtained from S_ins and S_lam

下载: 全尺寸图片幻灯片

图 4 双点交叉过程

Figure 4. The process of 2-point chiasma

下载: 全尺寸图片幻灯片

图 5 OX过程

Figure 5. The process of OX

下载: 全尺寸图片幻灯片

图 6 单点交叉过程

Figure 6. The process of single-point chiasma

下载: 全尺寸图片幻灯片

图 7 单点变异过程

Figure 7. The process of single-point mutation

下载: 全尺寸图片幻灯片

图 8 反转变异过程

Figure 8. The process of swap mutation

下载: 全尺寸图片幻灯片

图 9 用于变量S_lam的修复策略^[11]

Figure 9. The repair strategy for S_lam variable^[11]

下载: 全尺寸图片幻灯片

图 10 铺层优化流程

Figure 10. The layering optimization process

下载: 全尺寸图片幻灯片

图 11 经典benchmark问题算例^[5]

Figure 11. Classic examples of benchmark problems^[5]

下载: 全尺寸图片幻灯片

图 12 4种可选铺层角度下最小重量-进化代数收敛曲线

Figure 12. Minimum weight-generation convergence curves under 4 alternative ply angles

下载: 全尺寸图片幻灯片

图 13 12种可选铺层角度下最小重量-进化代数收敛曲线

Figure 13. Minimum weight-generation convergence curves under 12 alternative ply angles

下载: 全尺寸图片幻灯片

表 1 优化算法参数取值

Table 1. Optimization algorithm parameter values

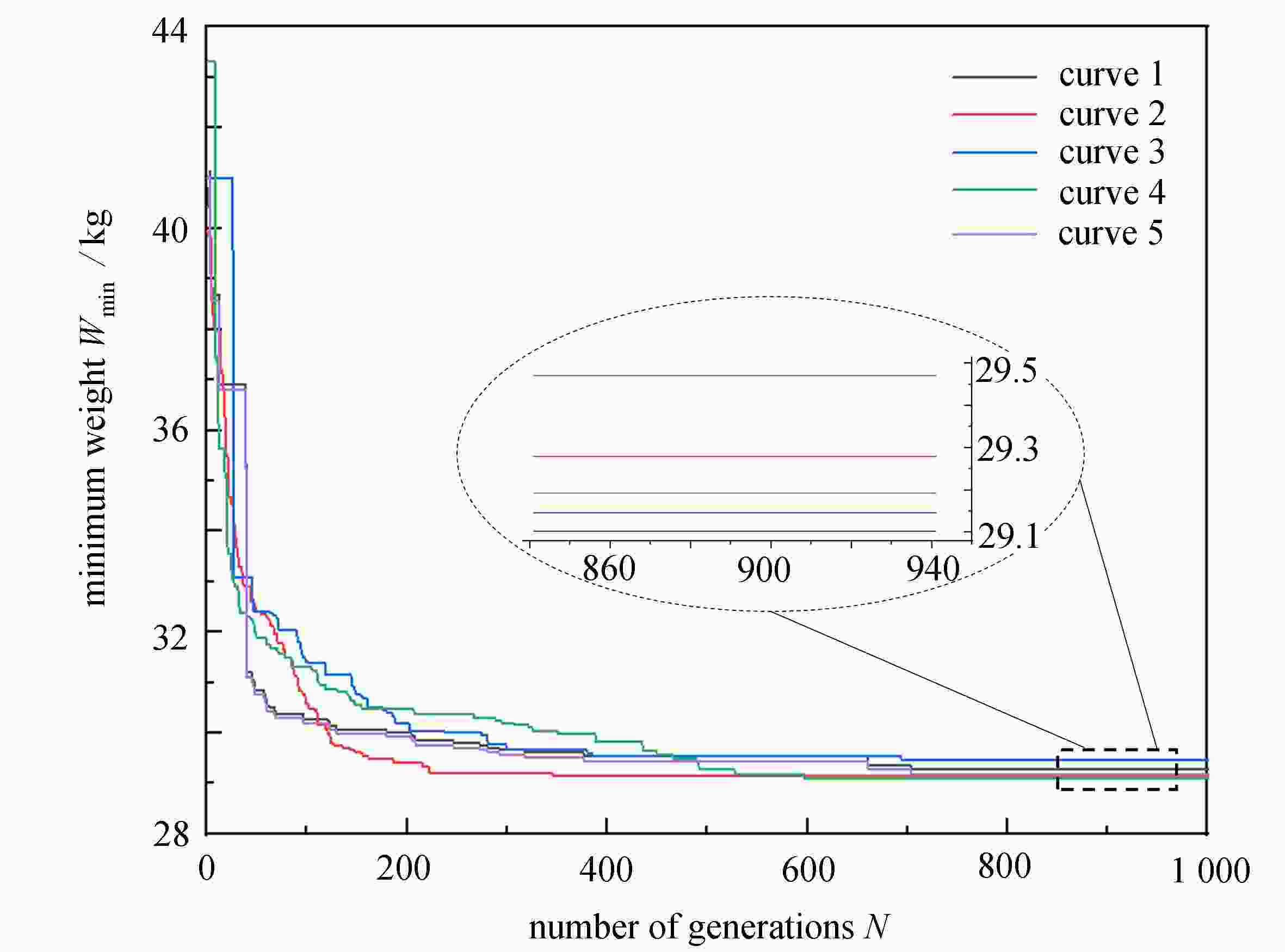
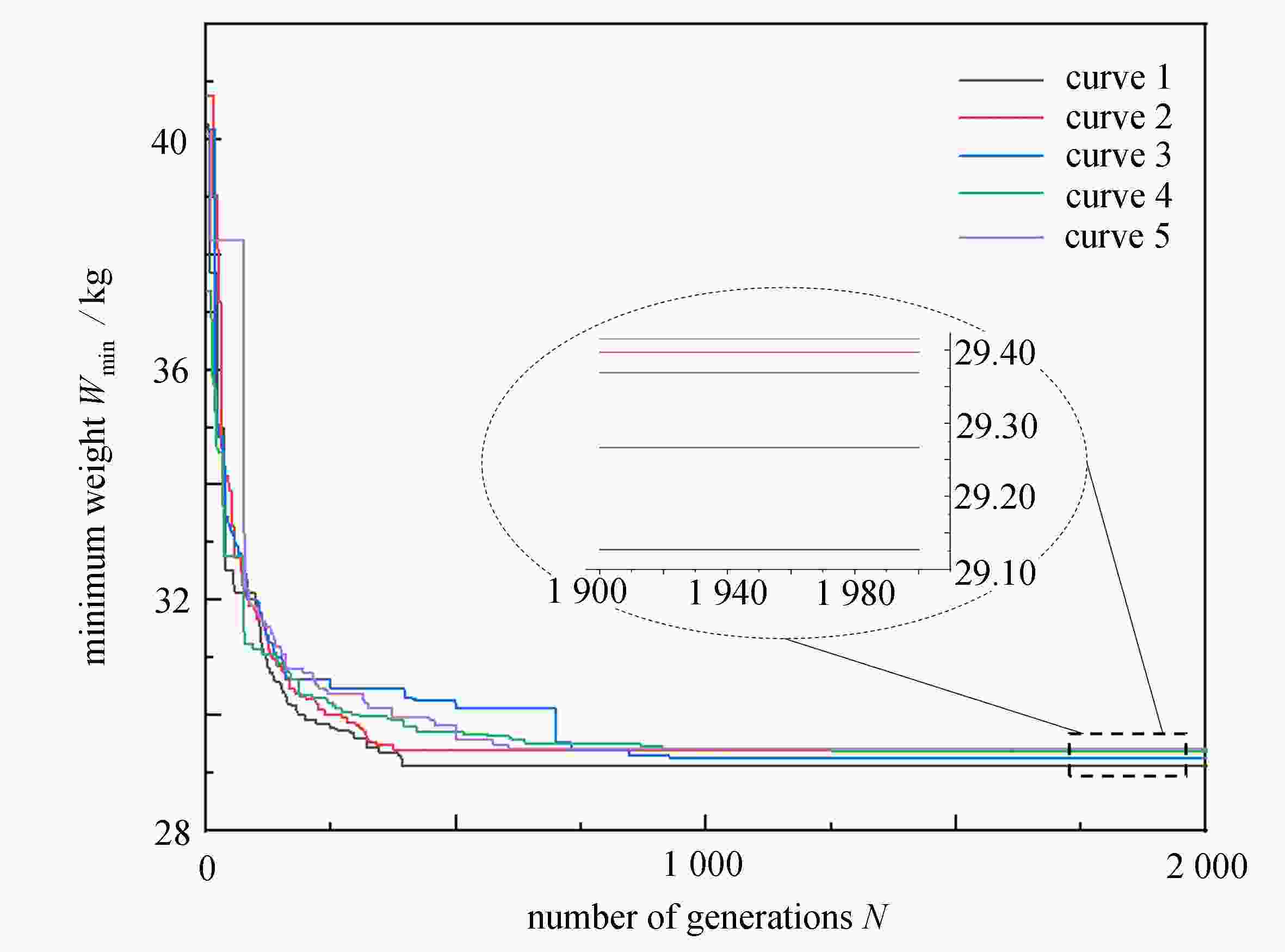
parameter	value
populationsize S	100
number of generations G	1000, 2 000
probability of chiasma P_c/%	0.9
probability of mutation P_m/%	0.1
elite reserved individual I	2
number of competition selections c	2

下载: 导出CSV

表 2 四种可选铺层角度下最优解变量取值

Table 2. Variable value of the optimal solution under 4 alternative ply angles

variable	variable value
N_str	[35 29 23 19 17 23 19 25 39 36 31 29 23 19 26 32 19 23]
final N_str	[34 29 21 19 16 22 19 25 39 36 31 29 22 19 26 32 19 23]
S_lam	[−45° 0° −45° 90° 45° 90° −45° −45° 0° −45° −45° 90° 45° 0° 45° 0° 0° 45° 0° 0° 45° 90° 45° 0°]
S_ins	[0 1 2 2 0 1 6 1 5 1 0 0 8 4 1 4 0 1 1 1 7 0 9 1 3 1 0 7 5 6 0 3]

下载: 导出CSV

表 3 与SST方法最优解的比较

Table 3. Comparison with the optimal solution of the SST method

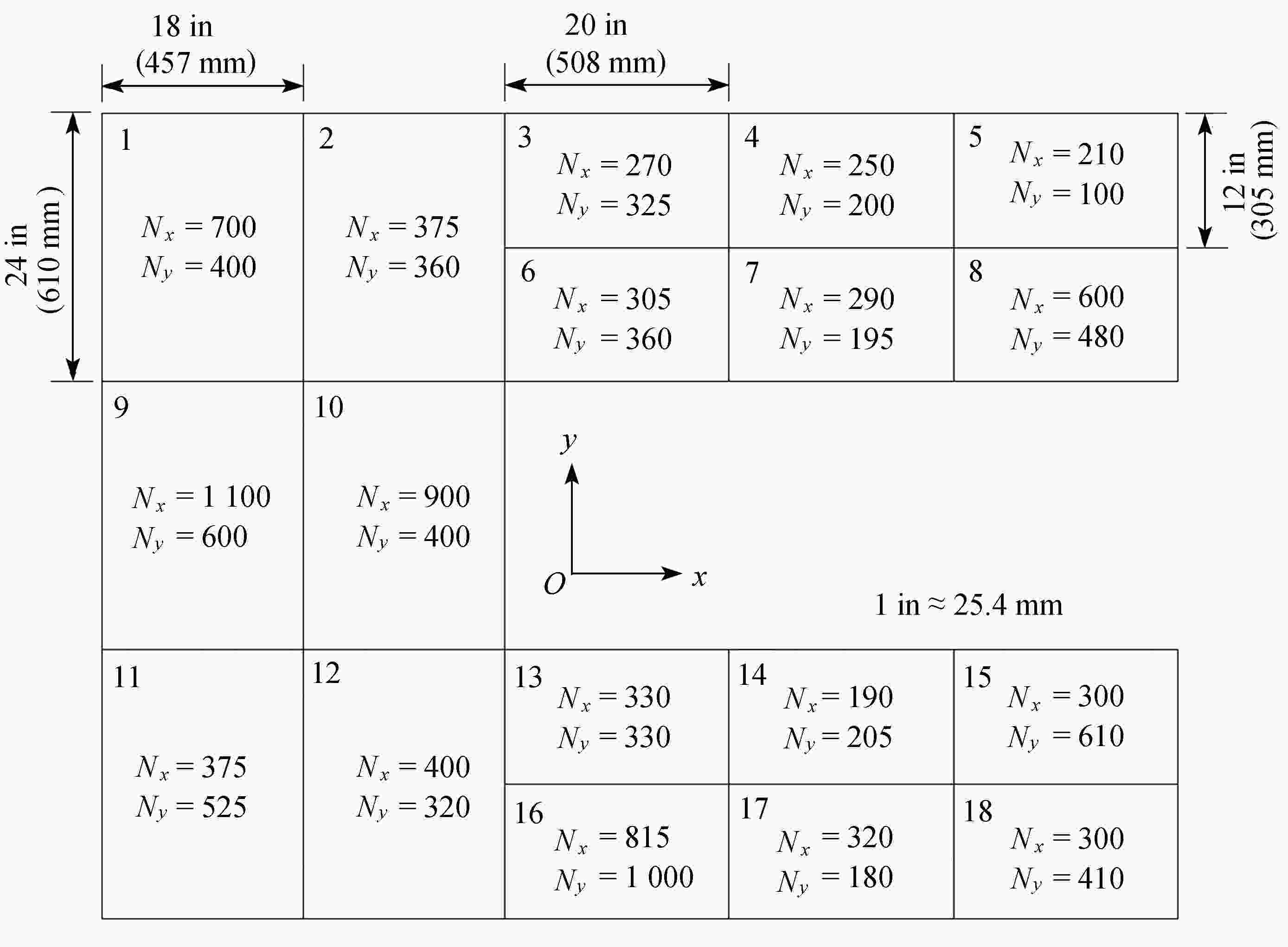
panel	this paper solution		SST
panel	number of plies N_plie	margin η/%	number of plies N_plie	margin η/%
1	34(0)	2.7	34	17.2
2	29(−1)	0.6	30	15.9
3	21(−1)	1.4	22	36.4
4	19( + 1)	9.6	18	13.3
5	16(−2)	10.3	18	59.3
6	22(0)	4.4	22	22.6
7	19( + 1)	6.2	18	9.8
8	25(−1)	2.8	26	31.9
9	39( + 1)	0.6	38	6.9
10	36(−2)	0.6	38	25.6
11	31( + 1)	5.7	30	10.0
12	29(−1)	0.1	30	27.1
13	22(0)	9.3	22	28.3
14	19( + 1)	16.3	18	20.2
15	26(0)	11.9	26	27.8
16	32( + 2)	3.2	30	6.8
17	19( + 1)	7.7	18	11.3
18	23( + 1)	8.0	22	11.2
number of total plies N_total	461		460
weight W/kg	28.829 1		28.85

下载: 导出CSV

表 4 12种可选铺层角度下最优解变量取值

Table 4. Variable values of the optimal solution under 12 alternative ply angles

variable	variable value
N_str	[34 29 21 19 16 22 19 25 40 36 31 31 22 19 27 32 19 23]
final N_str	[34 29 21 19 16 22 19 25 39 36 31 29 22 19 26 31 19 23]
S_lam	[90° −45° −30° 0° −30° 0° −45° −30° −60° −45° 90° 45° 30° 45° 60° 30° 60° 30° 45° 0° 30° 0° −30° 0°]
S_ins	[0 9 1 3 1 1 0 1 0 7 1 6 0 2 0 8 1 7 6 0 1 5 0 1 2 3 5 0 4 1 4 1]

下载: 导出CSV

表 5 与SST方法最优解的比较

Table 5. Comparison with the optimal solution of the SST method

panel	this paper solution		SST
panel	number of plies N_plie	margin η/%	number of plies N_plie	margin η/%
1	34(0)	5.8	34	17.2
2	29(− 1)	2.9	30	15.9
3	21(− 1)	1.4	22	36.4
4	19( + 1)	9.4	18	13.3
5	16(− 2)	8.6	18	59.3
6	22(0)	4.6	22	22.6
7	19( + 1)	6.0	18	9.8
8	25(− 1)	2.8	26	31.9
9	39( + 1)	4.6	38	6.9
10	36(− 2)	3.1	38	25.6
11	31( + 1)	10.5	30	10.0
12	29(− 1)	2.4	30	27.1
13	22(0)	9.5	22	28.3
14	19( + 1)	16.1	18	20.2
15	26(0)	11.7	26	27.8
16	31( + 1)	4.6	30	6.8
17	19( + 1)	7.5	18	11.3
18	23( + 1)	7.7	22	11.2
number of total plies N_total	460		460
weight W/kg	28.782 6		28.85

下载: 导出CSV

参考文献(11)

[1]	朱迪, 姚远, 彭雄奇. 碳纤维汽车底盘后纵臂CAE设计的优化算法[J]. 应用数学和力学, 2018, 39(8): 925-934 ZHU Di, YAO Yuan, PENG Xiongqi. An optimization algorithm for CAE design of carbon fiber reinforced composite chassis longitudinal arms[J]. Applied Mathematics and Mechanics, 2018, 39(8): 925-934.(in Chinese)
[2]	ZEHNDER N, ERMANNI P. A methodology for the global optimization of laminated composite structures[J]. Composite Structures, 2006, 72(3): 311-320. doi: 10.1016/j.compstruct.2005.01.021
[3]	KIM J S, KIM C G, HONG C S. Optimum design of composite structures with ply drop using genetic algorithm and expert system shell[J]. Composite Structures, 1999, 46(2): 171-187. doi: 10.1016/S0263-8223(99)00052-5
[4]	KRISTINSDOTTIR B P, ZABINSKY Z B, et al. Optimal design of large composite panels with varying loads[J]. Composite Structures, 2001, 51(1): 93-102. doi: 10.1016/S0263-8223(00)00128-8
[5]	SOREMEKUN G, GURDAL Z, KASSAPOGLOU C, et al. Stacking sequence blending of multiple composite laminates using genetic algorithms[J]. Composite Structures, 2002, 56(1): 53-62. doi: 10.1016/S0263-8223(01)00185-4
[6]	LIU D, TOROPOV V V, QUERIN O M, et al. Bi-level optimization of blended composite panels[J]. Journal of Aircraft, 2011, 48(1): 107-18. doi: 10.2514/1.C000261
[7]	YANG J, SONG B, ZHONG X, et al. Optimal design of blended composite laminate structures using ply drop sequence[J]. Composite Structures, 2016, 135: 30-37. doi: 10.1016/j.compstruct.2015.08.101
[8]	IRISARRI F X, LASSEIGNE A, LEROY F H, et al. Optimal design of laminated composite structures with ply drops using stacking sequence tables[J]. Composite Structures, 2014, 107: 559-569. doi: 10.1016/j.compstruct.2013.08.030
[9]	GHIASI H, PASINI D, LESSAED L. Optimum stacking sequence design of composite materials part Ⅰ: constant stiffness design[J]. Composite Structures, 2009, 90(1): 1-11. doi: 10.1016/j.compstruct.2009.01.006
[10]	张洁. 复合材料铺层设计准则的一些理解[J]. 科技创新导报, 2013(14): 57-58. ZHANG Jie. Some understandings of design guidelines for composite laminated layers[J]. Science and Technology Innovation Review, 2013(14): 57-58. (in Chinese)
[11]	FEDON N, WEAVER P M, PIRRERA A, et al. A repair algorithm for composite laminates to satisfy lay-up design guidelines[J]. Composite Structures, 2021, 259: 113448. doi: 10.1016/j.compstruct.2020.113448

施引文献

资源附件(0)

访问统计

图(13) / 表(5)

计量

文章访问数: 782
HTML全文浏览量: 223
PDF下载量: 103
被引次数: 0

姓名
邮箱
手机号码
标题
留言内容
验证码

留言板

变厚度连续纤维增强复合材料铺层设计优化方法

doi: 10.21656/1000-0887.420410

作者简介:
杜晨（1999—），男，硕士生（E-mail：ChenDu@sjtu.edu.cn）

彭雄奇（1970—），男，教授，博士，博士生导师（通讯作者. E-mail：xqpeng@sjtu.edu.cn）

计量

Lamination Design Optimization for Continuous Fiber Reinforced Composites of Variable Thicknesses

计量

目录

留言板

变厚度连续纤维增强复合材料铺层设计优化方法

doi: 10.21656/1000-0887.420410

作者简介: 杜晨（1999—），男，硕士生（E-mail：ChenDu@sjtu.edu.cn） 彭雄奇（1970—），男，教授，博士，博士生导师（通讯作者. E-mail：xqpeng@sjtu.edu.cn）

计量

出版历程

Lamination Design Optimization for Continuous Fiber Reinforced Composites of Variable Thicknesses

计量

出版历程

目录

作者简介:
杜晨（1999—），男，硕士生（E-mail：ChenDu@sjtu.edu.cn）

彭雄奇（1970—），男，教授，博士，博士生导师（通讯作者. E-mail：xqpeng@sjtu.edu.cn）