Finite Integral Transform Solutions for Free Vibrations of Rectangular Thin Plates With Mixed Boundary Constraints
edited-by
edited-by
(Contributed by LI Rui, M. AMM Editorial Board)-
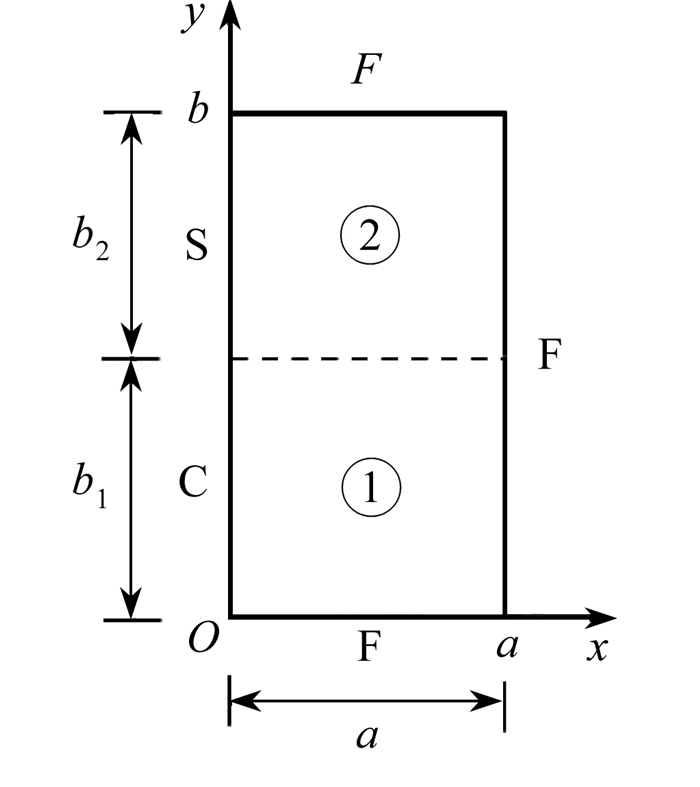
摘要: 解析解可以作为经验公式以及数值方法对比的基准、快速参数分析和优化的工具以及实验设计的理论依据，具有独特的研究价值，而传统解析方法(如Lévy解法)只能求解对边简支板壳的力学问题，对于复杂边界约束下的板壳力学问题难以获得解析解. 笔者等近年来发展了板壳力学问题的有限积分变换法，实现了非Lévy型板壳力学问题的求解，但仍无法直接求解由混合边界约束引起的板壳高阶偏微分方程复杂边值问题. 该文首次结合有限积分变换与子域分解方法，实现了混合边界约束下矩形薄板自由振动问题的解析求解. 首先根据混合边界约束将矩形板拆分为两部分，然后通过有限积分变换法对两部分分别进行求解，最后引入连续性条件，获得了原问题的解析解. 以工程中常见的边缘点焊悬臂板为背景，具体分析了一边固支-简支混合约束、其余三边自由的矩形薄板自由振动问题，获得的固有频率和振型结果均与有限元数值解及文献结果高度吻合，验证了该文推导和结果的准确性. 有限积分变换法的求解从基本控制方程出发，无需预先假设解的形式，因此是一种严格的分析方法，可以广泛求解以板壳力学问题为代表的高阶偏微分方程复杂边值问题.Abstract: Analytical solutions, with unique research value, can serve as benchmarks for empirical formulas and numerical methods, a tool for rapid parameter analysis and optimization, and a theoretical basis for experimental designs. Conventional analytical methods, e.g., the Lévy solution method, are only applicable to mechanical problems of plates and shells with opposite simply-supported edges, which, however, may fail to obtain analytical solutions for the issues with complex boundary constraints. In recent years, the finite integral transform method for plate and shell problems was developed to deal with non-Lévy-type plates and shells, but it is still infeasible to solve the mixed boundary constrains-induced complex boundary value problems of higher-order partial differential equations. Herein, for the first time, the finite integral transform method was combined with the sub-domain decomposition technique to solve the free vibrations of rectangular thin plates with mixed boundary constraints. The rectangular plate was first divided into 2 sub-domains according to the mixed boundary constraints, and the 2 sub-domains were solved analytically with the finite integral transform method. Finally, the continuity conditions were introduced to obtain the analytical solution of the original problem. Based on the side spot-welded cantilever plates commonly used in engineering, the free vibration problem of a rectangular thin plate with 1 edge subjected to clamped-simply supported constraints and the other 3 edges free, was analyzed. The obtained natural frequencies and mode shapes are in good agreement with those from the finite element method as well as the solutions in literature, thus verifying the accuracy of the proposed method. The solution procedure of the finite integral transform method can be implemented based on the governing equations without any assumption of the solution form. Therefore, this strict analytical method is widely applicable to complex boundary value problems of higher-order partial differential equations for such mechanical problems of plates and shells.
-
Key words:
- finite integral transform method /
- mixed boundary constraint /
- rectangular thin plate /
- free vibration /
- analytical solution
edited-byedited-by1) (我刊编委李锐来稿) -
图 1 CS-F-F-F型混合边界矩形薄板
Figure 1. The rectangular thin plate under CS-F-F-F mixed boundary constraints
图 2 CS-F-F-F型混合边界矩形薄板各子域示意图
Figure 2. Schematic diagram of the sub-domains of CS-F-F-F-F rectangular thin plates
表 1 CS-F-F-F方板前十阶无量纲固有频率$\omega b^2 \sqrt{\rho h / D} $收敛性研究
Table 1. Convergence study of the first 10 non-dimensional natural frequencies and $\omega b^2 \sqrt{\rho h / D} $ of CS-F-F-F square plates
M(N) mode 1st 2nd 3rd 4th 5th 6th 7th 8th 9th 10th 10 2.759 7.541 18.21 26.79 28.53 51.14 55.05 63.68 67.75 89.96 20 2.769 7.547 18.23 26.80 28.57 51.15 55.12 63.74 67.77 90.03 30 2.773 7.548 18.24 26.80 28.57 51.15 55.14 63.75 67.78 90.05 50 2.775 7.549 18.25 26.81 28.57 51.16 55.16 63.75 67.78 90.07 100 2.777 7.550 18.25 26.81 28.58 51.16 55.17 63.76 67.78 90.08 150 2.778 7.550 18.25 26.81 28.58 51.16 55.18 63.76 67.78 90.08 200 2.778 7.550 18.25 26.81 28.58 51.16 55.18 63.76 67.78 90.08 
下载: 导出CSV
表 2 不同长宽比CS-F-F-F板在b1=b2条件下的无量纲固有频率
Table 2. Non-dimensional natural frequencies of CS-F-F-F plates with different aspect ratios, with b1=b2
a/b method mode 1st 2nd 3rd 4th 5th 6th 7th 8th 9th 10th 0.5 present 8.906 18.63 38.19 68.19 74.32 93.39 116.9 134.0 162.9 204.4 FEM 8.915 18.63 38.19 68.20 74.32 93.40 116.9 134.0 162.9 204.4 XU et al. [4] 8.910 18.62 38.18 68.17 74.33 93.37 116.9 134.0 162.9 204.4 1 present 2.778 7.550 18.25 26.81 28.58 51.16 55.18 63.76 67.78 90.08 FEM 2.780 7.550 18.26 26.81 28.58 51.16 55.20 63.76 67.79 90.09 XU et al. [4] 2.778 7.552 18.25 26.81 28.58 51.16 55.18 63.77 67.80 90.10 1.5 present 1.320 4.710 8.531 16.20 23.39 25.29 33.63 37.35 49.03 57.74 FEM 1.321 4.710 8.534 16.20 23.39 25.29 33.63 37.35 49.04 57.75 XU et al. [4] 1.320 4.710 8.533 16.20 23.39 25.29 33.63 37.35 49.03 57.74 2 present 0.767 2 3.413 4.923 11.19 14.10 21.71 23.26 28.12 31.15 36.46 FEM 0.767 9 3.413 4.924 11.19 14.10 21.71 23.26 28.12 31.15 36.47 XU et al. [4] 0.767 2 3.412 4.923 11.19 14.10 21.71 23.25 28.11 31.15 36.46 2.5 present 0.500 4 2.659 3.224 8.344 9.362 15.89 18.13 22.94 25.61 28.03 FEM 0.501 3 2.660 3.224 8.345 9.363 15.89 18.13 22.95 25.61 28.03 XU et al. [4] 0.500 4 2.658 3.223 8.344 9.362 15.88 18.13 22.95 25.61 28.04 3 present 0.351 9 2.106 2.352 6.151 7.193 12.04 13.22 19.45 21.12 22.86 FEM 0.352 4 2.107 2.352 6.153 7.193 12.04 13.23 19.45 21.13 22.86 XU et al. [4] 0.351 9 2.106 2.352 6.150 7.194 12.04 13.23 19.45 21.12 22.86 3.5 present 0.260 8 1.613 1.933 4.590 5.984 9.073 10.69 15.01 16.45 21.95 FEM 0.261 3 1.614 1.934 4.591 5.984 9.075 10.69 15.01 16.45 21.96 XU et al. [4] 0.260 8 1.613 1.933 4.589 5.983 9.073 10.69 15.00 16.45 21.95 4 present 0.201 0 1.252 1.670 3.541 5.143 7.008 9.058 11.65 13.66 17.42 FEM 0.201 6 1.253 1.670 3.542 5.143 7.009 9.058 11.66 13.66 17.43 XU et al. [4] 0.200 9 1.252 1.669 3.540 5.143 7.006 9.060 11.66 13.66 17.43
下载: 导出CSV
-
[1] 田宇. 求解矩形薄板问题的辛-叠加方法[D]. 硕士学位论文. 大连: 大连理工大学, 2018.TIAN Yu. On the symplectic superposition method for rectangular thin plates[D]. Master Thesis. Dalian: Dalian University of Technology, 2018. (in Chinese) [2] 李若愚, 王天宏. 薄板热力耦合的屈曲分析[J]. 应用数学和力学, 2020, 41(8): 877-886. doi: 10.21656/1000-0887.400308LI Ruoyu, WANG Tianhong. Thermo-mechanical buckling analysis of thin plates[J]. Applied Mathematics and Mechanics, 2020, 41(8): 877-886. (in Chinese) doi: 10.21656/1000-0887.400308 [3] 张磊, 张文明, 王林, 等. 基于小波Galerkin法的矩形薄板二次屈曲分析[J]. 应用数学和力学, 2023, 44(1): 25-35. doi: 10.21656/1000-0887.430097ZHANG Lei, ZHANG Wenming, WANG Lin, et al. Secondary buckling analysis of thin rectangular plates based on the wavelet Galerkin method[J]. Applied Mathematics and Mechanics, 2023, 44(1): 25-35. (in Chinese) doi: 10.21656/1000-0887.430097 [4] XU D, NI Z F, LI Y H, et al. On the symplectic superposition method for free vibration of rectangular thin plates with mixed boundary constraints on an edge[J]. Theoretical and Applied Mechanics Letters, 2021, 11(5): 100293. doi: 10.1016/j.taml.2021.100293 [5] 王磊, 蔡松柏. 简支任意四边形板的弯曲、稳定和振动的Navier解法[J]. 湖南大学学报, 1989, 16(1): 104-114. https://www.cnki.com.cn/Article/CJFDTOTAL-HNDX198901017.htmWANG Lei, CAI Songbo. Navier's method for solving the problem of bending, buckling and vibration of all edges simply supported quadrilateral plates[J]. Journal of Hunan University, 1989, 16(1): 104-114. (in Chinese) https://www.cnki.com.cn/Article/CJFDTOTAL-HNDX198901017.htm [6] THAI H T, CHOI D H. Levy solution for free vibration analysis of functionally graded plates based on a refined plate theory[J]. KSCE Journal of Civil Engineering, 2014, 18(6): 1813-1824. doi: 10.1007/s12205-014-0409-2 [7] 丁鹏飞. 超高强度汽车钢板电阻点焊结构强度的优化分析[D]. 硕士学位论文. 上海: 华东理工大学, 2016: 1-26.DING Pengfei. Optimization on structure strength of resistance spot welding of ultra high strength steel[D]. Master Thesis. Shanghai: East China University of Science and Technology, 2016: 1-26. (in Chinese) [8] ZIENKIEWICZ O C, CHEUNG Y K. The finite element method for analysis of elastic isotropic and orthotropic slabs[J]. ICE Proceedings, 1964, 28(4): 471-488. [9] BUCZKOWSKI R, TORBACKI W. Finite element modelling of thick plates on two-parameter elastic foundation[J]. International Journal for Numerical and Analytical Methods in Geomechanics, 2001, 25(14): 1409-1427. doi: 10.1002/nag.187 [10] CHEUNG B Y K. Finite Strip Method in Structural Analysis[M]. London: Pergamon Press, 1976. [11] HENWOOD D J, WHITEMAN J R, YETTRAM A L. Finite difference solution of a system of first-order partial differential equations[J]. International Journal for Numerical Methods in Engineering, 2010, 17(9): 1385-1395. [12] CHAKRAVORTY A K, GHOSH A. Finite difference solution for circular plates on elastic foundations[J]. International Journal for Numerical Methods in Engineering, 2010, 9(1): 73-84. [13] BREBBIA C A. The Boundary Element Method for Engineers[M]. New York: Halsted Press, 1978. [14] PEREIRA WL A, KARAM V J, CARRER J A M, et al. A dynamic formulation for the analysis of thick elastic plates by the boundary element method[J]. Engineering Analysis With Boundary Elements, 2012, 36(7): 1138-1150. doi: 10.1016/j.enganabound.2012.02.002 [15] LIEW K M, HAN J B. Bending solution for thick plates with quadrangular boundary[J]. Journal of Engineering Mechanics, 1998, 124(1): 9-17. doi: 10.1061/(ASCE)0733-9399(1998)124:1(9) [16] LIU F L, LIEW K M. Differential cubature method for static solutions of arbitrarily shaped thick plates[J]. International Journal of Solids Structures, 1998, 35(28/29): 3655-3674. [17] ZHONG Y, SUN A M, ZHOU F L, et al. Analytical solution for rectangular thin plate on elastic foundation with four edges free by finite cosine integral transform method[J]. Chinese Journal of Geotechnical Engineering, 2006, 28(11): 2019-2022. [18] ZHONG Y, WANG G X, SUN A M. Vibration of a thin plate on Winkler foundation with completely free boundary by finite cosine integral transform method[J]. Journal of Dalian University of Technology, 2007, 47(1): 73-77. [19] ZHONG Y, YIN J H. Free vibration analysis of a plate on foundation with completely free boundary by finite integral transform method[J]. Mechanics Research Communications, 2008, 35(4): 268-275. doi: 10.1016/j.mechrescom.2008.01.004 [20] LI R, ZHONG Y, TIAN B, et al. On the finite integral transform method for exact bending solutions of fully clamped orthotropic rectangular thin plates[J]. Applied Mathematics Letters, 2009, 22(12): 1821-1827. [21] ZHONG Y, ZHAO X F, LI R. Freevibration analysis of rectangular cantilever plates by finite integral transform method[J]. International Journal for Computational Methods in Engineering Science and Mechanics, 2013, 14(3): 221-226. [22] AN D Q, XU D, NI Z F, et al. Finite integral transform method for analytical solutions of static problems of cylindrical shell panels[J]. European Journal of Mechanics A: Solids, 2020, 83: 104033. [23] CHEN Y M, AN D Q, ZHOU C, et al. Analytical free vibration solutions of rectangular edge-cracked plates by the finite integral transform method[J]. International Journal of Mechanical Sciences, 2023, 243: 108032. [24] KHALILI M R, MALEKZADEH K, MITTAL R K. A new approach to static and dynamic analysis of composite plates with different boundary conditions[J]. Composite Structures, 2005, 69(2): 149-155. -
图(3) / 表(2)
计量
- 文章访问数: 536
- HTML全文浏览量: 197
- PDF下载量: 67
- 被引次数: 0
渝公网安备50010802005915号