一类Caputo-Fabrizio型分数阶微分方程的三次B样条方法

胡行华; 蔡俊迎

doi:10.21656/1000-0887.430195

一类Caputo-Fabrizio型分数阶微分方程的三次B样条方法

doi: 10.21656/1000-0887.430195

胡行华,
蔡俊迎^,

辽宁工程技术大学理学院，辽宁阜新 123000

基金项目:

辽宁省自然科学基金项目 2020-MS-301

教育部人文社会科学研究项目 2021YJCZH204

辽宁省教育厅高等学校基本科研项目 LJ2019JL005

辽宁省教育厅高等学校基本科研项目 LJ2020ZD002

辽宁省教育厅高等学校基本科研项目 2022lslwtkt-069

详细信息

作者简介:
胡行华(1978—)，男，副教授，硕士生导师(E-mail: 121681692@qq.com)

通讯作者:
蔡俊迎(1998—)，女，硕士生(通讯作者. E-mail: caijy0302@163.com)

中图分类号: O241.8
计量
- 文章访问数: 469
- HTML全文浏览量: 174
- PDF下载量: 42
- 被引次数: 0
出版历程
- 收稿日期: 2022-06-07
- 修回日期: 2022-08-24
- 刊出日期: 2023-06-01

The Cubic B-Spline Method for a Class of Caputo-Fabrizio Fractional Differential Equations

HU Xinghua,
CAI Junying^,

School of Sciences, Liaoning Technical University, Fuxin, Liaoning 123000, P.R.China

摘要

摘要: 基于分数阶微积分基本定理和三次B样条理论，构造了求解线性Caputo-Fabrizio型分数阶微分方程数值解的三次B样条方法，利用分数阶微积分基本定理将初值问题转化为关于解函数的表达式，再使用三次B样条函数逼近表达式中积分项的被积函数，进而计算了一类Caputo-Fabrizio型分数阶微分方程的数值解. 给出了所构造的三次B样条方法的误差估计、收敛性和稳定性的理论证明. 数值实验表明，该文数值方法在求解一类Caputo-Fabrizio型分数阶微分方程数值解时具有一定的可行性和有效性，且计算精度和计算效率优于现有的两种数值方法.
- Caputo-Fabrizio分数阶导数 /
- 三次B样条方法 /
- 误差估计 /
- 收敛性 /
- 稳定性
Abstract: Based on the basic theorem of fractional calculus and the cubic B-spline theory, the cubic B-spline method for numerical solution of linear Caputo-Fabrizio fractional differential equations was proposed. The basic theorem of fractional calculus was used to transform the initial value problem into an expression about the solution function, and the cubic B-spline function was used to approximate the integrand function in the expression. Then the numerical solutions of the Caputo-Fabrizio fractional differential equations were calculated. The error estimation, convergence and stability of the constructed cubic B-spline method were given theoretically. Numerical experiments show that, the presented numerical method is feasible and effective in solving a class of Caputo-Fabrizio fractional differential equations, and the computation accuracy and efficiency are better than the 2 existing numerical methods.
- Caputo-Fabrizio fractional derivative /
- cubic B-spline method /
- error estimation /
- convergence /
- stability

HTML全文

图 1 当α=0.3时，3种数值方法的最大误差(情况1)

Figure 1. Maximum errors of the 3 numerical methods for α=0.3 (case 1)

下载: 全尺寸图片幻灯片

图 2 当α=0.7时，3种数值方法的最大误差(情况1)

Figure 2. Maximum errors of the 3 numerical methods for α=0.7 (case 1)

下载: 全尺寸图片幻灯片

图 3 不同α值时各节点的绝对误差(情况1)

Figure 3. Absolute errors of each node with different α values (case 1)

下载: 全尺寸图片幻灯片

图 4 不同α值时各节点的数值解(情况1)

Figure 4. Numerical solutions of each node with different α values (case 1)

下载: 全尺寸图片幻灯片

图 5 当α=0.3时，3种数值方法的最大误差(情况2)

Figure 5. Maximum errors of the 3 numerical methods for α=0.3 (case 2)

下载: 全尺寸图片幻灯片

图 6 当α=0.7时，3种数值方法的最大误差(情况2)

Figure 6. Maximum errors of the 3 numerical methods for α=0.7 (case 2)

下载: 全尺寸图片幻灯片

图 7 不同α值时各节点的绝对误差(情况2)

Figure 7. Absolute errors of each node with different α values (case 2)

下载: 全尺寸图片幻灯片

图 8 不同α值时各节点的数值解(情况2)

Figure 8. Numerical solutions of each node with different α values (case 2)

下载: 全尺寸图片幻灯片

图 9 当α=0.3时，3种数值方法的相对误差

Figure 9. Relative errors of 3 numerical methods for α=0.3

下载: 全尺寸图片幻灯片

图 10 当α=0.5时，3种数值方法的相对误差

Figure 10. Relative errors of 3 numerical methods for α=0.5

下载: 全尺寸图片幻灯片

图 11 当α=0.7时，3种数值方法的相对误差

Figure 11. Relative errors of 3 numerical methods for α=0.7

下载: 全尺寸图片幻灯片

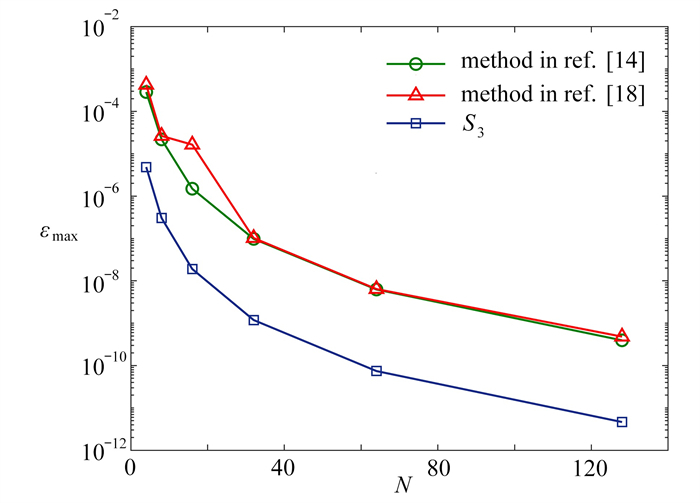
表 1 当α=0.3时，3种数值方法的最大误差和收敛阶(情况1)

Table 1. Maximum errors and convergence orders of 3 numerical methods for α=0.3 (case 1)

N	method in ref. [14]	rate r	CPU time T/s	method in ref. [18]	rate r	CPU time T/s	S₃	rate r	CPU time T/s
4	2.863 3E-4	-	0.000 6	4.244 2E-4	-	0.000 3	4.861 4E-6	-	0.000 1
8	2.181 2E-5	3.714 5	0.001 5	2.634 4E-5	4.001 0	0.001 1	3.039 0E-7	3.999 7	0.000 2
16	1.495 5E-6	3.866 4	0.004 3	1.640 7E-6	4.005 1	0.001 2	1.899 5E-8	3.999 9	0.000 2
32	9.776 7E-8	3.935 1	0.010 7	1.023 6E-7	4.002 6	0.015 7	1.187 2E-9	4.000 0	0.000 4
64	6.247 4E-9	3.968 0	0.046 2	6.391 9E-9	4.001 3	0.033 1	7.420 7E-11	3.999 9	0.002 8
128	3.947 8E-10	3.984 1	0.292 1	4.821 1E-10	3.728 8	0.185 1	4.645 2E-12	3.997 8	0.005 6

下载: 导出CSV

表 2 当α=0.7时，3种数值方法的最大误差和收敛阶(情况1)

Table 2. Maximum errors and convergence orders of 3 numerical methods for α=0.7 (case 1)

N	method in ref. [14]	rate r	CPU time T/s	method in ref. [18]	rate r	CPU time T/s	S₃	rate r	CPU time T/s
4	1.498 5E-3	-	0.000 5	2.440 9E-3	-	0.000 3	6.803 8E-5	-	0.000 2
8	1.131 7E-4	3.727 0	0.002 7	1.477 4E-4	4.046 3	0.001 1	4.278 2E-6	3.991 3	0.000 2
16	7.205 3E-6	3.973 3	0.004 1	9.070 8E-6	4.025 7	0.001 5	2.677 9E-7	3.997 8	0.000 3
32	4.431 2E-7	4.023 3	0.021 2	5.616 7E-7	4.013 4	0.016 8	1.674 3E-8	3.999 5	0.000 3
64	3.077 3E-8	3.848 0	0.046 9	3.493 8E-8	4.006 9	0.040 4	1.046 6E-9	3.999 9	0.002 6
128	2.046 0E-9	3.910 8	0.303 5	2.178 4E-9	4.003 5	0.212 4	6.541 1E-11	4.000 0	0.005 5

下载: 导出CSV

表 3 当α=0.3时，3种数值方法的最大误差和收敛阶(情况2)

Table 3. Maximum errors and convergence orders of 3 numerical methods for α=0.3 (case 2)

N	method in ref. [14]	rate r	CPU time T/s	method in ref. [18]	rate r	CPU time T/s	S₃	rate r	CPU time T/s
4	1.044 5E-3	-	0.000 8	1.882 9E-3	-	0.000 2	2.909 0E-5	-	0.000 2
8	7.592 6E-5	3.782 0	0.003 5	1.104 9E-4	4.090 9	0.001 2	1.806 0E-6	4.009 7	0.000 3
16	5.354 7E-6	3.825 7	0.004 6	6.649 0E-6	4.054 7	0.001 9	1.127 0E-7	4.002 3	0.000 3
32	3.633 3E-7	3.881 5	0.021 3	4.070 3E-7	4.030 0	0.014 8	7.040 6E-9	4.000 6	0.000 6
64	2.381 4E-8	3.931 4	0.047 4	2.512 5E-8	4.017 9	0.038 2	4.400 3E-10	4.000 0	0.003 8
128	1.319 4E-9	4.173 9	0.300 7	1.338 0E-9	4.231 0	0.269 6	2.753 6E-11	3.998 2	0.007 7

下载: 导出CSV

表 4 当α=0.7时，3种数值方法的最大误差和收敛阶(情况2)

Table 4. Maximum errors and convergence orders of 3 numerical methods for α=0.7 (case 2)

N	method in ref. [14]	rate r	CPU time T/s	method in ref. [18]	rate r	CPU time T/s	S₃	rate r	CPU time T/s
4	2.550 6E-3	-	0.000 8	4.181 8E-3	-	0.000 3	2.068 7E-4	-	0.000 2
8	2.102 5E-4	3.600 7	0.003 2	2.302 9E-4	4.182 6	0.001 3	1.256 8E-5	4.041 0	0.000 2
16	1.295 5E-5	4.020 5	0.004 7	1.352 3E-5	4.090 0	0.001 7	7.797 0E-7	4.010 6	0.000 4
32	7.477 9E-7	4.114 7	0.025 8	8.194 8E-7	4.044 6	0.015 6	4.863 9E-8	4.002 7	0.000 6
64	4.419 4E-8	4.080 7	0.050 2	5.043 7E-8	4.022 2	0.035 4	3.038 5E-9	4.000 7	0.004 1
128	2.930 5E-9	3.914 6	0.333 5	3.128 2E-9	4.011 1	0.284 8	1.898 9E-10	4.000 2	0.007 3

下载: 导出CSV

表 5 α=0.3, N=10 000时，3种数值方法在不同节点处的绝对误差和相对误差

Table 5. The absolute and relative errors of the 3 numerical methods at different nodes for α=0.3, N=10 000

t_i	method in ref. [14] (CPU time T=25.11 s)		method in ref. [18] (CPU time T=23.05 s)		S₃ (CPU time T=10.91 s)		exact solution
t_i	absolute error ε	relative error ε'	absolute error ε	relative error ε'	absolute error ε	relative error ε'	exact solution
100	1.179 7E-6	-2.329 6E-6	1.105 8E-6	-2.183 6E-6	5.867 9E-9	-1.158 8E-8	-0.506 4
200	1.418 9E-6	-1.624 8E-6	1.594 7E-6	-1.826 1E-6	5.127 6E-9	-5.871 5E-9	-0.873 3
300	1.591 7E-6	-1.592 1E-6	1.658 1E-6	-1.658 4E-6	2.809 5E-8	-2.810 0E-8	-0.999 8
400	1.650 6E-6	-1.939 8E-6	1.278 6E-6	-1.502 6E-6	5.670 9E-8	-6.664 6E-8	-0.850 9
500	1.579 2E-6	-3.375 8E-6	5.608 3E-7	-1.198 9E-6	8.309 1E-8	-1.776 2E-7	-0.467 8
600	1.397 3E-6	3.161 3E-5	2.977 0E-7	6.735 3E-6	9.997 6E-8	2.261 9E-6	0.044 2
700	1.154 9E-6	2.123 0E-6	1.060 5E-6	1.949 4E-6	1.027 2E-7	1.888 2E-7	0.544 0
800	9.188 7E-7	1.027 8E-6	1.517 6E-6	1.697 5E-6	9.055 4E-8	1.012 9E-7	0.894 0
900	7.540 9E-7	7.557 5E-7	1.543 1E-6	1.546 5E-6	6.684 1E-8	6.698 8E-8	0.997 8
1 000	7.059 8E-7	8.537 7E-7	1.129 9E-6	1.366 4E-6	3.814 1E-8	4.612 5E-8	0.826 9

下载: 导出CSV

表 6 α=0.5, N=10 000时，3种数值方法在不同节点处的绝对误差和相对误差

Table 6. The absolute and relative errors of the 3 numerical methods at different nodes for α=0.5, N=10 000

t_i	method in ref. [14] (CPU time T=25.21 s)		method in ref. [18] (CPU time T=21.79 s)		S₃ (CPU time T=10.72 s)		exact solution
t_i	absolute error ε	relative error ε'	absolute error ε	relative error ε'	absolute error ε	relative error ε'	exact solution
100	3.560 3E-6	-7.030 6E-6	-7.030 6E-6	-4.480 6E-6	9.535 4E-8	-1.883 0E-7	-0.506 4
200	4.582 3E-6	-5.247 2E-6	-5.247 2E-6	-3.733 8E-6	9.478 3E-8	-1.085 3E-7	-0.873 3
300	5.143 2E-6	-5.144 3E-6	-5.144 3E-6	-3.374 4E-6	6.810 3E-8	-6.811 6E-8	-0.999 8
400	5.088 5E-6	-5.980 1E-6	-5.980 1E-6	-3.028 3E-6	2.266 0E-8	-2.663 1E-8	-0.850 9
500	4.433 2E-6	-9.476 7E-6	-9.476 7E-6	-2.329 0E-6	2.903 1E-8	-6.205 8E-8	-0.467 8
600	3.357 8E-6	7.596 9E-5	7.596 9E-5	1.535 6E-5	7.273 7E-8	1.645 6E-6	0.044 2
700	2.158 5E-6	3.967 8E-6	3.967 8E-6	4.119 3E-6	9.642 3E-8	1.772 5E-7	0.544 0
800	1.165 4E-6	1.303 6E-6	1.303 6E-6	3.542 4E-6	9.356 7E-8	1.046 6E-7	0.894 0
900	6.520 4E-7	6.534 7E-7	6.534 7E-7	3.208 8E-6	6.495 6E-8	6.509 9E-8	0.997 8
1 000	7.597 7E-7	9.188 2E-7	9.188 2E-7	2.824 8E-6	1.840 6E-8	2.2258E-8	0.826 9

下载: 导出CSV

表 7 α=0.7, N=10 000时，3种数值方法在不同节点处的绝对误差和相对误差

Table 7. The absolute and relative errors of the 3 numerical methods at different nodes for α=0.7, N=10 000

t_i	method in ref. [14] (CPU time T=26.02 s)		method in ref. [18] (CPU time T=22.70 s)		S₃ (CPU time T=10.21 s)		exact solution
t_i	absolute error ε	relative error ε'	absolute error ε	relative error ε'	absolute error ε	relative error ε'	exact solution
100	1.272 2E-5	-2.512 2E-5	3.742 0E-6	-7.389 4E-6	1.541 5E-6	-3.044 1E-6	-0.506 4
200	1.759 9E-5	-2.015 2E-5	5.318 1E-6	-6.089 7E-6	1.565 8E-6	-1.793 0E-6	-0.873 3
300	1.973 9E-5	-1.974 3E-5	5.409 3E-6	-5.410 4E-6	1.555 0E-6	-1.555 3E-6	-0.999 8
400	1.855 2E-5	-2.180 3E-5	3.990 5E-6	-4.689 7E-6	1.512 1E-6	-1.777 0E-6	-0.850 9
500	1.436 5E-5	-3.070 8E-5	1.452 4E-6	-3.104 7E-6	1.448 9E-6	-3.097 2E-6	-0.467 8
600	8.331 4E-6	1.884 9E-4	1.506 2E-6	3.407 7E-5	1.382 8E-6	3.128 4E-5	0.044 2
700	2.112 3E-6	3.882 8E-6	4.070 5E-6	7.482 5E-6	1.332 0E-6	2.448 5E-6	0.544 0
800	2.579 9E-6	2.885 8E-6	5.534 5E-6	6.190 7E-6	1.310 5E-6	1.465 9E-6	0.894 0
900	4.453 1E-6	4.462 9E-6	5.495 0E-6	5.507 1E-6	1.324 2E-6	1.327 2E-6	0.997 8
1 000	2.991 4E-6	3.617 6E-6	3.962 9E-6	4.792 5E-6	1.369 6E-6	1.656 3E-6	0.826 9

下载: 导出CSV

参考文献(25)

[1]	张嫚, 曹艳华, 杨晓忠. 一类分数阶Langevin方程block-by-block算法的数值分析[J]. 应用数学和力学, 2021, 42(6): 562-574. doi: 10.21656/1000-0887.410337 ZHANG Man, CAO Yanhua, YANG Xiaozhong. Numerical analysis of a class of fractional Langevin equations with the block-by-block method[J]. Applied Mathematics and Mechanics, 2021, 42(6): 562-574. (in Chinese) doi: 10.21656/1000-0887.410337
[2]	鲍四元, 沈峰. 分数阶常微分方程的改进精细积分法[J]. 应用数学和力学, 2019, 40(12): 1309-1320. doi: 10.21656/1000-0887.390355 BAO Siyuan, SHEN Feng. An improved precise integration method for fractional ordinary differential equations[J]. Applied Mathematics and Mechanics, 2019, 40(12): 1309-1320. (in Chinese) doi: 10.21656/1000-0887.390355
[3]	CAPUTO M, FABRIZIO M. A new definition of fractional derivative without singular kernel[J]. Progress in Fractional Differentiation and Applications, 2015, 1: 73-85.
[4]	CAPUTO M, FABRIZIO M. Applications of new time and spatial fractional derivatives with exponential kernels[J]. Progress in Fractional Differentiation and Applications, 2016, 2(1): 1-11. doi: 10.18576/pfda/020101
[5]	LOSADA J, NIETO J J. Properties of a new fractional derivative without singular kernel[J]. Progress in Fractional Differentiation and Applications, 2015, 1(2): 87-92.
[6]	OWOLABI K M, ATANGANA A. Analysis and application of new fractional Adams-Bashforth scheme with Caputo-Fabrizio derivative[J]. Chaos, Solitons and Fractals, 2017, 105: 111-119. doi: 10.1016/j.chaos.2017.10.020
[7]	ATANGANA A, ALQAHTANI R T. Numerical approximation of the space-time Caputo-Fabrizio fractional derivative and application to groundwater pollution equation[J]. Advances in Difference Equations, 2016, 2016(1): 156. doi: 10.1186/s13662-016-0871-x
[8]	BALEANU D, JAJARMI A, MOHAMMADI H, et al. A new study on the mathematical modelling of human liver with Caputo-Fabrizio fractional derivative[J]. Chaos, Solitons and Fractals, 2020, 134: 109705. doi: 10.1016/j.chaos.2020.109705
[9]	BOUDAOUI A, MOUSSA Y, HAMMOUCH Z, et al. A fractional-order model describing the dynamics of the novel coronavirus (COVID-19) with nonsingular kernel[J]. Chaos, Solitons and Fractals, 2021, 146: 110859. doi: 10.1016/j.chaos.2021.110859
[10]	GONG X, FATMAWATI, KHAN M A. A new numerical solution of the competition model among bank data in Caputo-Fabrizio derivative[J]. Alexandria Engineering Journal, 2020, 59(4): 2251-2259. doi: 10.1016/j.aej.2020.02.008
[11]	LIAO X, LIN D, DONG L, et al. Analog implementation of fractional-order electric elements using Caputo-Fabrizio and Atangana-Baleanu definitions[J]. Fractals, 2021, 29(7): 2150235. doi: 10.1142/S0218348X21502352
[12]	ARIF M, ALI F, SHEIKH N A, et al. Fractional model of couple stress fluid for generalized Couette flow: a comparative analysis of Atangana-Baleanu and Caputo-Fabrizio fractional derivatives[J]. IEEE Access, 2019, 7: 88643-88655. doi: 10.1109/ACCESS.2019.2925699
[13]	YOUSSEF H M, EL-BARY A A. Generalized fractional viscothermoelastic nanobeam under the classical Caputo and the new Caputo-Fabrizio definitions of fractional derivatives[J]. Waves in Random and Complex Media, 2023, 33(3): 545-566. doi: 10.1080/17455030.2021.1883767
[14]	CAO J, WANG Z, XU C. A high-order scheme for fractional ordinary differential equations with the Caputo-Fabrizio derivative[J]. Communications on Applied Mathematics and Computation, 2020, 2(2): 179-199. doi: 10.1007/s42967-019-00043-8
[15]	GUO X, LI Y T, ZENG T Y. A finite difference scheme for Caputo-Fabrizio fractional differential equations[J]. International Journal of Numerical Analysis and Modeling, 2020, 17(2): 195-211.
[16]	AL-SMADI M, DJEDDI N, MOMANI S, et al. An attractive numerical algorithm for solving nonlinear Caputo-Fabrizio fractional Abel differential equation in a Hilbert space[J]. Advances in Difference Equations, 2021, 271: 2-18.
[17]	DOUAIFIA R, BENDOUKHA S, ABDELMALEK S. A Newton interpolation based predictor-corrector numerical method for fractional differential equations with an activator-inhibitor case study[J]. Mathematics and Computers in Simulation, 2021, 187: 391-413.
[18]	何广婷. 带有Caputo-Fabrizio导数的分数阶微分方程的快速高阶算法的研究[D]. 硕士学位论文. 哈尔滨: 哈尔滨师范大学, 2021. HE Guangting. Fast higher order algorithms research for fractional differential equations with Caputo-Fabrizio derivatives[D]. Master Thesis. Harbin: Harbin Normal University, 2021. (in Chinese)
[19]	李鹏柱, 李风军, 李星, 等. 基于三次样条插值函数的非线性动力系统数值求解[J]. 应用数学和力学, 2015, 36(8): 887-896. doi: 10.3879/j.issn.1000-0887.2015.08.010 LI Pengzhu, LI Fengjun, LI Xing, et al. A numerical method for the solutions to nonlinear dynamic systems based on cubic spline interpolation functions[J]. Applied Mathematics and Mechanics, 2015, 36(8): 887-896. (in Chinese) doi: 10.3879/j.issn.1000-0887.2015.08.010
[20]	PODLUBNY I. Fractional Differential Equations[M]. New York: Academic Press, 1999.
[21]	RAMEZANI M, MOKHTARI R, HAASE G. Some high order formulae for approximating Caputo fractional derivatives[J]. Applied Numerical Mathematics, 2020, 153: 300-318.
[22]	李庆扬, 关治, 白峰杉. 数值计算原理[M]. 北京: 清华大学出版社, 2000. LI Qingyang, GUAN Zhi, BAI Fengshan. Theory of Numerical Calculation[M]. Beijing: Tsinghua University Press, 2000. (in Chinese)
[23]	黎天送. 基于粒子群的全局双三次B样条曲面插值方法及实验研究[D]. 硕士学位论文. 昆明: 云南大学, 2014. LI Tiansong. Global bicubic B-spline surface interpolation method based on particle swarm optimization and experimental research[D]. Master Thesis. Kunming: Yunnan University, 2014. (in Chinese)
[24]	KINCAID D R, CHENEY W. Numerical Analysis: Mathematics of Scientific Computing[M]. Pacific Grove, CA, USA: Cole Publishing Company, 2002.
[25]	HALL C A, MEYER W W. Optimal error bounds for cubic spline interpolation[J]. Journal of Approximation Theory, 1976, 16(2): 105-122.